10
$\begingroup$

Historical note (skip if you like):

In this interesting puzzle @TSLF asks for the "maximum number of black and white pieces that are involved in a checkmate position".

Originally, they left much open to interpretation, and interpret me and a handful of others did!

My interpretation did not exactly win, but I actually quite like it, so I decided to make a separate question of it.

Challenge:

Construct a legal position where white to move mates in one, subject to:

  1. The mate is unique. Update: This riddle smith is not above the law as layed down by the World Federation for Chess Composition in their Handbook of Chess Composition, article 13(2) with footnote 16 (thanks @trolley813) where it says: "[...] except that in the final move a promotion into different pieces having partially the same power (for example queen/rook or queen/bishop) may be tolerated.
  2. Removing any piece except a king results in a legal position (white to move, i.e. white king may be in check, black king mustn't).

An "accessory to regicide" is any piece which when removed leaves a position where white can no longer mate in one.

Your task: Maximise the number of accessories.

Bonus:

A "witness" is a non-king piece that is not an accessory. For those not well versed in the fine art of regicide: A perfect regicide is one where there are no witnesses. Let us define the regicide score as number of accessories - 3 x number of witnesses

Bonus task: Maximise the regicide score.

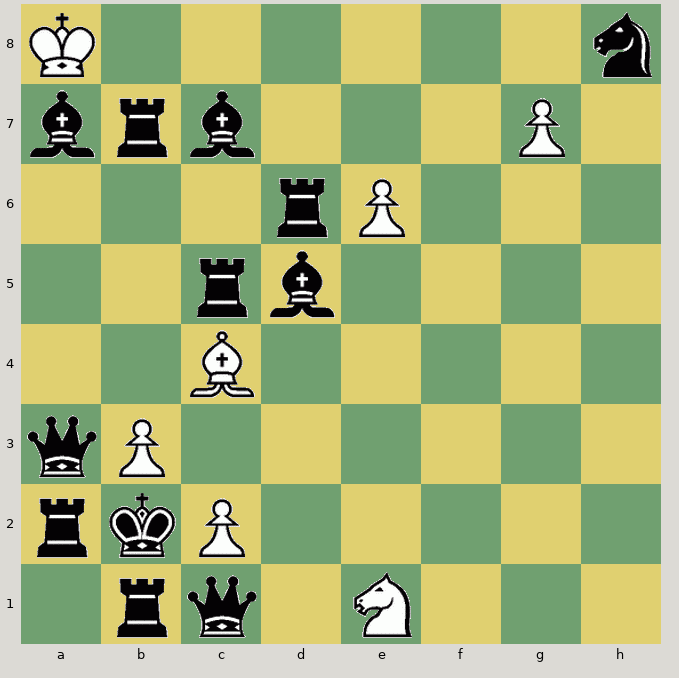
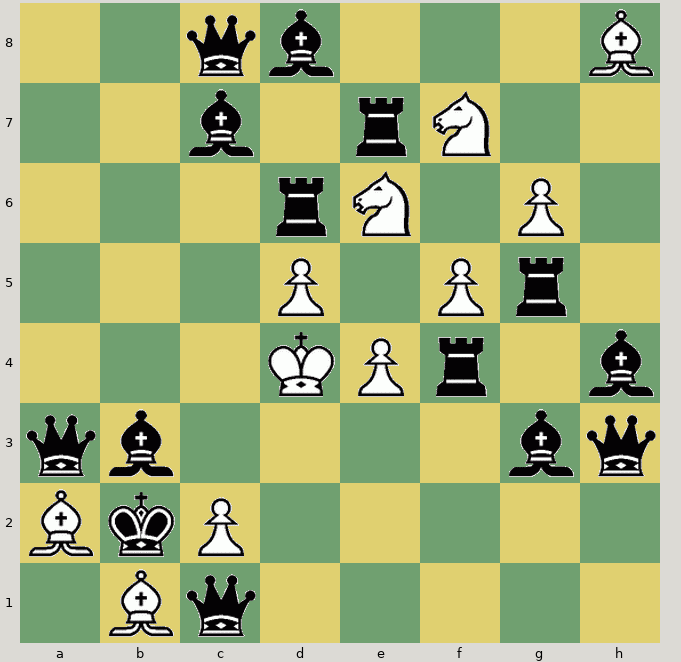
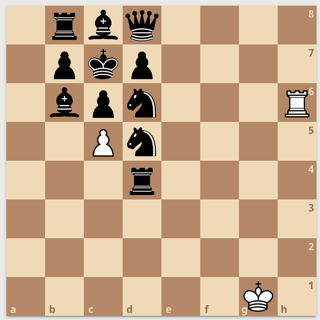
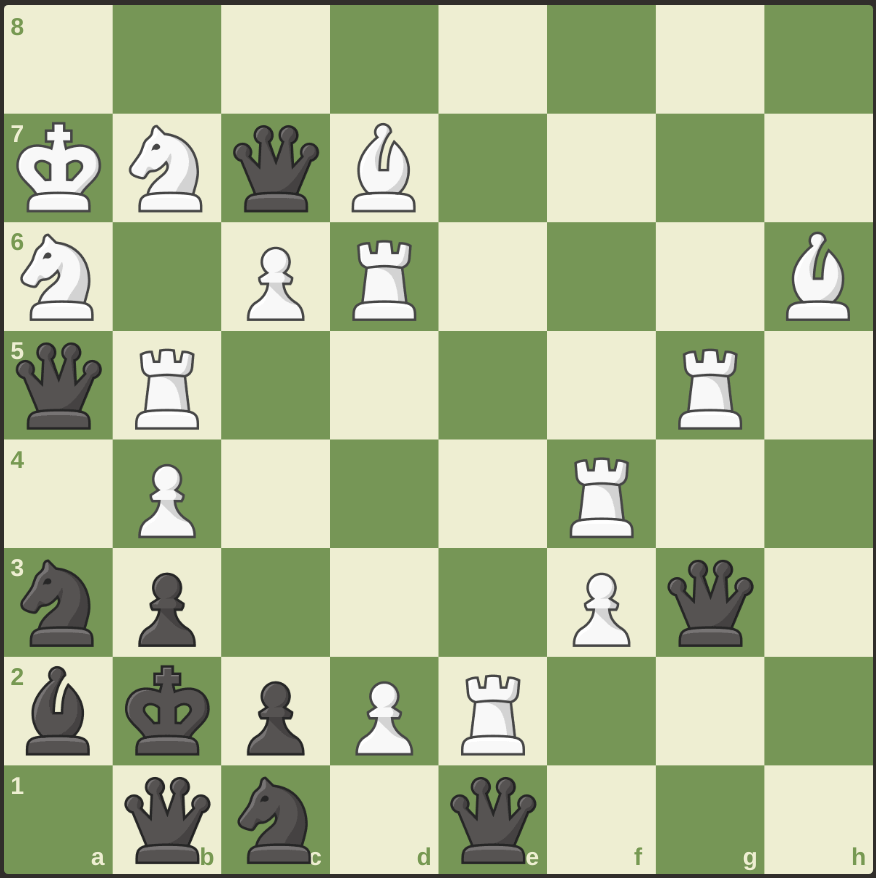
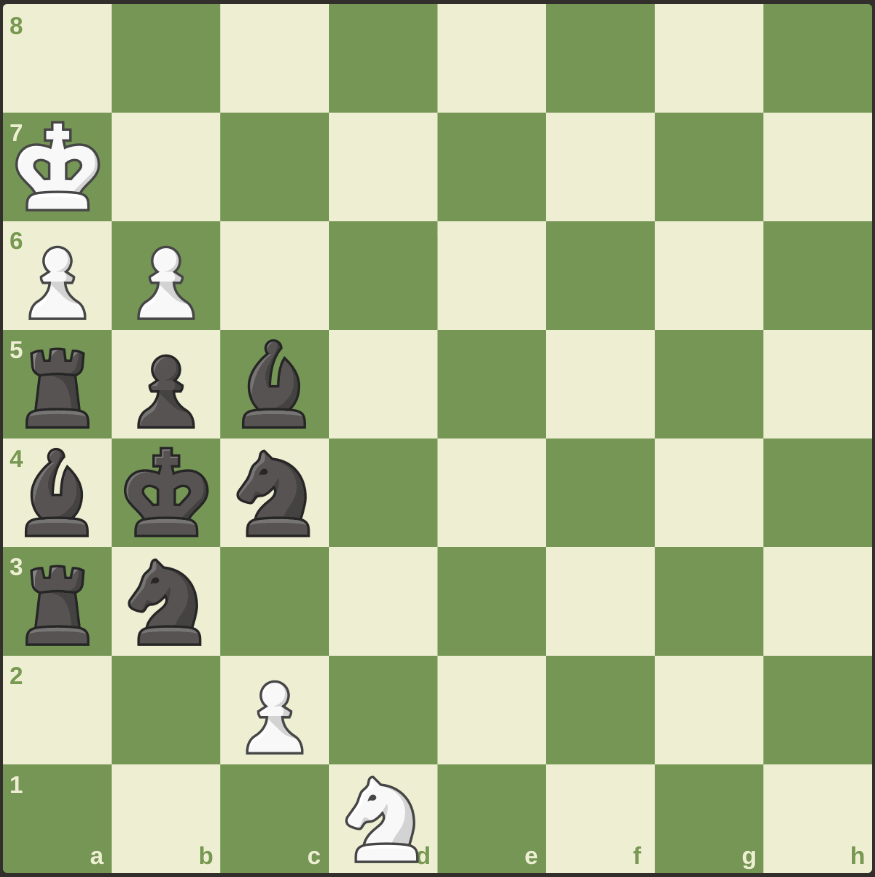
Perfectly valid example per article 13(2) and footnote 16 of the Handbook of Chess Comoposition:Faulty example (thanks @Magma who points out that the Nc8 cannot be removed without creating an illegal position.)

Note board is rotated. White pawns move down.
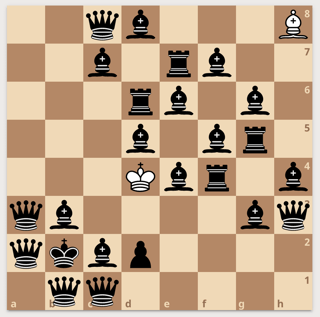
![enter image description here]
20 accessories (The kings and the black queens except for the one directly adjacent to her king do not count.) Four witnesses. Score: 8. This is not a valid example because there are technically two mates: gxh8B# and gxh8Q#

Improve this question
$\endgroup$
7
  • 1
    $\begingroup$ Does the position have to be theoretically reachable from the starting position? If not, I think I can improve on Albert's discovery. $\endgroup$
    – Bass
    Commented Mar 10, 2021 at 7:43
  • 1
    $\begingroup$ There's now an illegal regicide (now that's a term a lawyer would rarely need) at the bottom of my answer. $\endgroup$
    – Bass
    Commented Mar 10, 2021 at 8:28
  • 1
    $\begingroup$ Note that in "regular" chess composition (at least according to World Federation for Chess Composition's Handbook of Chess Composition), situations when the last move can be a promotion into different pieces sharing the same power (Q/R or Q/B) are usually tolerated (see Article 13 (2), footnote 16). $\endgroup$
    – trolley813
    Commented Mar 10, 2021 at 10:43
  • 2
    $\begingroup$ Wow, no shortage of arcane knowledge in this community! @trolley813 $\endgroup$
    – loopy walt
    Commented Mar 10, 2021 at 10:48
  • 2
    $\begingroup$ how is the position without the knight on c8 legal? $\endgroup$
    – Magma
    Commented Mar 11, 2021 at 23:44

3 Answers 3

Reset to default
10
$\begingroup$

Update: Committing the perfect crime is so much easier when one has the fine lawyers of the World Federation for Chess Composition on one's side:

Regicide score = number of accessories (no witnesses):

17
enter image description here
the unique (!) mate is gxh8Q / gxh8B.

End of update.

Exploiting the fact that kings are exempt from the removal test

we can construct a discovered checkmate

with

21

accessories and

2

witnesses for a regicide score of

15.


enter image description here
unique checkmate is 1. Kd3#

Some more explanation for casual players (pros will want to skip this):

The checkmate is delivered by the bishop in the far corner (h8), let's call him the assassin. It comes as a discovered check, i.e. in the diagram the assassin's path is blocked by the white king on d4 and only once the king has stepped aside to d3 the path to the black king is opened up.

Just as the white king can block the assassin's path, so could most other pieces. So let's skip forward and assume the white king as moved out of the way. The two witnesses, the queens on c8 and h3, could both save their king by moving to block the assassins path on c3, if they weren't themselves blocked by the bishops on c7 and g3. Which makes these two bishops complicit in their sovereign's demise.

What's more, either of those bishops could atone for their treachery by body blocking the assassin on e5 if they weren't blocked by the rooks on d6 and f4. These rooks in turn also could block the diagonal etc. We see that everybody in the north east of the board have blood on their hands except for the two witnesses. The sole reason for all the chaining (and branching) of A is blocked by B is blocked by C etc. is to achieve a high assassin to witness ratio.

The queens on a3 and c1 are similar to the witnesses in that they could block the assassin on c3 if they had clear sight of c3, but they also take away two escape squares for their king, making them accomplices.

Improve this answer
$\endgroup$
9
  • 2
    $\begingroup$ Love the Q-B-R cascades! $\endgroup$
    – loopy walt
    Commented Mar 9, 2021 at 12:37
  • 3
    $\begingroup$ Right, cool tricks to chain the black pieces to reduce the number of witnesses! And I like the storytelling in the explanation, haha $\endgroup$
    – justhalf
    Commented Mar 9, 2021 at 19:06
  • 2
    $\begingroup$ oeps, missed that $\endgroup$
    – Retudin
    Commented Mar 10, 2021 at 16:58
  • 2
    $\begingroup$ Nice, the chain just got longer! a2-a7-c5-d5-b7-c7-d6-e6! $\endgroup$
    – justhalf
    Commented Mar 11, 2021 at 3:49
  • 2
    $\begingroup$ The no witnesses solution is even prettier than the original one! $\endgroup$
    – loopy walt
    Commented Mar 13, 2021 at 5:54
6
$\begingroup$

The most sinister coup crowd I found has all the black non-pawn pieces conspiring against their own king, for a total of

12

regicide points. There are many ways to construct such a position, this seemed the most interesting:

enter image description here

EDIT: The question asks for a legal position, but in the comments OP indicated that an illegal position might be of interest too. Since I couldn't make a legal position with this idea, I decided to go completely overboard with the illegality, which allowed me to cram an extra piece into @Albert.Lang's brilliant discovery:

enter image description here

The basic idea is, of course, that if black could somehow have just one more non-knight piece, then replacing the c2 pawn with a black bishop would remove the lethal move from the white king, creating a need for yet another blocking piece at d2 or b4.

Improve this answer
$\endgroup$
6
  • $\begingroup$ Not the perfect score but the perfect crime ;-) $\endgroup$
    – Albert.Lang
    Commented Mar 9, 2021 at 12:11
  • $\begingroup$ The double masked bishop is cute! $\endgroup$
    – loopy walt
    Commented Mar 9, 2021 at 12:41
  • $\begingroup$ Ah, the Bishop-Rook-Knight is interesting. I didn't know you can chain the black pieces too! $\endgroup$
    – justhalf
    Commented Mar 9, 2021 at 19:04
  • $\begingroup$ Could you improve this by removing the white rook and putting the king at e5? $\endgroup$
    – Darrel Hoffman
    Commented Mar 9, 2021 at 19:11
  • 1
    $\begingroup$ @DarrelHoffman The goal of the puzzle is "maximum number of pieces with a task", so while the "king at e5" variant is way more efficient at mating the black king, effectiveness is the polar opposite of what we want :-) $\endgroup$
    – Bass
    Commented Mar 10, 2021 at 5:59
4
$\begingroup$

Maximizing number of accesories
Number of accessories: 19 pieces.
Regicide score: 19 - 3x4 = 7 points.

Cheap solution, which is the setup in the post, shifted a bit. The unique mate is Bg7#.
The four black queens are witnesses, the rest are accessories.
enter image description here
We might be able to add black rook at e7 for one more accessories by adding black pawns at f7 and e6 (and remove d7), but I think it's not a legal position (not reachable from initial setup).

Maximizing the regicide score
Number of accessories: 11 pieces.
Regicide score: 11 points.

Unique mate: c3#.
enter image description here

Improve this answer
$\endgroup$
6
  • $\begingroup$ Good start. It's interesting to see two strategies emerging. "No-witnesses" strategy (you and @Bass) and "mob" strategy (@Albert.Lang). I wonder which one will come out on top. $\endgroup$
    – loopy walt
    Commented Mar 9, 2021 at 12:35
  • $\begingroup$ You can easily improve max score a bit by moving white pawn to b2 or d2 and adding a black queen to c3. $\endgroup$
    – Zizy Archer
    Commented Mar 10, 2021 at 12:33
  • $\begingroup$ @loopywalt I think I managed to beat them at their own game (for the moment) while taking full advantage of that new definition of unique ;-) $\endgroup$
    – Albert.Lang
    Commented Mar 10, 2021 at 15:38
  • $\begingroup$ @ZizyArcher yeah, I mentioned it in another comment too. Decided not to change it since it's probably not maximal anyway. $\endgroup$
    – justhalf
    Commented Mar 11, 2021 at 3:41
  • $\begingroup$ @Albert.Lang nice. You're really into this =D $\endgroup$
    – justhalf
    Commented Mar 11, 2021 at 3:53

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.