-10
$\begingroup$

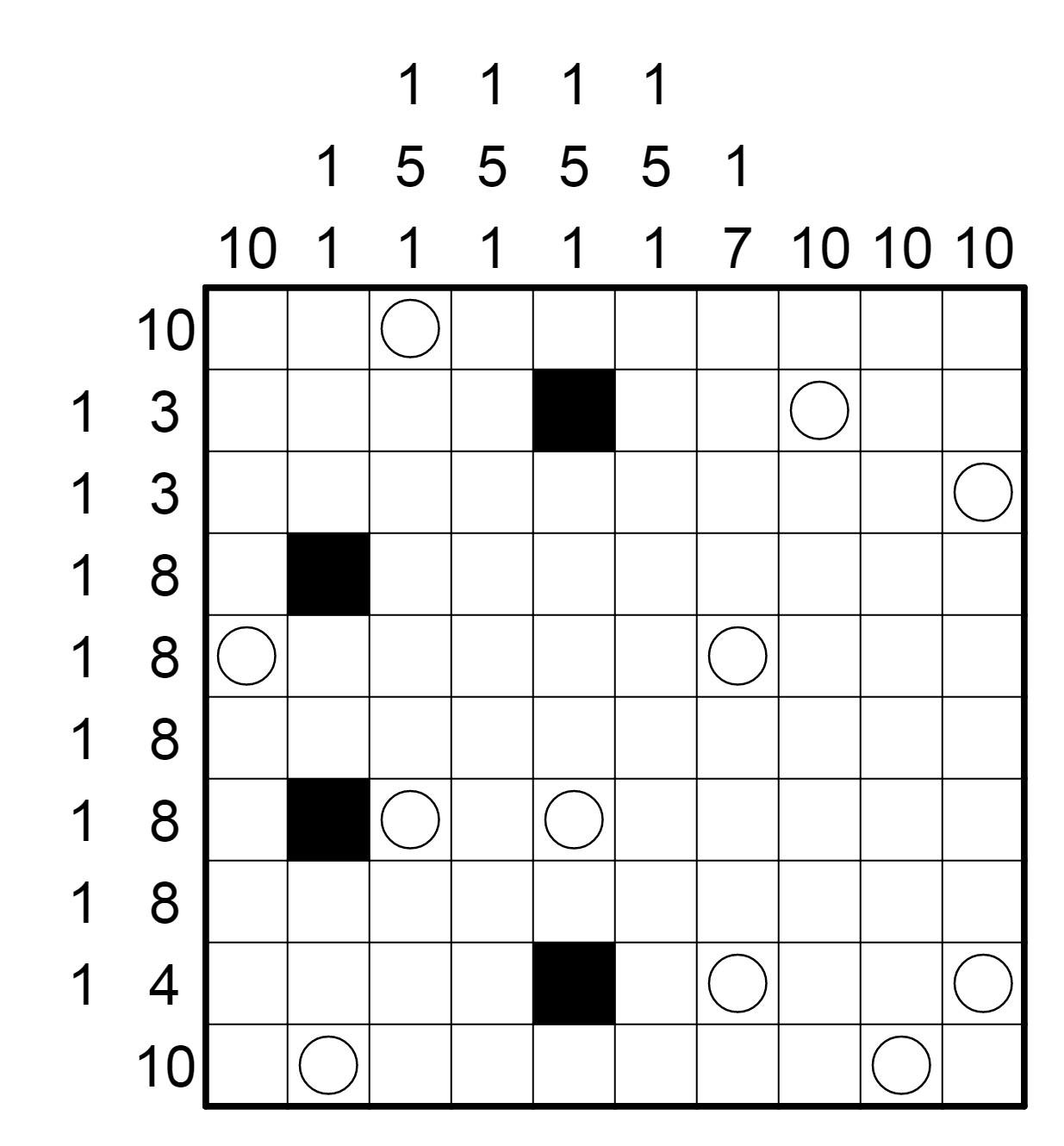
Introduction puzzle: here

So, my first Shikakui Hebi puzzle didn't go well, so I'm trying again! Only this time, no more arrows. Instead, it's now a nonogram-like!

This is a Shikakui Hebi puzzle. Rules of Shikakui Hebi are:

  • Solvers are to draw 1 big snake/a long line with twists and turns around a rectangular grid.

  • The snake is not allowed to form a loop, which means the edges of the snake cannot connect.

  • The snake always starts in the lower right corner.

  • The snake MUST NOT cross through shaded squares.

  • The snake MUST cross through circles.

New rules:

  • Outer numbers mean that there are that many squares that contain the piece of the snake.

  • If the numbers say 1 3, then it means that there is at least 1 empty square between the 1 square and the 3 squares.

Enjoy!

enter image description here

Improve this question
$\endgroup$
4
  • 4
    $\begingroup$ Are you sure the solution is unique? I can see the snake can be in many different positions, each makes a same solution. $\endgroup$
    – Anonymous
    Commented Jan 3, 2021 at 7:56
  • $\begingroup$ I checked, and the outer numbers make the solution unique. In fact, I would be happy if you tell me the other solutions in an answer so I can fix them. $\endgroup$
    – Anonymus 25
    Commented Jan 3, 2021 at 7:59
  • $\begingroup$ Look, being the puzzle like a nonogram, I can see no importance of the circles. Solving the nonogram directly gives you the path. Is there any relationship I am missing with the circles? $\endgroup$
    – Anonymous
    Commented Jan 3, 2021 at 8:04
  • $\begingroup$ The nonogram means that a piece of the snake is in that place, not the direction it's facing. The circles are supposed to help with that. $\endgroup$
    – Anonymus 25
    Commented Jan 3, 2021 at 8:08

1 Answer 1

Reset to default
5
$\begingroup$

There are a lot of solutions, the puzzle is not unique. I am only showing 2 of them.

Solution 1:-

Solution 2:-

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ Yikes, ok, I didn't expect that $\endgroup$
    – Anonymus 25
    Commented Jan 3, 2021 at 8:26
  • 5
    $\begingroup$ Also, a note. You got downvotes probably because for this reason that the solution is not unique. $\endgroup$
    – Anonymous
    Commented Jan 3, 2021 at 8:27
  • 1
    $\begingroup$ I missed that, sorry $\endgroup$
    – Anonymus 25
    Commented Jan 3, 2021 at 8:27

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.