Splitting figures on the Cartesian plane

Question

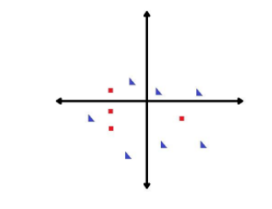
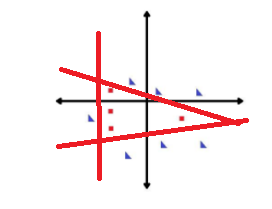
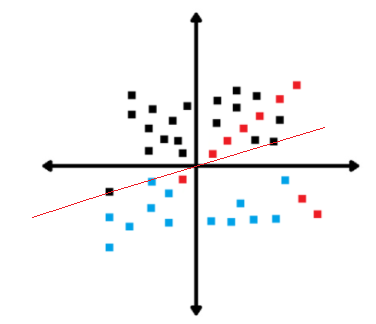
What is the minimum number of lines to separate the sets?

a) 2 b) 3 c) 4 d) 1 e) 5

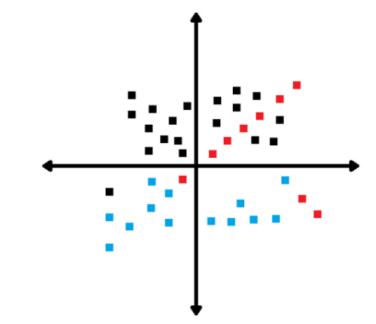
Observe the graph below, it is possible to separate linearly with a line at least two of the classes?

a) Yes, just pass a straight line on the vertical axis.
b) Yes, just pass a straight line on the horizontal axis.
c) Yes, with all points separated.
d) No, because the classes mix.
e) No, because there are four quadrants.

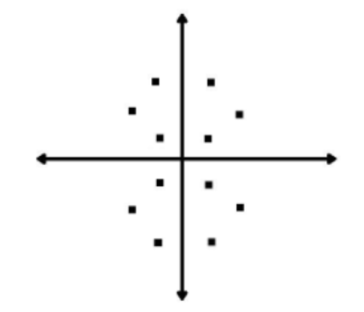
How many circles are needed to group all points into three groups with 2 points and a group with 4 points?

a) 3 circles
b) 5 circles
c) 4 circles
d) Points would remain
e) There is no answer

I believe the answers are

1. 3
2. No, because the classes mix
3. Points would remain

These challenges were proposed to me by a discreet math teacher and, as they are "challenges", I don't imagine that the answers are so simple.

Source

Answer 1

Answer 1

3, as follows

It's not too difficult to show that 2 is impossible. The four blue points near the y-axis cannot possibly be grouped together which necessitates at least two lines and the blue point on the left can be grouped with at most one other blue point which leads to the necessity for the third line.

Answer 2

If squares represent points with their centres then I think it is possible to linearly separate the black and blue classes as follows

but that does not seem to quite match up with the multiple choice.

Answer 3

3x2 + 4 = 10 so there are, at most, 10 points in our groupings but there are 12 points displayed. Hence, I think the appropriate answer is "Points would remain", although that is not much fun.