No.
If there is exactly one way to fill out the grid in a valid way, then that can be found without using any logic based on uniqueness. (It may be very painful, and require brute-forcing a lot, but it won't be impossible to find.)
If there are exactly two valid ways to fill out the grid, then uniqueness logic cannot help you. You can never make a deduction of the form "if X happens, then there are multiple solutions, so it can't be X" that rules out exactly one of the two solutions -- because all deductions of that form must rule out the multiple solutions including X!
If there are three or more valid ways to fill out the grid, uniqueness logic can give you any of them! In the extreme case, you can enumerate all possible solutions, and say: "It's either solution #7, or it's not solution #7. If it's not #7, then there are multiple solutions. Therefore it's solution #7."
So, no matter what, you can't make a puzzle solvable with only uniqueness logic. You might be able to make one that's easier with uniqueness logic, but not one that's only possible with it.
Of course, you may not be convinced by my last case there -- it seems very artificial. There are more natural examples that demonstrate the problem, though.
The paper "Uniqueness in Logic Puzzles" has a good explanation of why this is, and they give a particularly good example of how uniqueness logic can go wrong when there are multiple solutions. They give this Slitherlink puzzle, that has exactly three possible solutions:
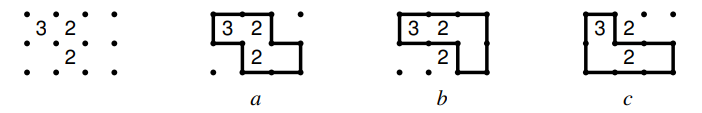
And then show that, depending on where you check for uniqueness, you'll get to a different solution. If you check a segment near the top left, you'll find that solution (c) is 'the correct one'; if you check a segment near the bottom right, you'll find that solution (b) is 'the correct one'.
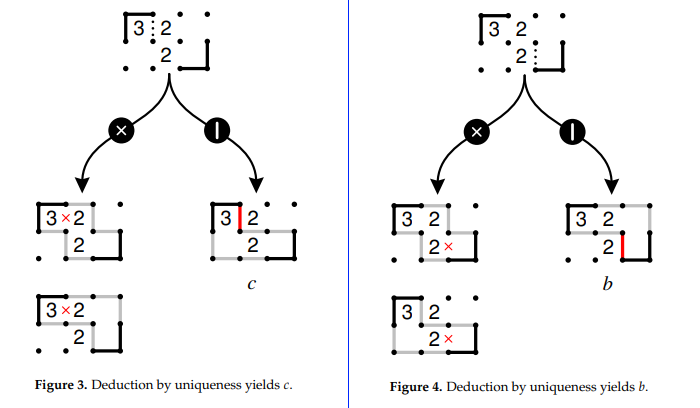
This is a relatively trivial example, but this could be embedded as one corner in a larger puzzle (with the loop escaping in the bottom right rather than wrapping around). If it was embedded like that, an experienced Slitherlink solver could very easily notice one of those. And if they used uniqueness logic, they would get either solution (b) or (c) without ever realizing something was wrong.
It may be possible to have only one "natural" solution if you assume uniqueness: that is, a "natural" set of uniqueness deductions will lead to only one of the solutions. But now we've left the realm of pure logic, and you're looking for something that will be "natural" to every single solver -- this would be very subjective, and unlikely to work for all but the most trivial examples.


grid-deductiontag. $\endgroup$