Filling numbers in a grid

Question

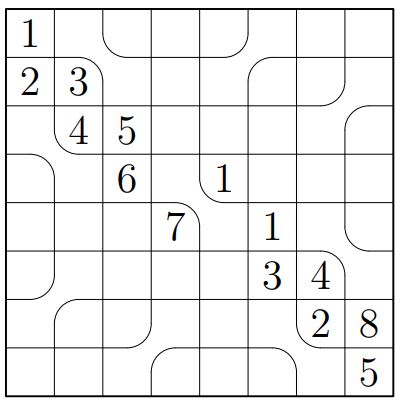
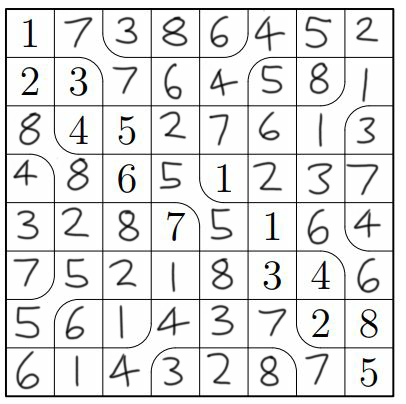
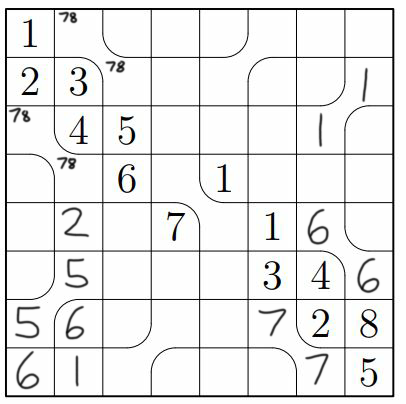
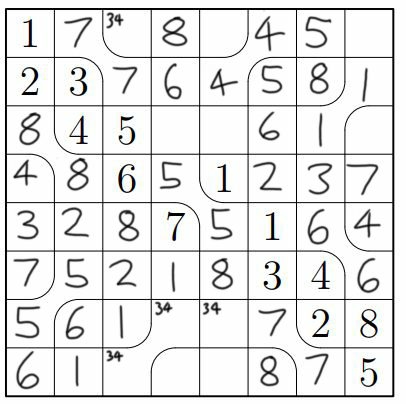
Here's the problem and the diagram that goes with it

Fill in each empty space of the grid in the image below with a number from 1 to 8 so that every row & column contains each of these digits only once. Some diagonally adjacent spaces have been joined together. For these pairs of joined spaces the same number must be written in both.

Diagram:

A substitute teacher provided us with this puzzle. Can you please provide an explanation of how to get to the answer?

Answer 1

The solved grid:

And here's how to solved it:

---

STEP 1:

Look at the conjoined cells on the right at the bottom. These are 'seen' by all numbers except 6, so must be 6. This fixes 6s in the bottom left, meaning three other conjoined cells are 'seen' by all but 1 number, which we can then place.

STEP 2:

Look at the ones. All the ones can now be placed through normal rules. This then allows us to fill out some rows/columns and hence place a few others through deduction.

STEP 3:

Now look at the sixes. These can all be filled in. Filling in certain rows allows us to place numbers where we had two candidates, as one of the pair is ruled out.

FINAL STEP:

From here, the rest of the cells can be filled in simply by looking at what remains in each row/column and voila!