18
$\begingroup$

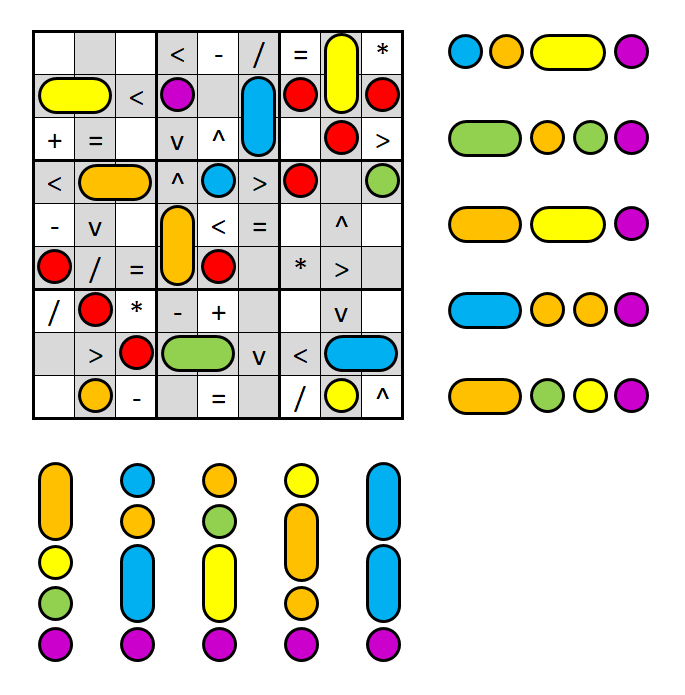
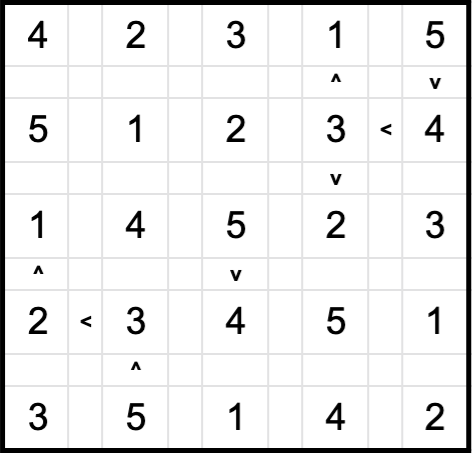
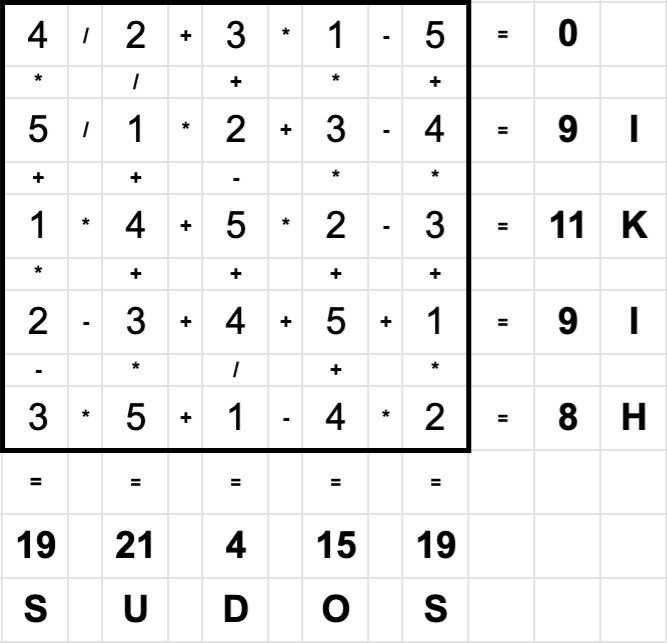
I believe I have invented a new type of puzzle...

What is its name?

enter image description here

Colour-blind-friendly version available here.

Begin by solving the 9x9 sudoku; each of the 9 symbols /, *, +, -, =, ^, >, v and < must appear exactly once in each row, column and thick-bordered 3x3 box. Then apply some (!) and discover its name!

Hint 1:

Every colour used in this puzzle is there to help you solve it, whether for deriving and solving the second-stage grid-deduction puzzle or deriving its name. Every colour...

Hint 2:

then then

Other puzzles in the 'This new puzzle type needs a name' theme: 01, 02, 03, 04

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ I think it's time for another hint $\endgroup$
    – ThePuzzlingPlatypus
    Commented Feb 22, 2020 at 21:28

2 Answers 2

Reset to default
12
$\begingroup$

First...

... solve the Sudoku, as instructed.

Credit to dcfyj and Kevin Cruijssen for solving this step first.

The completed grid, as depicted by Kevin Cruijssen, looks like this:

The completed Sudoku grid.

Next...

... solve the 5x5 Futoshiki puzzle formed by the white squares and the red-circle inequality symbols between them.

The finished grid looks like this:

The completed Futoshiki grid.

Finally...

... we apply the color-coded arithmetic symbols to each row and column. There are four symbols in each row/column (plus an equals sign), which fits perfectly!

Standard order of operations apply. The completed grid reveals our answer.

The completed arithmetic.

The new puzzle type's name is...

SUDOSHIKI.

Great construction, Stiv!

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ Great job at wrapping this up, mbingo :) Could you include the sudoku image from the comment by @KevinCruijssen (with credit, of course) at the start of your answer for completeness? That would make for a nice comprehensive solution... Then the checkmark will be yours! Thanks :) $\endgroup$
    – Stiv
    Commented Feb 24, 2020 at 15:18
  • 1
    $\begingroup$ Done! And again, excellent puzzle (and series)! $\endgroup$
    – mbingo
    Commented Feb 24, 2020 at 15:30
  • 2
    $\begingroup$ Brilliant, thanks mbingo. I figured Kevin's great diagram needed to be given some 'airtime' rather than being hidden away in the comments. This rounds it off nicely. Have a checkmark! $\endgroup$
    – Stiv
    Commented Feb 24, 2020 at 15:35
  • $\begingroup$ How are you fill color slots after the first step? $\endgroup$
    – Nick
    Commented Aug 25, 2020 at 0:19
  • $\begingroup$ Hi @Nick - the symbols in the grid are the ones which result from solving the sudoku (using the symbols instead of numbers 1-9). You then fill the coloured patches around the outside with the symbols from the corresponding region of the same shape & colour within the grid. Hope that helps! :) $\endgroup$
    – Stiv
    Commented Aug 25, 2020 at 8:56
5
$\begingroup$

Partial Solution:

Here's the Sudoku solved

Solved Sudoku with colors

So far I'm not really seeing any viable paths using both the colors and the solved Sudoku symbols. I do see that red

only uses > < V for its symbols.

Which I find interesting, but I'm not sure how to use it. Also, because of the first hint I noticed that

every color clue rests on a gray line, which implies to me that they all should, but if I use the arrows as viable color slots I have too many options, and if I use the arrows for red highlights only, I have too few for the other colors (provided I only place them on the gray)

Which basically just says, I'm still at a loss lol but at least I feel like I'm making a little progress

Improve this answer
$\endgroup$
4
  • 4
    $\begingroup$ Ah, you beat me to it. Was working on it as well: [spoiler] i.sstatic.net/X983n.png [/spoiler]. ;) $\endgroup$
    – Kevin Cruijssen
    Commented Feb 17, 2020 at 18:29
  • $\begingroup$ Oh! thanks for the clue, @KevinCruijssen, I hadn't thought about doing that $\endgroup$
    – dcfyj
    Commented Feb 17, 2020 at 18:40
  • $\begingroup$ I'd say that finding in your last spoiler (right now) may well be puh-retty relevant... Keep going! $\endgroup$
    – Stiv
    Commented Feb 19, 2020 at 15:52
  • $\begingroup$ @Stiv I figured it was, I'm just not seeing how at the moment. I'll give it a proper look see later today when I have time. $\endgroup$
    – dcfyj
    Commented Feb 19, 2020 at 18:16

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.