8
$\begingroup$

Adapted from Professor Stewart's Casebook of Mathematical Mysteries*

I'll present three puzzles, in stages. Try not to look at the next stage before completing the previous one.

Note that 'a line of four stones' means a line with exactly four stones.

Stage 1

Place twelve stones on a table such that there exist six lines of four stones.

Stage 2

Place twelve stones on a table such that there exist seven lines of four stones.

Stage 3

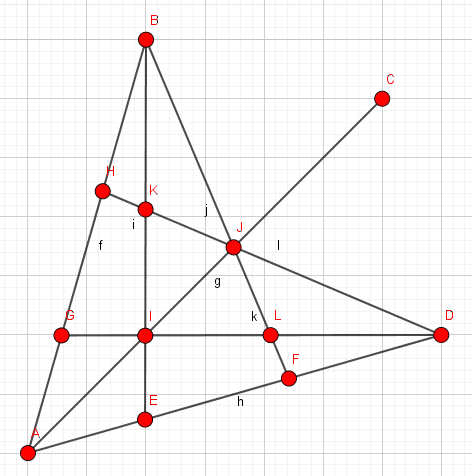
Move four stones from a certain configuration such that there exist seven lines of four stones. To make the initial configuration, intersect two equilateral triangles to make a six pointed star and put a stone at each intersection of two lines.

*(super duper) highly recommended for anybody who likes mathematical puzzles. Although it is ever so slightly more on the maths side than the puzzle side, there are quite a few enigmas. In fact, there are many other interesting problems and content in the book (e.g insert a mathematical symbol between $4$ and $9$ to make a number between 1 and 10.). I was just wondering if anybody'd be interested in more 'mathematical puzzles' from that book.

Dunno what to tag this, feel free to change the tags (and this message)

Improve this question
$\endgroup$
1
  • 2
    $\begingroup$ Is this the literal riddle? Given this wording, and the fact that all the other answers also allow stones to be part of several lines at once (obviously), I would put the 12 stones in a row. I would then have rows that cover stones no. 1-4, 2-5, ..., 9-12, that is, 9 rows of four stones. And if I take rows of four stones that do not have to be next to each other, I believe I even have ${12\choose 4}$ different rows of four stones there. That is 495. $\endgroup$
    – Marie. P.
    Commented Nov 19, 2017 at 16:45

4 Answers 4

Reset to default
14
$\begingroup$

Solution for stage 1

enter image description here

Solution for stage 2

enter image description here

Solution for stage 3

enter image description here

Improve this answer
$\endgroup$
2
$\begingroup$

Partial answer

Stage-1

            *

      *   *   *   *

        *       *

      *   *   *   *

            *

That is ,

Stones arranged in the shapes of two overlapped equilateral triangles, each side having 4 stones. One triangle right up and the other one up-side down. That is the final shape is a star with one stone each at vertices and at intersections of sides of triangles.

Improve this answer
$\endgroup$
2
$\begingroup$

I believe I did find an alternate solution for stage 1 based on squares instead of triangles:

enter image description here

Improve this answer
$\endgroup$
0
$\begingroup$

I haven't solved stages 2 or 3 yet, but I have an alternate solution to stage 1 that I think is mathematically different from the ones already given:

Stage 1:

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ I'm sorry... I'm fairly certain that, in some sense, it's the same solution as it's just two equilateral triangles with parallel sides, with stones placed at each intersection of any two lines (this time, the lines are extended as opposed to last time) $\endgroup$
    – Wen1now
    Commented Nov 20, 2017 at 1:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.