7
$\begingroup$

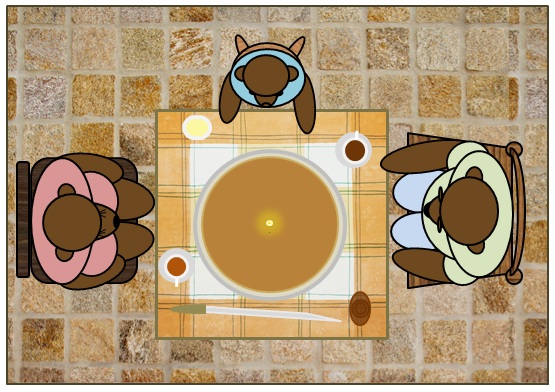
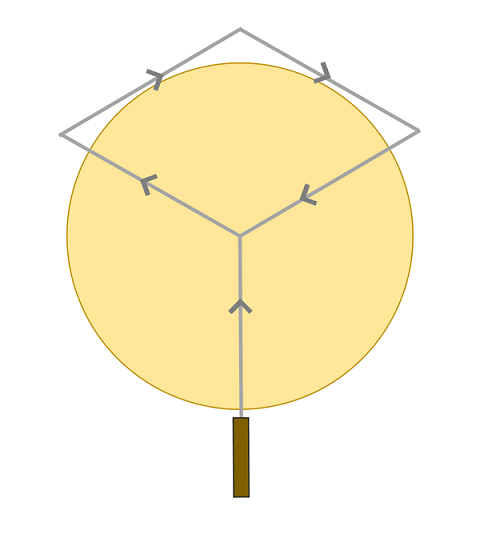
enter image description here

The three bears are regular customers at the Goldilock's (see illustration). When Goldilock brought the cake as ordered by the Bear family, Little bear asked her if she can divide the big round cake into 3 equal pieces. So the girl marked 3 equally distanced points along the cake's perimeter. Then made 3 straight cuts with the tip of the knife on the middle (removing the candle first) and slicing through the markers.

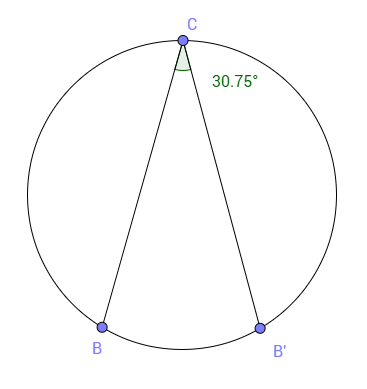
After the bears had eaten their equal shares, they ordered another whole cake. The young bear was hurrying so he asked if Goldilock can divide it again equally as before, but this time with just two cuts. To do that, she bent the knife into a 120 degrees angle and sliced down through with the knife vertex on the center where a candle was to make a 1/3 piece. Next, she divided the bigger part exactly in two for the 2nd cut.

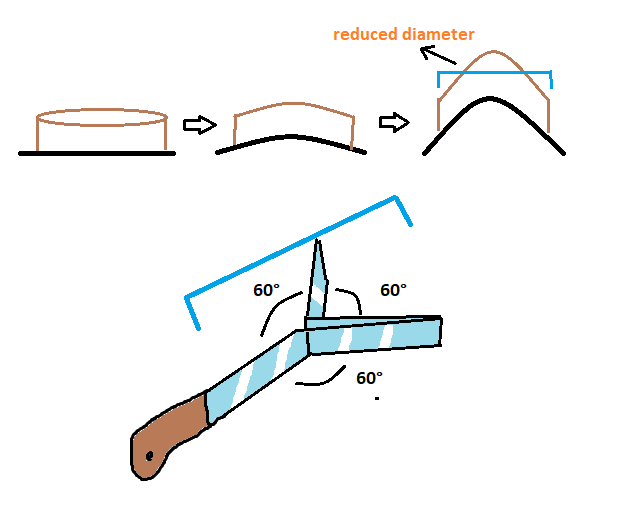
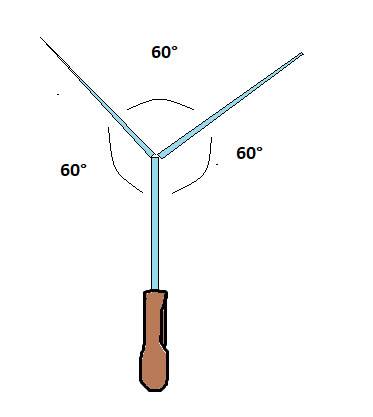
After the bears had eaten their equal shares, they ordered another whole cake. The young bear was really in a hurry so he asked if Goldilock can divide it again equally as before but this time with just one single cut. While she hopes that that was the last order they made today, she gladly did as the bear requested. How did Goldilock manage to do it with one straight cut?

Improve this question
$\endgroup$
6
  • 5
    $\begingroup$ (Incidentally, nice top view of the candle! And fine artwork in general.) $\endgroup$
    – humn
    Commented Mar 5, 2017 at 17:43
  • 7
    $\begingroup$ @humn The illustration was done with excel.. $\endgroup$
    – TSLF
    Commented Mar 6, 2017 at 13:18
  • $\begingroup$ Why couldn't Goldilock cut the cake horizontally through the height of the cake? $\endgroup$
    – Mr Pie
    Commented Mar 25, 2018 at 0:26
  • $\begingroup$ I came across this quite old puzzle but can't find an accepted answer or an explanation. Does one exist? $\endgroup$
    – puck
    Commented Jul 22, 2023 at 18:25
  • $\begingroup$ @puck As best I can tell, TSLF self-answered (but didn't mark accepted), involving folding the cake before cutting it. Otherwise I propose Goldilock use the bent knife as before to cut a slice, pause the cut while lifting the slice onto the other two thirds, and then finish the cut to divide the second piece in half. $\endgroup$
    – Amoz
    Commented Jul 27, 2023 at 4:46

9 Answers 9

Reset to default
14
$\begingroup$

Not sure if this is a valid solution either, but

Goldilock can fold the knife according to the diagram below:
enter image description here
The shape of the knife outside the cake (the two diagonal segments on the top) does not matter, since it won't cut anything. The arrows are there to show the path of the knife; the knife ends at the center of the cake where it meets the point of the first fold.

Improve this answer
$\endgroup$
2
  • $\begingroup$ There can't be another knife..but the bent knife that is just a little longer than the diameter of cake. (refer to illustration).She can actually use the bent knife or she can straighten it again for use. $\endgroup$
    – TSLF
    Commented Mar 6, 2017 at 13:28
  • $\begingroup$ @TSLF Ah alright, I had assumed the knife could be as long as we wanted. Oh well :P $\endgroup$
    – DooplissForce
    Commented Mar 6, 2017 at 13:39
11
$\begingroup$

I don't know if this is a valid solution, but with lateral thinking puzzles you can never be 100% sure:

You can do this division here in two ways:
enter image description here
Either by bending a really long knife in that shape ($BCB'$) or by folding the cake in half and do one straight cut along $BC$.

Improve this answer
$\endgroup$
5
  • 3
    $\begingroup$ I hope it's your second option! (And while at it, why not fold the entire puzzle in half?) $\endgroup$
    – humn
    Commented Mar 5, 2017 at 15:04
  • $\begingroup$ Equal weight is fine but to divide equally as before suggest 1/3 pieces of 120 degrees slices. $\endgroup$
    – TSLF
    Commented Mar 5, 2017 at 15:16
  • $\begingroup$ @TSLF Yeah I wasn't sure if the pieces had to have the same shape or not, but I decided to post this answer anyway. $\endgroup$
    – FrodCube
    Commented Mar 5, 2017 at 15:20
  • 2
    $\begingroup$ @FrodCube your answer got the hard part of the puzzle somehow $\endgroup$
    – TSLF
    Commented Mar 8, 2017 at 15:26
  • $\begingroup$ Checked for second option. Still open for the solution. $\endgroup$
    – TSLF
    Commented Apr 6, 2017 at 15:37
6
$\begingroup$

As long as we are allowed to bend the knife and it is at least as long as twice the diameter of the cake, then bend the knife into a U shape (where the curve extends beyond the diameter of the cake) and slice the knife through the edge of the cake's cylinder - rather than downward against a face of the cake.

The knife, against a sufficiently firm cake, will slice the single layer cake into a 3-layer cake where each slice is of the same size and mass.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Against a squishy cake, you'll end up with a mess more like Goldilocks' typical porridge. $\endgroup$
    – Lonnie
    Commented Mar 5, 2017 at 21:04
  • $\begingroup$ A good way to divide a sufficiently firm cake in 3 equal parts unless the knife is just as long as shown. $\endgroup$
    – TSLF
    Commented Mar 10, 2017 at 17:40
2
$\begingroup$

I think bending the knife in the following fashion would get portions which could be divided among the three bears. enter image description here

Improve this answer
$\endgroup$
1
$\begingroup$

She broke knife in half (removing the handle) and placed the 2 pieces together, but one in each hand. She then cut from the edge before separating the blades when reaching the centre of the cake.

Improve this answer
$\endgroup$
0
1
$\begingroup$

So that's my solution. Prerequisite : the cake has to be extremely elastic and resistant, and the knife should be something like the Goemon's zantetsu-ken nagareboshi katana. The idea is pretty simple btw, putting the cake in a curve surface (I'm not a mathematician so I don't know how much exactly the surface has to be curved) its complessive diameter will be reduced if we look the cake from a top-down perspective (the same of the cutting knife). In that way, the length of the bent blade (see reference picture) will be enough to cut the cake in 3 equal parts.

enter image description here

Edit: you could also simply slice the second half of the blade in two divergent blades forming three 60° angles

enter image description here

Improve this answer
$\endgroup$
0
-1
$\begingroup$

She marked 3 equally distanced points x,y,z along the perimeter. Then warp the hot cake so that all the markers meet at a point. Slicing straight from center to meeting point could fairly divide into 3 equal size and shape pieces. enter image description here

Improve this answer
$\endgroup$
6
  • $\begingroup$ Dunno how you can do this in a hurry! $\endgroup$
    – boboquack
    Commented Apr 10, 2017 at 9:58
  • $\begingroup$ she got 120 degree bent knife..if you are referring to marking time $\endgroup$
    – TSLF
    Commented Apr 10, 2017 at 15:52
  • $\begingroup$ How she can 'warp the cake' in a hurry $\endgroup$
    – boboquack
    Commented Apr 10, 2017 at 21:56
  • $\begingroup$ It' was the bear who is in a hurry..Anyway she, making 3 markers meet is fast enough. 1) warp /fold the round flat cake so that 2 of the marks (y & z) coincides. 2) warp/bend again so that radius (c-x) is aligned with radius c-(y,z). $\endgroup$
    – TSLF
    Commented Apr 11, 2017 at 15:51
  • $\begingroup$ Um. Have you ever tried doing something like this to a cake? [They don't bend like that, even when hot.] $\endgroup$
    – Sneftel
    Commented Mar 28, 2018 at 11:38
-1
$\begingroup$

I don't think anyone has suggested this yet.

The cake is a layer cake with two layers. Goldilocks cuts of a piece size 1/3 and then splits the remainder by separating the top and bottom layers, so the 2/3 piece becomes two 1/3 pieces.

Improve this answer
$\endgroup$
-1
$\begingroup$

Goldilock bends the knife to 90o. The cut is initiated by passing through the outer two-thirds from top to bottom, and then continues half way around the cake before twisting the knife 180o and rotates it 180o before finishing the round cut, leaving one-third in the centre and two one-third pieces on the outside. This cannot be a straight cut per say, but a single cut with a twist of the wrist between each part of the action.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.