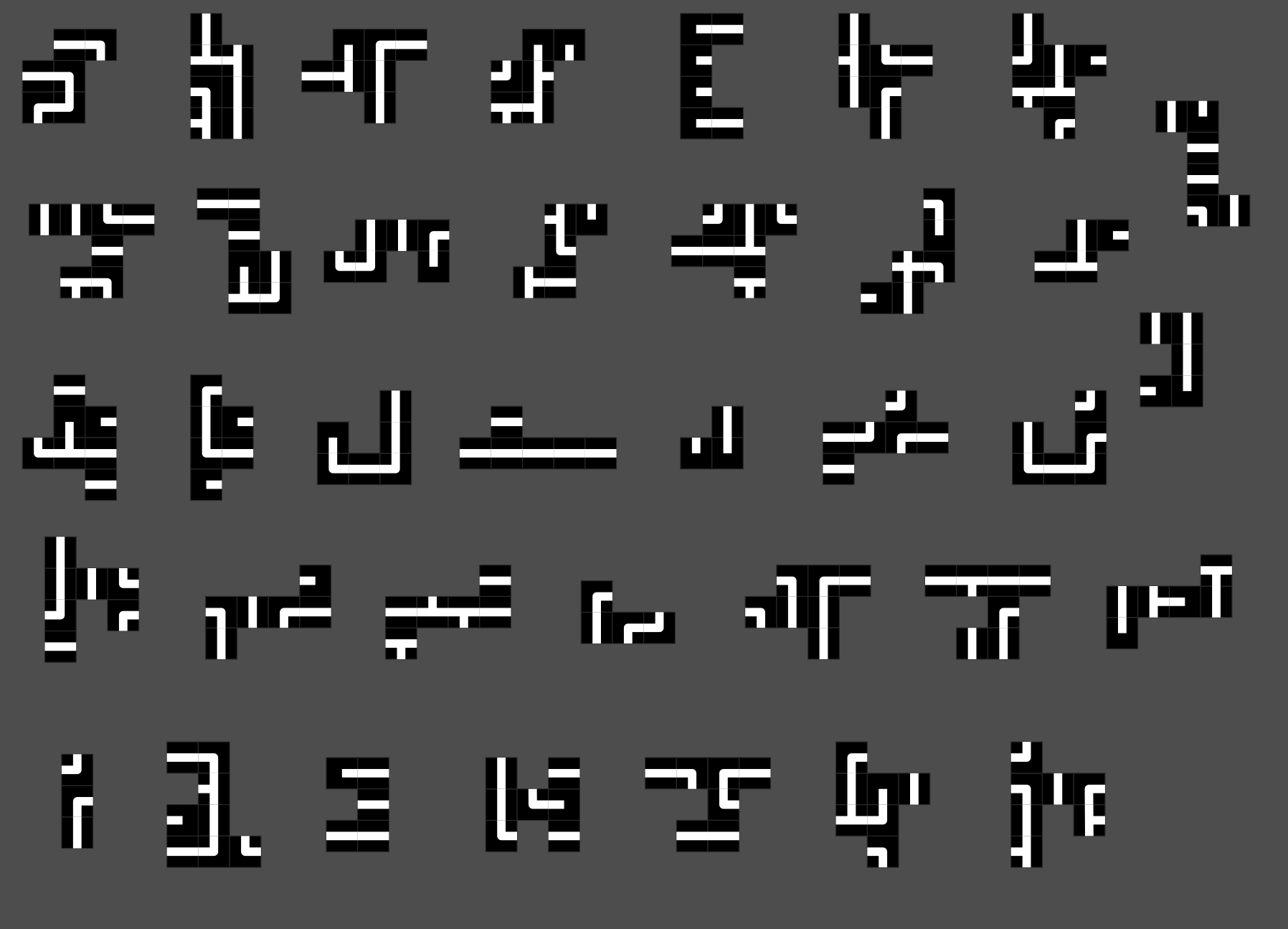14
$\begingroup$

This is an entry to the 12th fortnightly challenge

"No, No, No! You are smart, I give you that, but finding your way through the previous mazes will not free you from my fury!"

Sigh, and there you thought to have seen the end of it...

"So, what is then, mad wizard, what you have served us this time?"

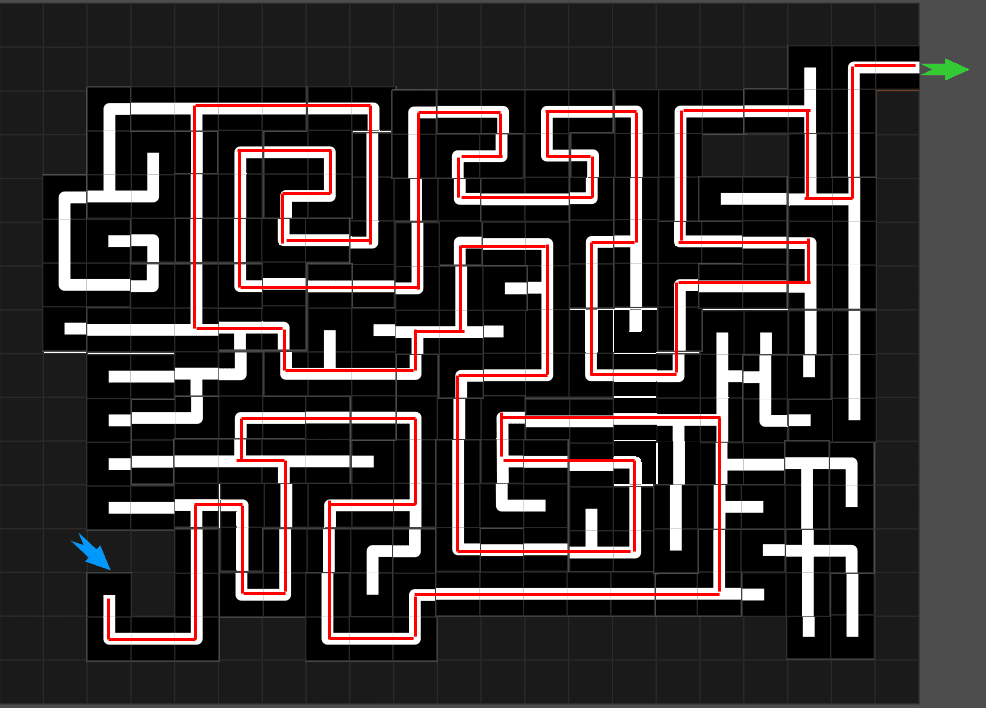
"Oh, hi hi hi, nothing special. Just a nice big cavern with a single entry and a single exit. Look!"

A blue shimmering doorway opens before you and gives the view onto a corridor before you. Heat flashes into your face.

"Of course, I hope you don't mind a bit of lava flooring? Ha Ha Ha."

"Very funny, old man. So, where's the trick? You don't expect us to get cold feet, do you?"

"Oh no, this time I'm very generous. I let you built the maze yourself! I'm giving you a set of 37 magical tiles. Assemble them such that the fill the cavern exactly, and the shown pathway will appear for you. Of course, if you do it badly, there might not be a way out of tis cavern ever... ha Ha Ha Ha HA !"

The Lava Pit

The tiles

The puzzle is rather straight forward: Assemble all the tiles so that they:

  • Don't overlap anywhere
  • Fill all lava tiles completely
  • Produce a continues (white) path from the blue to the green arrow

Also note: The path starts in the centre of the first lava-tile the blue arrow points to. It does not need to connect to the wall the arrow points at exactly.

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ Are we allowed to have disconnected white paths as long as there path from start to exit is uninterrupted? or do all white paths have to be connected? $\endgroup$
    – crcroberts
    Commented Jul 17, 2016 at 20:59
  • $\begingroup$ @crcroberts Good question. The intended solution has a continuous path, but I don't know if it is unique. I would allow a disconnected white path solution as 'inferior' but valid, as long as the tiling is complete. $\endgroup$
    – BmyGuest
    Commented Jul 17, 2016 at 21:10
  • $\begingroup$ Is there more than one correct answer? Just curious $\endgroup$
    – Areeb
    Commented Jul 17, 2016 at 22:17
  • $\begingroup$ Can white paths run into walls? $\endgroup$
    – DooplissForce
    Commented Jul 17, 2016 at 23:54

1 Answer 1

Reset to default
9
$\begingroup$

The solution (assuming it's unique) appears to be:

final maze

I feel like I should include some sort of explanation, but given the hint, and an assumption that no path would butt into a wall, there were a few key pieces that had limited ways to fit together, and it all kind of fell into place without too much difficulty after that (would've been significantly more difficult if rotation were required).

Improve this answer
$\endgroup$
3
  • $\begingroup$ Yeah, I wanted to keep it simple this time in order to not have too large a searching field. Didn't consider the power of the puzzlers here on site correctly, it seems :-) $\endgroup$
    – BmyGuest
    Commented Jul 18, 2016 at 18:34
  • 2
    $\begingroup$ Ah, false assumptions all the way down on my part... I had ruled out the very first piece as it appeared to start in a mandated dead end (and thus not connect with the start tile); this lead me to believe that paths had to be able to end abruptly at walls... yeah basically 100% wrong. Well done Alconja! $\endgroup$
    – crcroberts
    Commented Jul 18, 2016 at 21:20
  • $\begingroup$ @crcroberts Hm, an interesting thought. I should have made it a bit more clear, I guess. Noted for the next time. Thanks. $\endgroup$
    – BmyGuest
    Commented Jul 19, 2016 at 6:17

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.