A friend of mine is a professor at a nearby university. Last week, he gave three of his students a simple assignment: conduct an experiment (all three of them separately and independently from each other), and record the development of a single value over time. And indeed, a few days later, all of them handed him a diagram, as seen below (here edited into a single image):
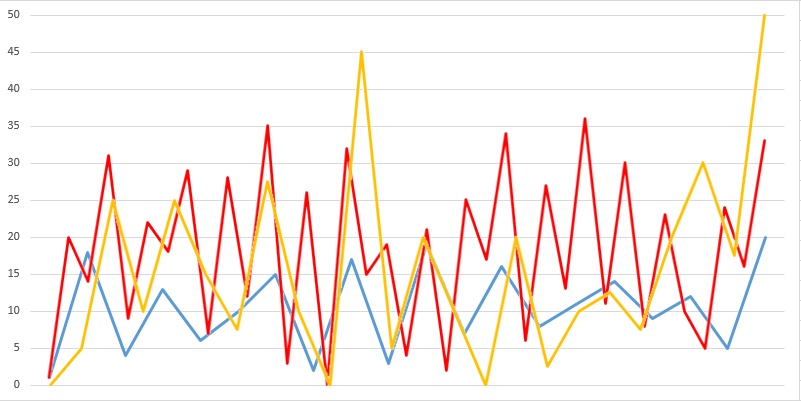
This came as a bit of a surprise for my friend. All three students were known to be incredibly lazy, and he half expected that least one of them spent his time drinking, gaming, watching TV or doing something else not work-related. Could it be that they took their assignments seriously this time? They would not just make up a few data points - would they? Although he coulnd not quite put his finger on it, my friend knew that there was something wrong with this data. Someone is going to be in trouble for this.
So, can you tell me who my friend will order into his office tomorrow?
Student 1 (Blue line)?
Student 2 (Red line)?
Student 3 (Yellow line)?
Or even more than one of them?
A few clarifications:
All three students performed the same experiment, but separately from each other. The different number of data points, different starting values, all-integer-values (except for student 3) are all normal for this experiment and not reasons to suspect foul play.
All data values are integers, except for yellow, who has a few .5-values. Again, something that can happen in this experiment.
As you have already noticed, student 1 has all numbers from 1 - 20 in his results. That is somewhat unlikely, but not impossible in the experiment. Now if he had 1 - 20 in ascending order, that would be a reason to call him in.
This has nothing to do with statistics. Rather, all suspect lines follow a specific, but not too obvious pattern that screams "I was copied from somewhere".
Hints:
Student 1 is 21 and British.
Student 2 is 25 and from a rich family with French roots.
Student 3 is 52 and American.
