Ten thousand tiles are arranged in a $100\times100$ square. Each tile is colored red, blue, yellow, or green, so that each $2\times2$ (contiguous) sub-square of the square contains all four colors.
Question: What are the possible color combinations for the four tiles in the corners of the square?
The entire square can be divided into 2500 2x2 squares, so it contains 2500 of each color.
If we ignore the first and last columns, we have a 98x100 rectangle which can be divided into 2450 2x2 squares. It must contain 2450 of each color.
Similarly, if we ignore the first and last rows, we have 2450 of each color.
Finally, if we ignore all four edges, we have a 98x98 square, which has 2401 of each color.
If we start with the original square, add a copy of the 98x98 square, and remove both of the rectangles, we are left with just the corners. So the corners must be 1 of each color.
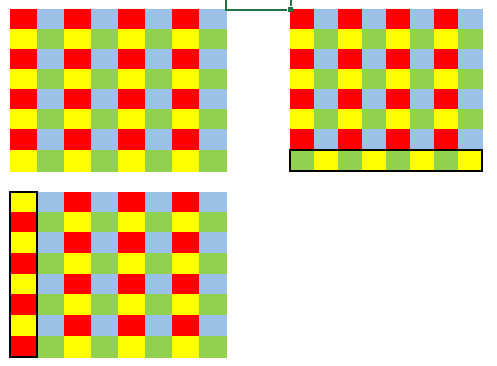
Not really any better than the others but a different way to look at it:
Consider two squares in the top row colored A and B. Then the two squares below that are C and D in some order, and below that A and B in some order, then below that C and D, etc. Eventually, in the bottom row, you have a C and D.
What this means is if you remove the whole bottom row and place it on top, the square will still satisfy the key property (of each $2 \times 2$ subsquare having four colors).
By symmetry, you can then remove the whole right side column and move it all the way to the left, and it still satisfies the key property.
Now the squares that use to be in the four corners are located in the first $2 \times 2$ subsquare in the upper left, and thus it is obvious that they are four different colors.
The answer is:
One of each colour
Because:
Given the even number of rows and columns, this means that:
Whichever rows OR columns you swap either:
The first column begins and ends with colours A and D and every even column begins and ends with colours B and E (either way round)
OR the first row begins and ends with colours A and B every even row begins and ends with colours D and E Leaving every corner with one of the ABDE colours we started with.
Resulting in 24 possible permutations (4!)
