Lepidopterology needed!

Question

This puzzle belongs to the puzzle series: hyper-modern art

---

The two friends in the gallery of Hyper-modern art are still discussing.

"You know what, to certain extent I start enjoying this Hyper-modern art, but I still think it lacks something important."

"And what should that be, my friend?"

"Beauty. Aesthetics. It is all well and good that the observer has to fully engage for deeper understanding, but I still would like to, well, like an image. It should be pleasing to watch."

"Hmm, yes, I get your point. But maybe the next example is more to your liking."

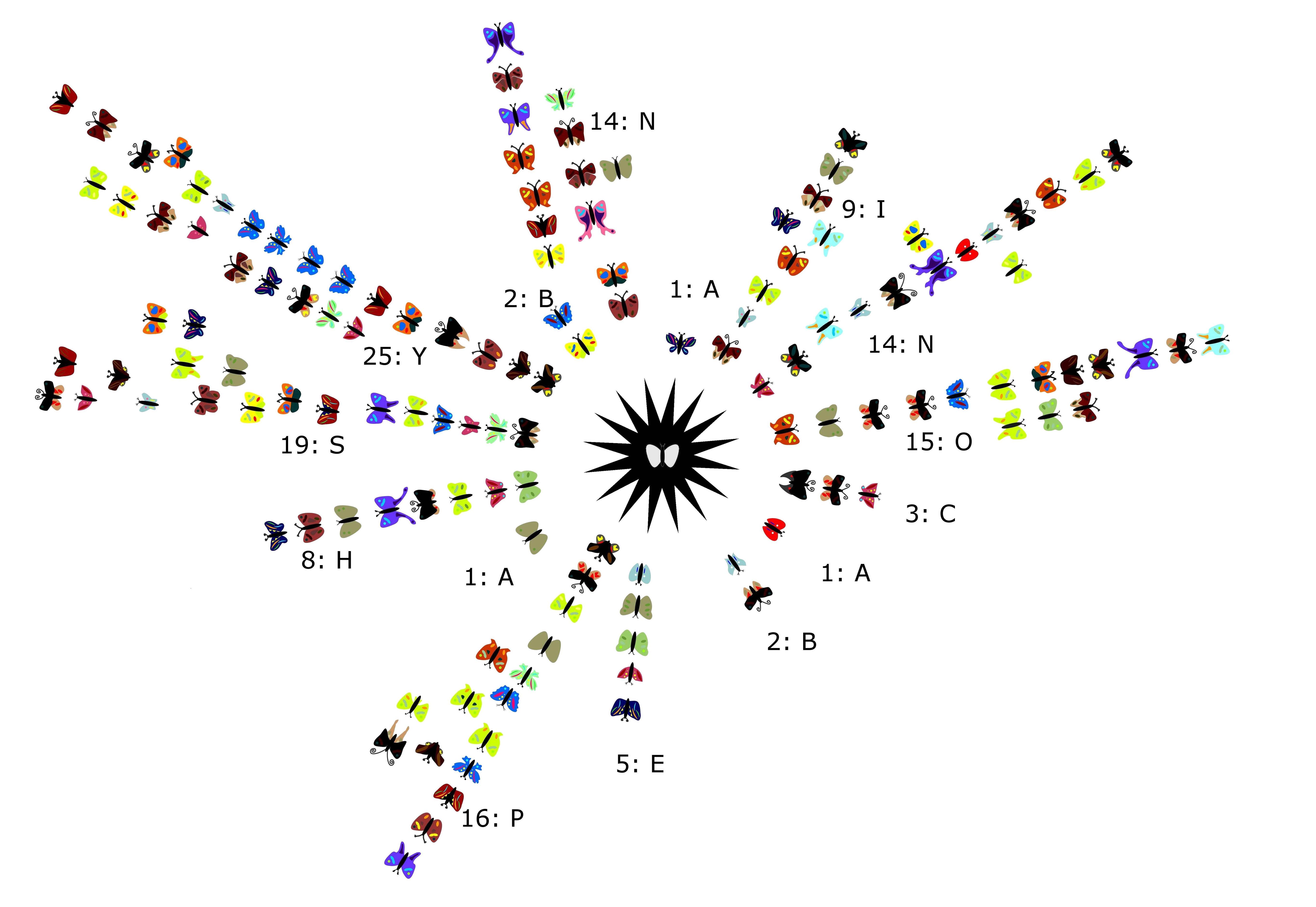
They move on to the forth room of the gallery, where a gigantic painting of 11x16 metre fills the whole side of the room.

"See what we have here: The painting is called 'Nature tells a story', and I think it is rather pleasing, don't you?"

"Well, it for sure is impressive... And yes, it is also nice. But what is the story nature wants to tell us here?"

"See, now we need our HUD again..."

---

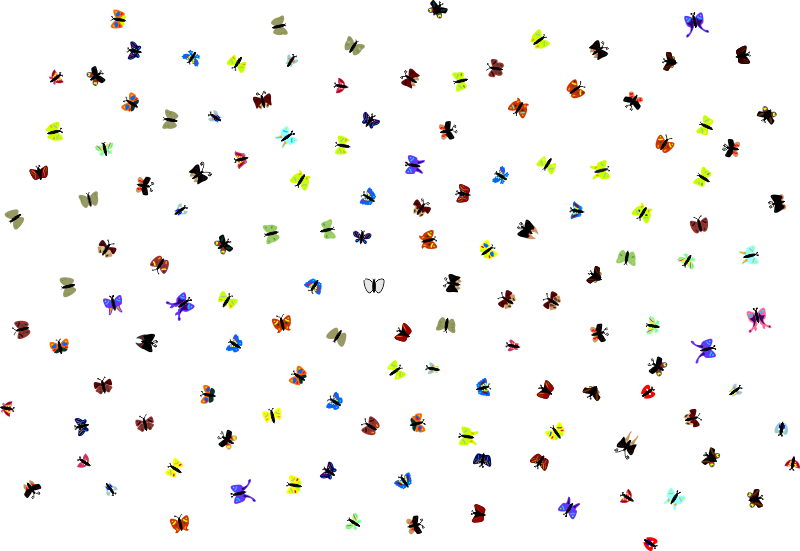Full resolution image, 1.3Mb, 6582 x 4539 pixels

---

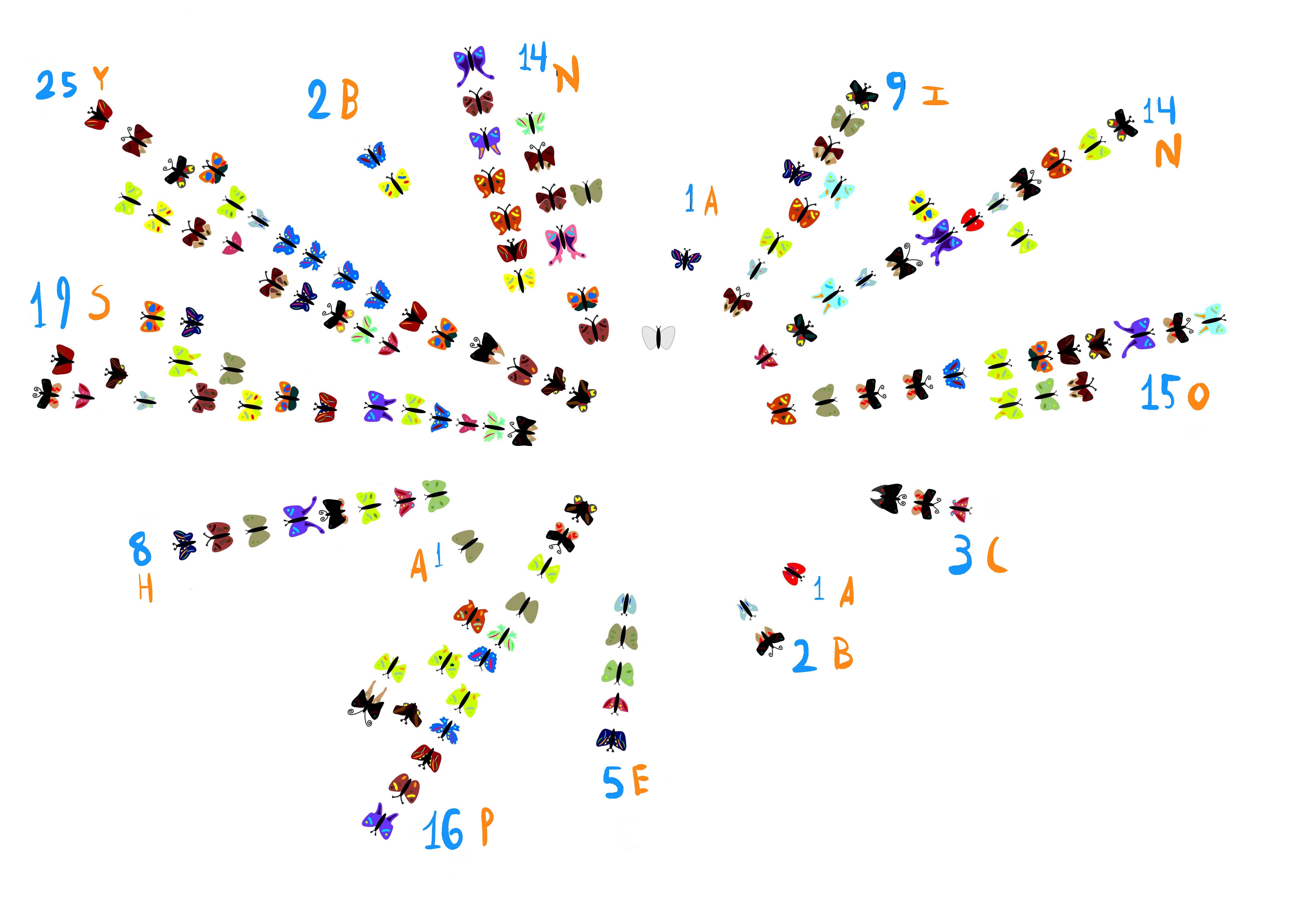
The goal of the puzzle is to find the message, this painting is telling us. This message is written in English and consists of five words. The puzzle will likely require some patience and work, and it does require the full-resolution image provided by the link.

While the full resolution is needed to see all details, the puzzle does not require any digital information. You can print the image and still solve it from that.

Bonus: An additional message is hidden in the image. This message is a clue for the actual puzzle. What is the message?

---

Hints

Hint 1:

You need a hint? Count!

Hint 2:

You think the flying direction of butterflies is random? Think again!

Hint 3:

A single butterfly (in one direction). But two?

Hint 4:

Have you taken a close look at hint 3 above?

Answer 1

The solution is

Darwin was right no creationism

Steps to the solution

1. Finding the hint

The butterflies have to be arranged according to their orientation. There are 16 different orientations (17 if you count the grey ancestor-butterfly in the center, but it can be ignored for this), evenly distributed with 22.5° difference each. Based on JTL's arrangement, slightly altered here, the first hint becomes visible (reading counter-clock-wise like mathematicians do and unlike cuckoo clocks): Hint: Baconian by shape

2. Preparing

To get the correct order, the butterflies have to be ordered: Starting with the grey butterfly in the middle the next butterfly-generation has exactly one property changed: either the shape (wings or antennae) or the color or pattern. With this rule a family-tree can be created like this (based on Alconja's work)

3. Solving

With the hint on Bacon's cipher the solution is hidden as a binary code with A=00000, B=00001, etc. In this riddle a butterfly is considered as 1 if its shape is different (wing-shape or antennae) and is considered as 0 if the shape is the same (and the color or pattern is different). Starting on the second image, the first branch becomes
000110000010001101100100001101
split into chunks of 5 yields
00011 = D, 00000 = A, 10001 = R, 10110 = W, 01000 = I, 01101= N.
Each new branch of the butterflies forms a new word, resulting in the solution: Darwin was right no creationism

A big thank you for this beautiful and challenging riddle! And of course to Alconja and JTL who did all the hard work. I'm new here, so if I can split the bounty, I'm happy to do so.

Answer 2

Partial answer

I mentioned in the comments above that I'd made some partial progress on this. I haven't had much time to take it any further, so I thought I'd post my work so far in case it helps others...

Firstly, I noticed that:

The butterflies are all unique, and that, more importantly, they follow a evolutionary progression. Starting with the completely plain butterfly in the centre and then making a single change each generation, you can map out a family tree of sorts.

Following this logic, I produced:

A labelled copy of the original image.

full size (1.5MB)

Numbers represent the generation (starting with 0), letters show where the species diverged into distinct parallel descendent branches (i.e. A18 & B18 both evolved from 17, and C43 & D43 both evolved from C42).

For reference, the leaf nodes (as shown in circles above), are:

So visualising that result another way we get:

The family tree.

full size (1.4MB)

The red numbers down the left are the generation, with the columns matching up with the various branches. The blue numbers are the total count of individual butterflies up to that point.

That's about all the concrete output I have, but I have a few ideas of what it could mean...

Theories and observations:

The path created by tracing the family tree through the original image seems pretty random, so I don't think it's forming shapes or anything of that sort. Therefore, I assume the answer must be derived from the features of the butterflies themselves.

We are told the answer is a five word phrase, so I'm guessing it will be something along the lines of "Out of chaos comes order", or "Stop and smell the roses", or "Life, uh... finds a way". There also happens to be exactly five branches to the family tree, so I'm guessing each branch (or each leaf node) represents a separate word.

The hint mentions counting, so I imagine we can find letters for each word by counting either changes in features, or the number of generations each feature survives for, or something similar, along each branch. That being said, no feature lasts particularly long in any given family branch, so there may be more to it (or the letters would all necessarily be early in the alphabet).
The tags on the puzzle include "cryptogram", so it's plausible that there's some decryption necessary beyond just mapping A=1, B=2... Baconian encryption came to mind due to the binary nature of changes, but given that each generation changes by exactly one bit, it seems unlikely.

Answer 3

This is not an answer, but some might find it helpful.

First of all, as has recently been indicated in some comments, I noticed the following:

Hint 2:

You think the flying direction of butterflies is random? Think again!

Hint 3:

A single butterfly (in one direction). But two?

Hint 4:

Have you taken a close look at hint 3 above?

Which seems to suggest that

The frequency of the directions might encode letters.

Here's a demonstration:

I did this with some drag'n'dropping:

Unfortunately, I think I waited too long to post this, so it may not help @Alconja much since it seems he's figured this out already. Having said that, I noticed that,

assuming the letters are correct, we have everything we need to spell some words, (most vowels, some useful consonants) though I couldn't get anything out of it. I tried some things like looking at first or last letters of butterflies with certain colors, and a few other things, as well, though I haven't put much effort into that yet, so there could still be something there.

All I really got out of it was a slight feeling of

Also, for what it's worth,

one of the first things that this kind of puzzle makes me think of is Morse code, however, in this case, I have a strong hunch that this is not relevant this time, even though it doesn't seem like a stretch to interpret the markings on the butterflies as dots and dashes. Some butterflies would have to encode two letters if that were the case, and there would have also been the question of direction, though I personally would have started with anteriorly to posteriorly and in a brachial direction.