DEKADOKU, the new challenge

Question

Thanks to @Beastly_Gerbil for the name. I'm sharing a new puzzle challenge with you. This is the first one I ever created, inspired by the Scientific American magazine cover from 1959. You can search for the answer online, but I will only award points if you explain the solution procedure in detail. Don’t worry, this one is easier. Good luck!

You already know the rules:

1. Fill the grid with all the combinations of two-digit numbers (from 00 to 99).
2. In each row or column, no two numbers can have the same number of tens.
3. In each row or column, no two numbers can have the same number of units.

Text version:

   15    30    03    96    44
63    16    02    98    55
   23    06    94    11    57
46    24    95    33    17
   86    91    22    37    19
14    81    66    27    39
   54    88    67    29    76
97    00    51    84    26
   90    64    36    53    85
70    99    34    56    83

Answer 1

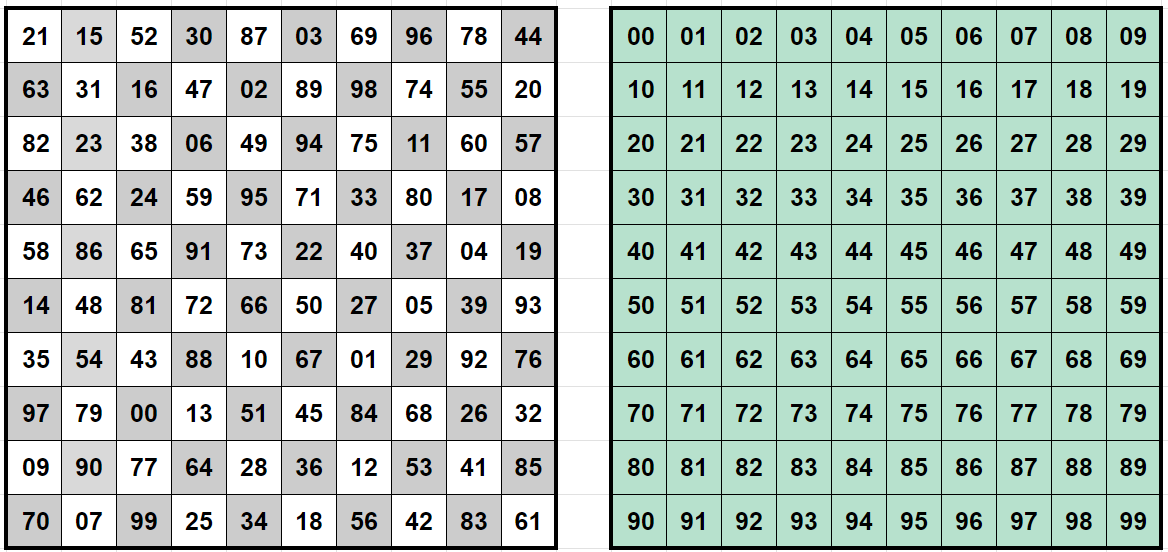
Another great puzzle, still quite tricky and I made quite a few mistakes that I had to backtrack over several times!

Here's the solution:

---

Setting up the tracking grid to automatically keep track of the numbers and alert if any appear twice (same formulas as from here)

2:

Note there are only two 9-s left, 92 and 93. These must appear in the last 2 columns, and in the 6th and 7th rows. The 9th column contains a -3, so this column must contain the 92,and both numbers can be placed.

We can also do the same for the -4s, appearing in the 8th and 9th columns, and the 2nd and 5th rows. The 2nd row contains 02, so this must also contain the 74, with 04 in the 5th row.

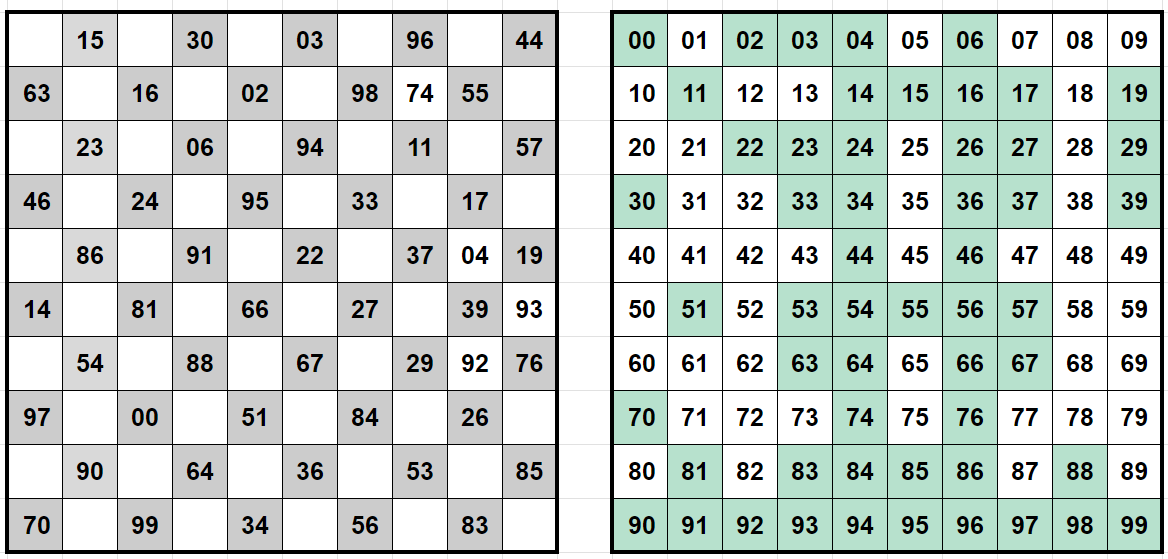
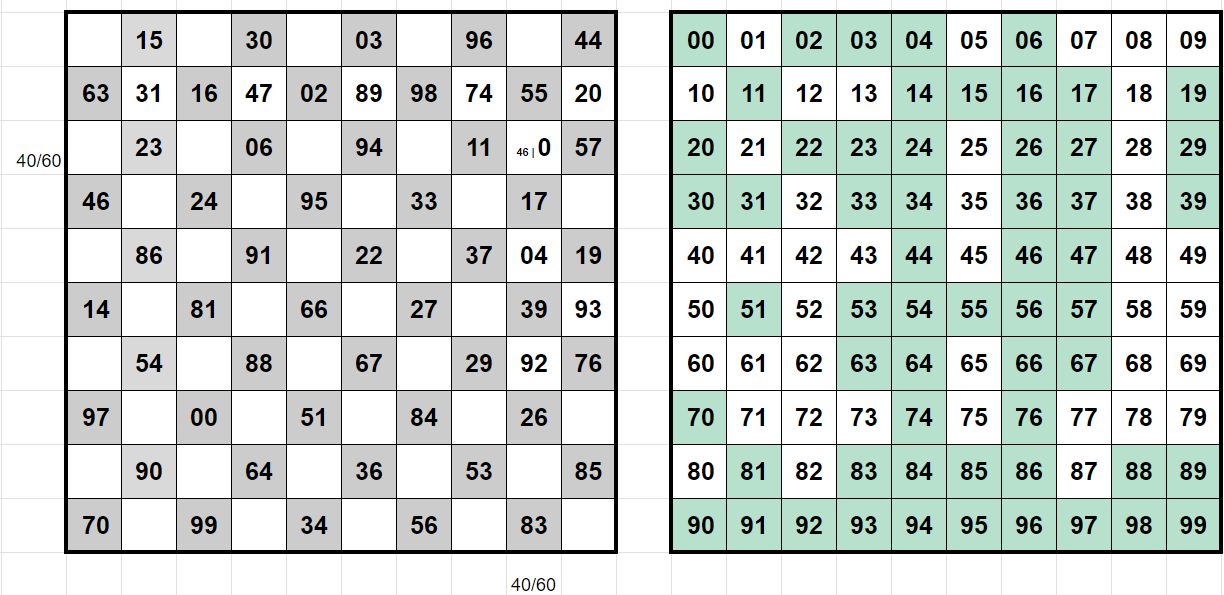
3:

Lets look at the 9th column as there are only 3 empty cells. -0 must be in the 3rd row, so this is either 40 or 60. However nothing else can be gleaned here.

So instead lets look at the second row. There is only one place where 8- can go, in the 6th cell, which must be 80 or 89. The 4th cell must start with 2 or 4, and end with 7 or 9, but as 27 and 29 are both taken, it must start with 4. This means the second cell starts with a 3, and the final cell with a 2. In fact the second cell can only be 31, which makes the final cell 20, and this allows us to finish off this row.

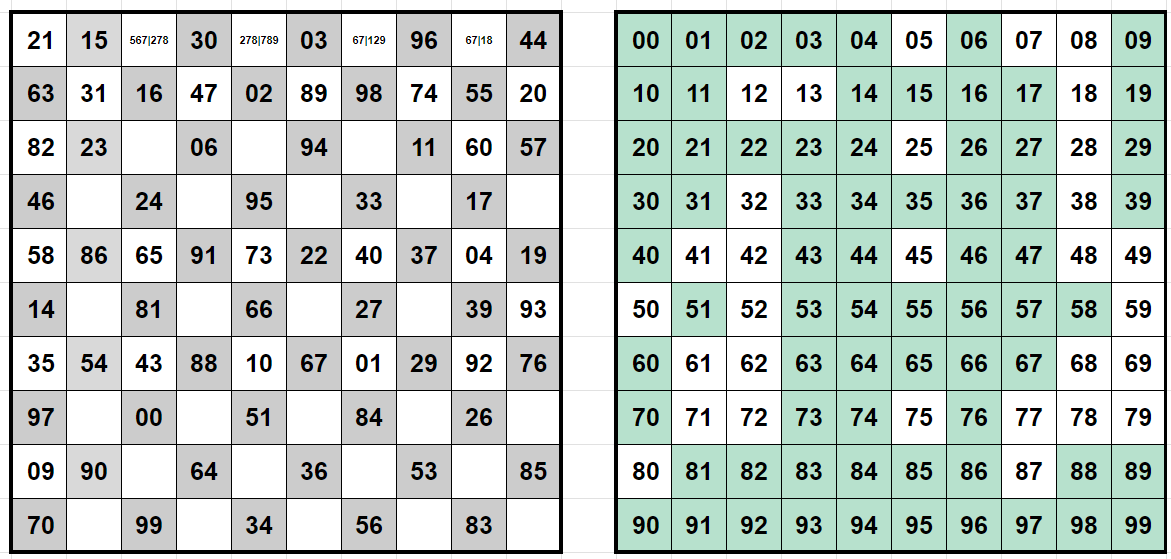
4:

In the 1st column, the fifth cell must be 58, whilst the 7th must be 35. The 3rd cell must be 82, and the first column can be resolved.

In the 7th row, the 3rd cell must be 43, and the rest of the row can be filled with 10 and 01.

This means in the 5th row, the 5th cell is 73, which resolves the 5th row.

The 5th row also contains 40, which resolves the 40/60 from earlier.

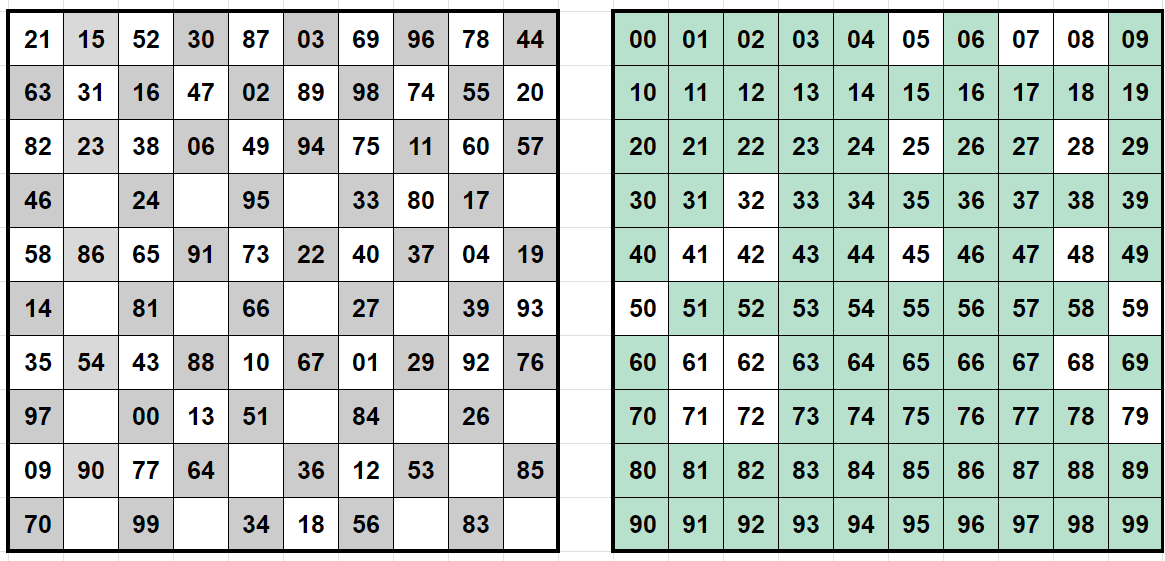
5:

Lets start looking at the tracking board now. There is only one -3 left, 13 so this can be placed and it is in the 4th column.

There are 2 1-s left, in the bottom two rows, and these can also be placed.

The final 8-s can be placed in the top and 4th rows, and this also places a 52 in the top row.

The 3rd column can now be completed with 38 and 77, which also allows the 3rd row to be completed.

The 60 placed earlier also resolves the top row.

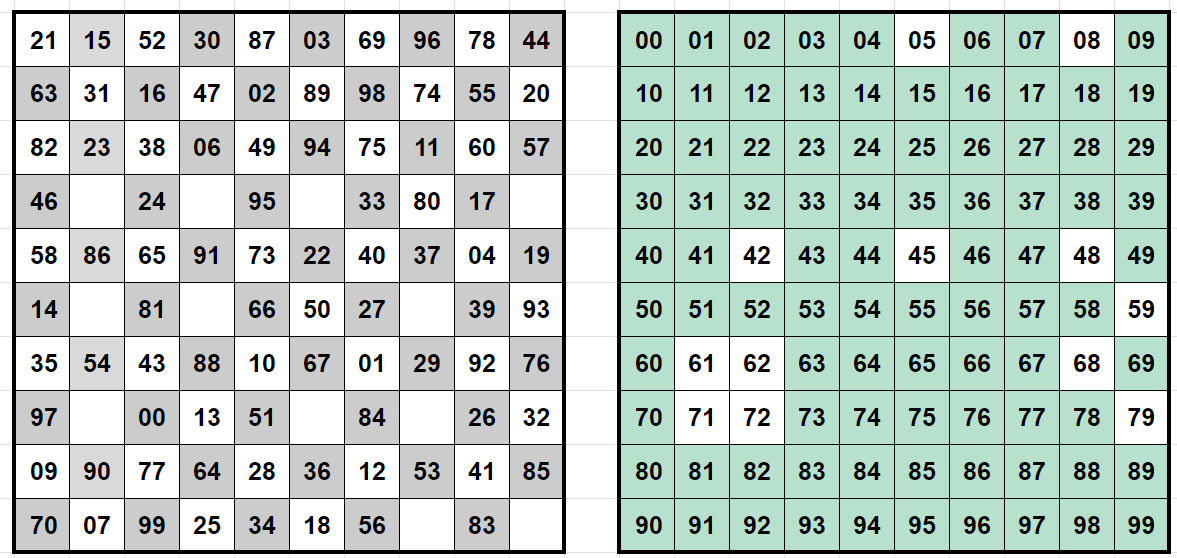
6:

The last -0 can be placed in row 6, as can the last 3- in the final column. The 9th column can then be solved, then the 9th row.

This leaves one 2-, 25, which can be placed in the final row. The final -7 can also be placed in the bottom row.

7:

Finally, the final 5- can be placed in the 4th row, followed by the final -9, in the second column.

Now to finish off, the 4th column solves, followed by the bottom row, then the final column.

The final -1 and -2s can be placed, leaving just 4 numbers. These quickly are placed to give he final solution:

Answer 2

I just want you to see the solution visually. On the cover of Scientific American, the colors represent the digits for the tens and units. The outer color corresponds to the tens, and the inner color to the units. In this pattern, 0 is black, 1 is yellow, 2 is blue, and so on. The perfection of this design is truly beautiful.