-1
$\begingroup$

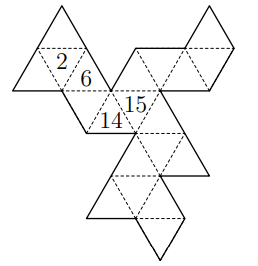
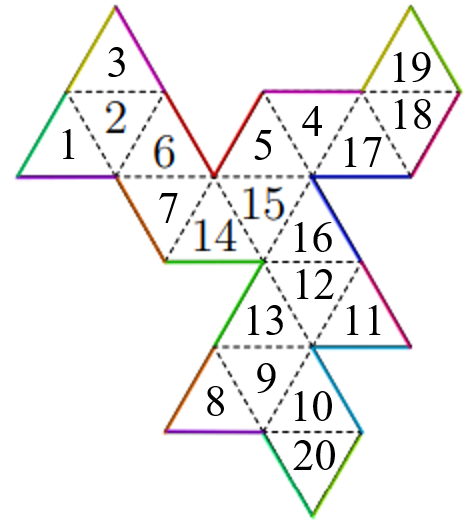
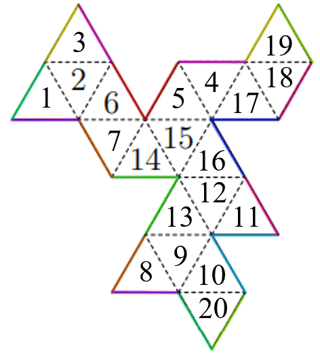The net of $20$ triangles shown to the right can be folded to form a regular icosahedron. Inside each of the triangular faces, write a number from $1$ to $20$ with each number used exactly once. Any pair of numbers that are consecutive must be written on faces sharing an edge in the folded icosahedron, and additionally, $1$ and $20$ must also be on faces sharing an edge.

enter image description here

Improve this question
$\endgroup$
1
  • 5
    $\begingroup$ If you wish to post questions from elsewhere that are not your own original creations, you MUST provide attribution to the original. This is a post from USAMTS Round 2, back in 2014-15. It is site policy here that users must make sure the original source is declared - please make sure you do this in your posts. $\endgroup$
    – Stiv
    Commented Jan 5 at 0:21

1 Answer 1

Reset to default
6
$\begingroup$

Solution (with touching edges highlighted):

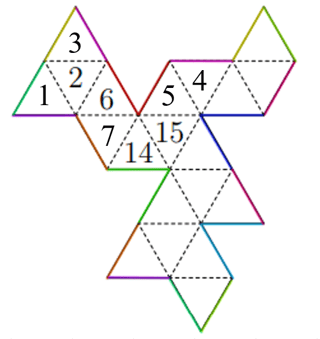
enter image description here

How to solve:

Firstly, lets label the edges to show what connects to what:

enter image description here

Now we can start to plan a route. We know the 5 must connect to the 6 so has to go right or down, but also has to be able to trace back to the 2. The only way this can be achieved is through the purple edge, and this means numbers 1 through 7 can be placed:

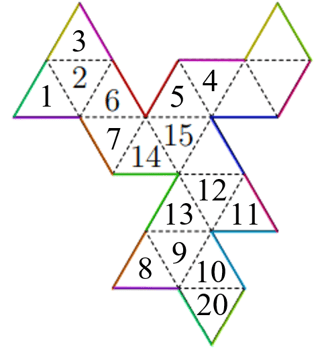
enter image description here

We now have the task of connecting the 7 to the 14. The 7 has to go through the orange edge to 8, but as 1 connects to 20 and the 8 cuts of one of the two edges to the 1 off, the 20 must go bottom right and connect through the turquoise edge.

The 14 can only connect to 13 via the green edge, and the 9 and 10 both have to go right from the 8. There is only then one way to circle round to the 13:

enter image description here

Finally, 16 to 19 have only one path that they can go to reach the the light green edge top right to connect back to the 20, and we have the answer:

enter image description here

Improve this answer
$\endgroup$
3
  • $\begingroup$ Solving in 40 minutes including the presentation is amazing. $\endgroup$
    – Weather Vane
    Commented Jan 4 at 22:05
  • $\begingroup$ @WeatherVane honestly the logic for placing the numbers was quite simple, it was just trying to wrap my head around how the net folded and which edge touched which that was the hard part! $\endgroup$
    – Beastly Gerbil
    Commented Jan 4 at 22:08
  • $\begingroup$ The edge connections are good. I found the easiest way was to quickly cut a rough model and fold the edges. $\endgroup$
    – Weather Vane
    Commented Jan 4 at 22:30

Not the answer you're looking for? Browse other questions tagged or ask your own question.