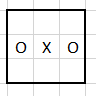
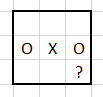It is my own creation. It is not part of a test, so there is no ABCD choice. You must figure out the underlying rule to find the missing pattern.
Before you ask: nothing to do with noughts and crosses (aka tic-tac-toe).
Hint:
The key is in the title.
Don't try to find a row rule or column rule.
Hint:
Hint:
It is marked as "progressive-matrix" because it is the closest. But I don't think "progressive" is the correct term. There is no pregression between the squares.
Also, instead of "holographic" I should better say "auto-holographic".
It should be called an auto-holographic matrix puzzle.
Hint: