__________ is the way to go!

Question

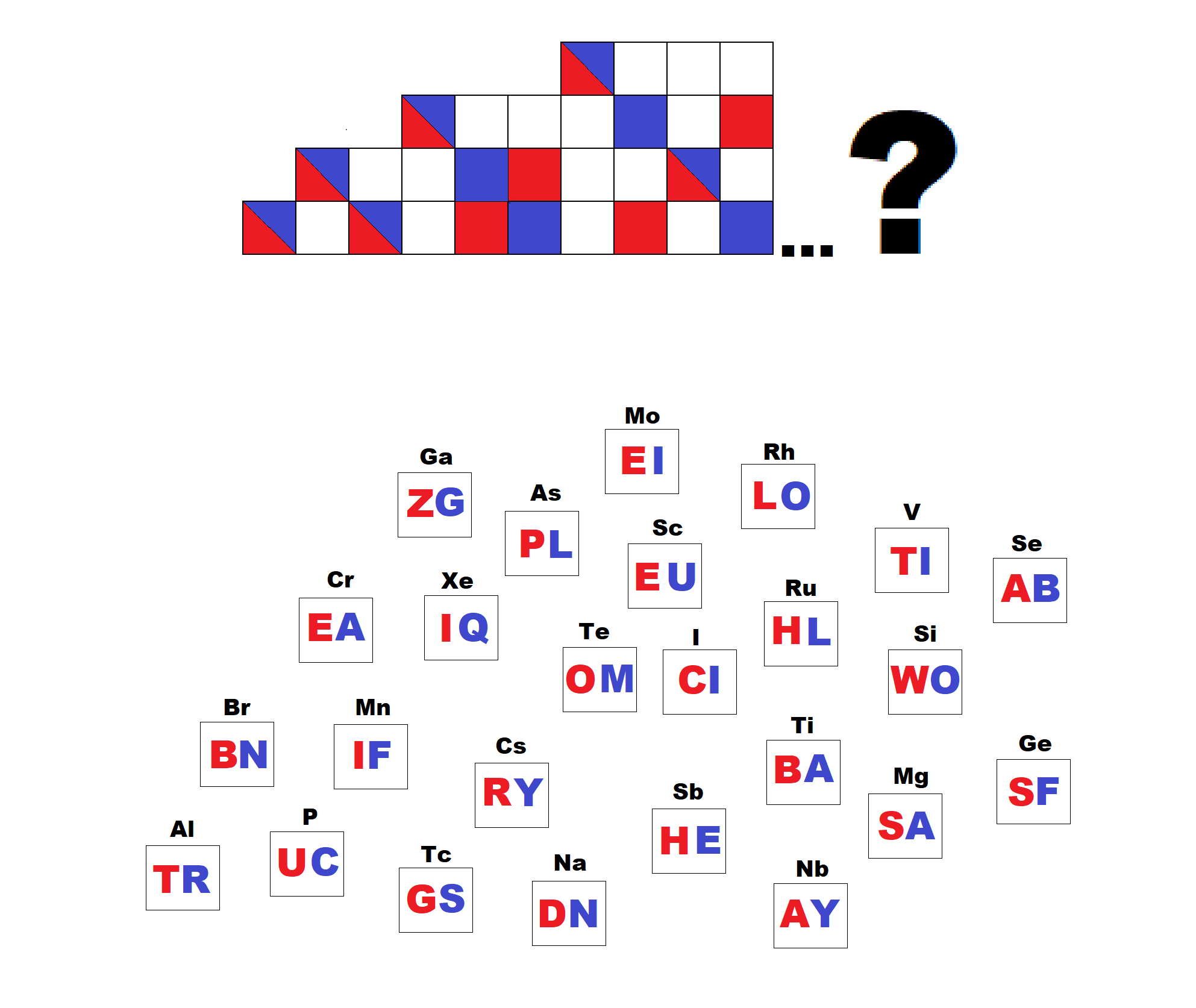
I'm looking for a 10-letter word.

---

---

Answer 1

Solution:

DIAGONALLY is the way to go.

Step 1:

I first looked at the unordered bunch of element names and letters in the bottom. The periodic table of elements returned these 25 numbers: 11, 12, 13, 14, 15, 21, 22, 23, 24, 25, 31, 32, 33, 34, 35, 41, 42, 43, 44, 45, 51, 52, 53, 54, 55. It seemed to me that these were equivalent to coordinates on a 5x5 grid, e.g. the element Na (11) would correspond to the top left corner (1,1), and so on.

Step 2:

The graphical pattern shows a row of regularly growing squares (1x1, 2x2, 3x3, 4x4). I assumed that the top left corner always stays, while the other section expands regularly with red and blue squares in the diagonal axis. Then, the next square (5x5), would look like this:

X . . . .
. B . . R
. . B R .
. . R B .
. R . . B

(X = red/blue, B = blue, R = red)

Step 3:

I laid the letters of the corresponding elements on the grid and took only the letters of the colored squares. I assumed that a red square returns only the red letter, a blue square only the blue one and a red/blue square both letters. This returned these letters:

DN .. .. .. ..
.. .A .. .. O.
.. .. .L G. ..
.. .. A. .L ..
.. I. .. .. .Y

Step 4:

... which can be reordered to DIAGONALLY.