10
$\begingroup$

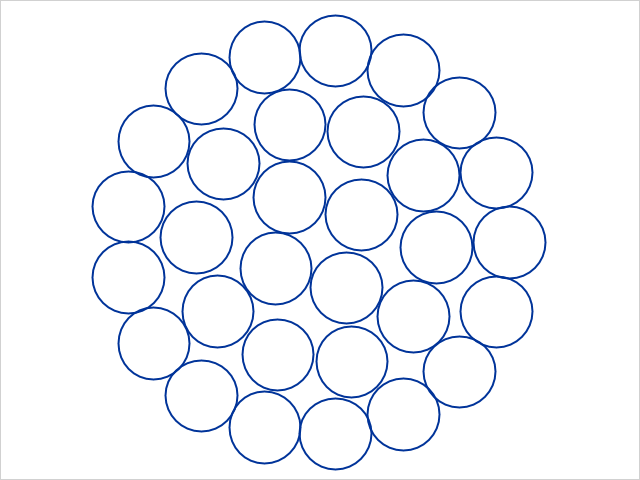
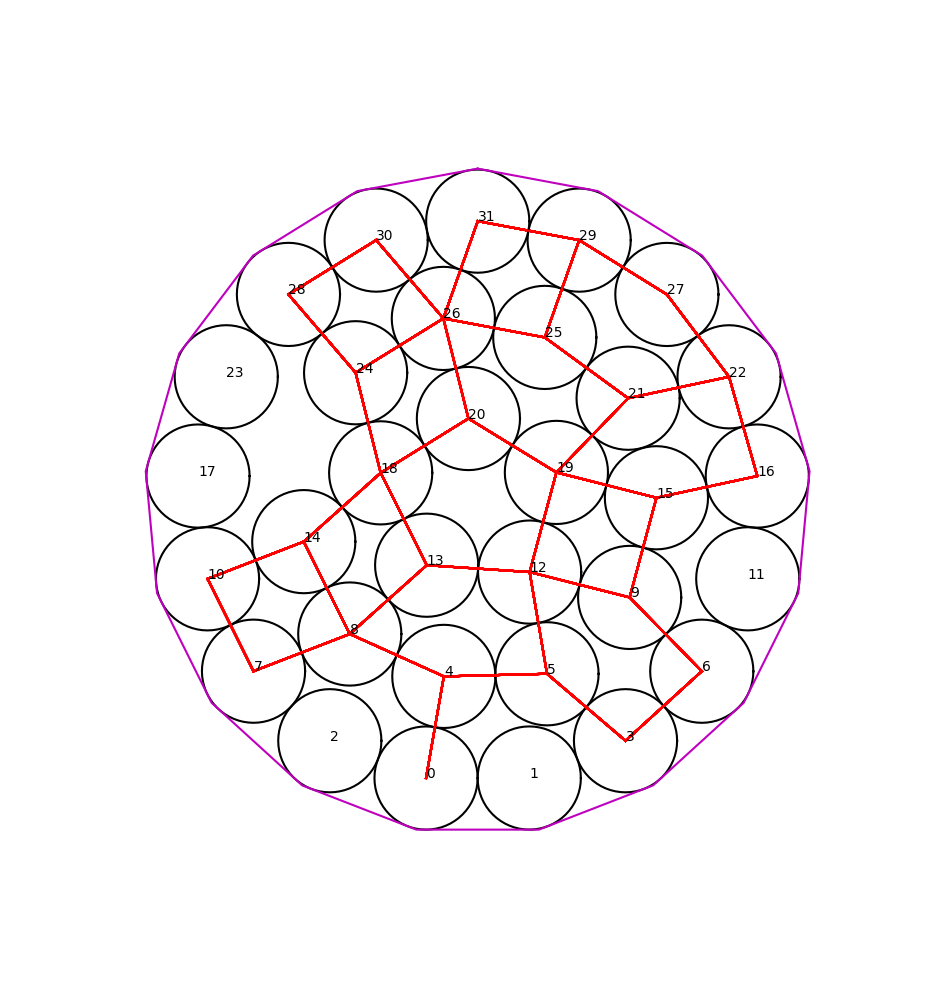
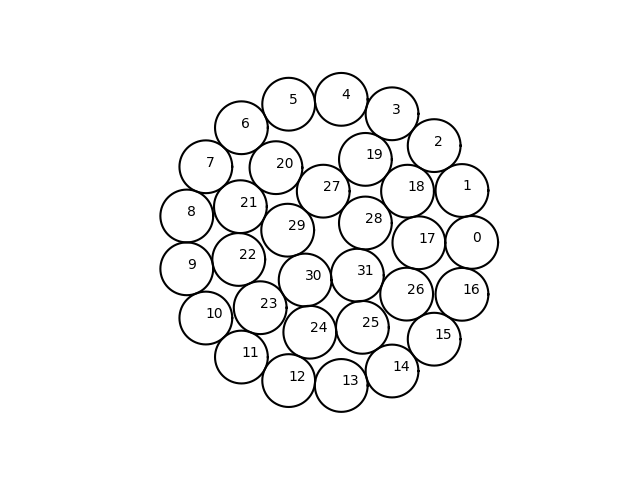
17 identical coins with diameter 1 are lying flat on a table, such that their midpoints build the vertices of a regular 17-gon (regular heptadecagon) and adjacent coins touch each other.

What is the maximum number of coins, which can be added inside the 17-gon, none of them overlap and lying flat on the table?

Note: the coins to add are of the same size as the coins building the 17-gon.

Improve this question
$\endgroup$
2
  • 2
    $\begingroup$ If it's the pool table I used to play on at a dive by the beach, then fifty easy. That thing was all kind of warped $\endgroup$
    – Dr Xorile
    Commented Apr 16, 2021 at 15:58
  • 2
    $\begingroup$ Are you, by any chance, in the process of making pepperoni pizza? :-) $\endgroup$
    – Bass
    Commented Apr 16, 2021 at 20:23

2 Answers 2

Reset to default
6
$\begingroup$

I'll get things started with

14

enter image description here

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ I got the same, filling concentric rings of width 1. It likely cannot be improved by 2 coins, assuming this best-known 33-coin packing is indeed optimal, but it is hard to tell whether maybe one extra coin can be squeezed in. $\endgroup$
    – Jaap Scherphuis
    Commented Apr 17, 2021 at 6:07
  • $\begingroup$ loopy walt pointed out a way to prove that it is optimal at the current state of research. $\endgroup$
    – justhalf
    Commented Apr 22, 2021 at 6:03
5
$\begingroup$

UPDATE: @Rob Pratt's is optimal at current state of research:

Here is the best known packing of 32 circles into a regular heptadekagon.
enter image description here
Coordinates taken from http://hydra.nat.uni-magdeburg.de/packing/ced/ced.html If we could pack 15 circles into a regular 17-ring we would beat that. Therefore if we believe the experts it is not possible.

Mind you, it's pretty darn close. All the red lines indicate touches. Those which are not marked but look as if they ought to be are less than 6 permille (1/10), 3 permille (1/10) or less than 1 permille (8/10) of a radius apart.

original post:

Tada!

15: enter image description here

No, sorry, I actually cheated.

There is a tiny amount of overlap between 30,31 30,24 and 31,25.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Interesting. This (your cheating solution) is on 17-coins ring, and it's so close to a solution, yet by adding one more coin in the circumference it still can't fit? (because otherwise we would have 33-coin config for 18-gon) $\endgroup$
    – justhalf
    Commented Apr 23, 2021 at 5:40
  • $\begingroup$ @justhalf I don't understand. One can actually relatively easily fit 16 inside an 18-ring. $\endgroup$
    – loopy walt
    Commented Apr 23, 2021 at 6:53
  • $\begingroup$ Oh, I misread your answer. I thought the line of thought was "with N-gon, we can fit M coins inside, totaling N+M coins. If we can fit M+1 in N-1-gon, we can simply add one coin to the outer ring to get M+1 coins inside N-gon, totaling N+M+1, beating the optimal, which is "impossible"". So why is Rob's answer with 14 coins inside 17-gon not able to be improved to 15 coins inside? The total is still 32, which is the optimal one. $\endgroup$
    – justhalf
    Commented Apr 23, 2021 at 7:12
  • $\begingroup$ @justhalf the subtlety is that fitting 15 in a 17-ring is harder than fitting 32 in a circle that can just hold the 17-ring because placing 17 as a ring is not optimal for the task of putting 32 no matter how. In fact, 32 can be packed in a circle that is actually too small to hold a 17-ring, so we do not get a bound from that. The 17-gon is in between easier than the ring but harder than the circle. And it happens to yield a bound for the ring question. $\endgroup$
    – loopy walt
    Commented Apr 23, 2021 at 7:21
  • $\begingroup$ Ah, ok. That's the part I'm missing. Thanks for the explanation! It's a bit complex then to say that we can't fit 15 into 17-gon. Maybe you can elaborate more on the image caption? $\endgroup$
    – justhalf
    Commented Apr 23, 2021 at 7:22

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.