Teachers around a circular table - who teaches geometry?

Question

The puzzle is as follows:

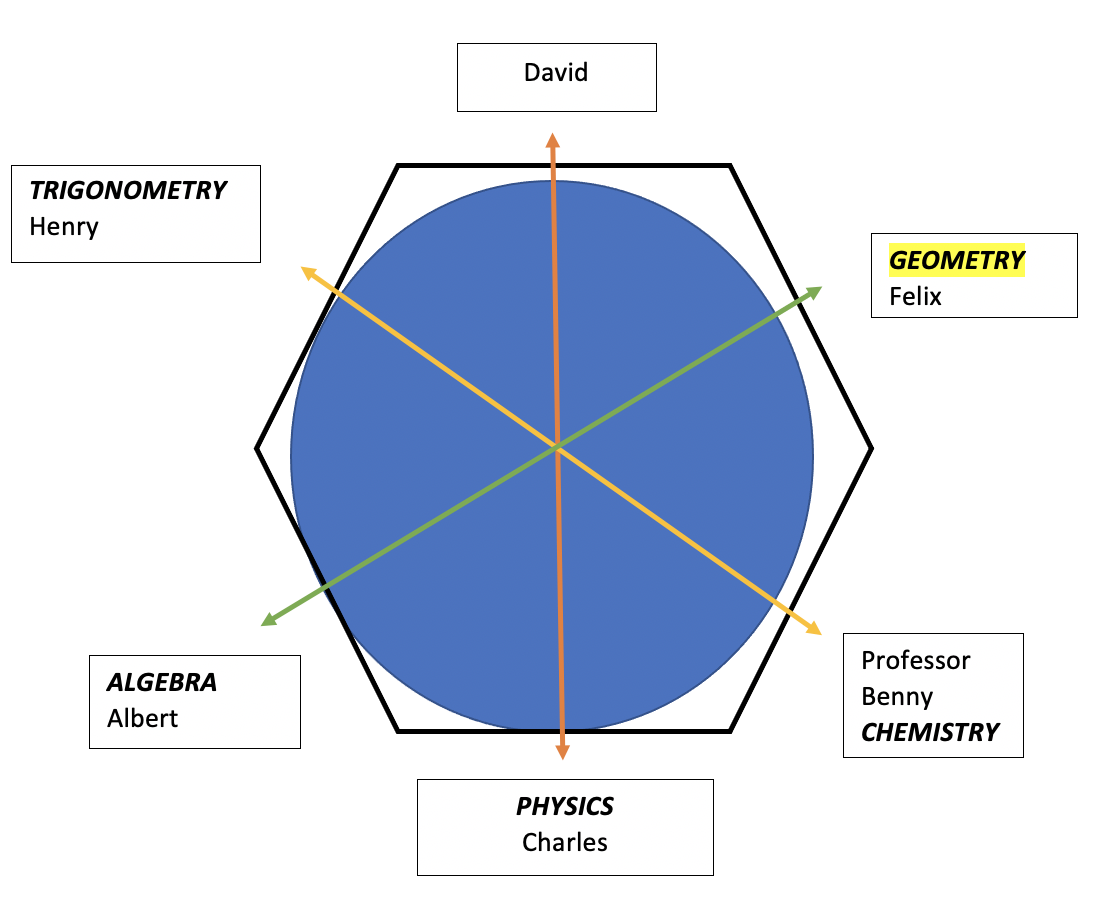
In a teacher's lounge there is a circular table where the teachers eat their meals during lunch time. The teachers' names are Albert, Benny, Charles, David, Henry and Felix, and they are teachers of algebra, arithmetics, geometry, trigonometry, physics and chemistry. The seats are positioned symmetrically around the table.

The physics teacher, who had differences with Albert, sat in front of David.

The algebra teacher sat between the trigonometry and physics teachers.

Henry sat next to, and to the right of, the algebra teacher.

Charles is sitting to the right of the chemistry teacher.

The algebra teacher sat in front from Felix and to the left of the physics teacher.

Professor Benny loves numerical calculations, and he is in front of the professor of trigonometry.

Given these clues, who is the geometry teacher?

The choices given in my book are as follows:

1. Felix
2. Albert
3. Charles
4. Henry

I found this puzzle in my book Reason and Logic from 2000s. It belongs to the topic of Ordering information. From the style it seems adapted from a reprinted copy of Martin Gardner's 70's book on Puzzle Carnival.

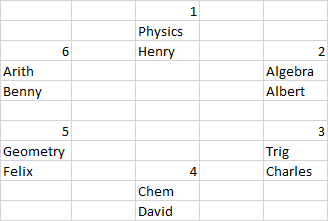
This sketch shows what I have tried so far:

However I'm stuck with because I don't know what sat in front of means. I think that they are equally distributed, so seats are arranged around the table like an hexagon (hence the hexagon in a lighter shade). This would mean that the phrase sat in front of could mean the directions indicated by the arrows. However, I'm not sure if this is the correct interpretation. I'm stuck with the Algebra teacher, because it seems he's used as a pivot to arrange the rest surrounding him. There seems to be a contradiction when I reach the part which mentions the chemistry teacher, which causes me to put David in another place.

I think that the occupations of each teacher around the table is not the same order as the given list, and that using the clues I must find which belongs to each person. But this part is confusing.

I'm not good with ordering or spotting places in a distribution relying on imagination. Therefore, all answers must include a drawing and a step by step approach explaining the meaning of each clue and how should be interpreted. I'm really lost on this one. Also, are my initial findings in the right track?

Answer 1

I drew the circle and drew a hexagon around it as you did. Instead of placing the teachers at each corner of the hexagon, I put them at each face.

Clue 1: "The physics teacher, who had differences with Albert, sat in front of David."

• This gives us two places on the hexagon. The physics teacher and David are at faces opposite each other.

Clue 2: "The algebra teacher sat between the trigonometry and physics teachers."

-Since the first clue gave us the location of the physics teacher and we know that the algebra teacher is right next to him, that means the algebra teacher is at either the left or right adjacent face to the physics teacher.

Let's skip to clue 5.

Clue 5: "The algebra teacher sat in front of Felix and to the left of the physics teacher."

-This verifies the location of the algebra teacher, he must be on the left adjacent face to the physics teacher.

-This also gives us Felix's location, he is on the face opposite to the algebra teacher.

-Clue 2 says that the algebra teacher is "between the trigonometry and physics teachers". Since the physics teacher is on the adjacent face to his right, then the trigonometry teacher is on the adjacent face to his left.

Clue 3: "Henry sat next to, and to the right of, the algebra teacher."

-We've just discovered that the teacher sitting to the right of the algebra teacher is the trigonometry teacher. Therefore, Henry is the trigonometry teacher and cannot be the answer.

Let's skip to clue 6.

Clue 6: "Professor Benny...is in front of the professor of trigonometry."

-Professor Benny is on the face opposite to the trigonometry teacher. This face is also the right adjacent face to the physics teacher.

Let's go back to clue 4.

Clue 4: "Charles is sitting to the right of the chemistry teacher."

• The only subjects yet to be located are chemistry, arithmetic, and geometry. We know that Charles cannot be the chemistry teacher. He also cannot be the trigonometry teacher (Henry). The teacher to the right of Henry is David, the teacher to the right of David is Felix, the teacher to the right of Felix is Professor Benny (whose subject is unknown) and the teacher to the right of Professor Benny is the physics teacher (whose identity is unknown). Therefore, by process of elimination, the chemistry teacher must be Professor Benny and the physics teacher must be Charles.

• This means that Charles cannot be the geometry teacher.

---

What we know so far:

Henry = Trigonometry Professor Benny = Chemistry Charles = Physics Albert = Unknown David = Unknown Felix = Unknown

-Based on David's location in front of the physics teacher (Charles), we know that he cannot be the algebra teacher who sits to the left of Charles. That means that the algebra teacher is either Felix or Albert. -Felix cannot be the algebra teacher because clue 5 states that the algebra teacher sits in front of Felix.

-Therefore,

Albert = Algebra Felix = Unknown David = Unknown

Nothing is stated about the arithmetic teacher nor the geometry teacher. Based on the choices provided:

Felix = Geometry Teacher

Answer 2

Here is the layout which I came up with.

In my approach I've taken in front of to be directly opposite the person and left and right to be next to the person.

1. The physics teacher, who had differences with Albert, sat in front of David.

Physics at position 1

David at position 4

5. The algebra teacher sat in front from Felix and to the left of the physics teacher.

Algebra at Position 2

Felix at Position 5

3. Henry sat next to, and to the right of, the algebra teacher.

Henry at position 1

2. The algebra teacher sat between the trigonometry and physics teachers.

Trigonometry at position 3

6. Professor Benny loves numerical calculations, and he is in front of the professor of trigonometry.

Benny at position 6

Arithmetic at position 6

4. Charles is sitting to the right of the chemistry teacher.

Charles at position 3

Chemistry at position 4

The table now has 1 position without a name and 1 position without a subject. Albert and Geometry are the unused names and subject.

Albert at position 2

Geometry at position 5

This table layout seems to satisfy all the clues, but there is one thing which makes me uncertain about my solution, and that is part of clue 1. The physics teacher, who had differences with Albert. My solution has these two people sitting next to each other. I'm not certain if the clue is to be intepreted they Albert is not the physics teacher, or if they do no sit next to each other.