5
$\begingroup$

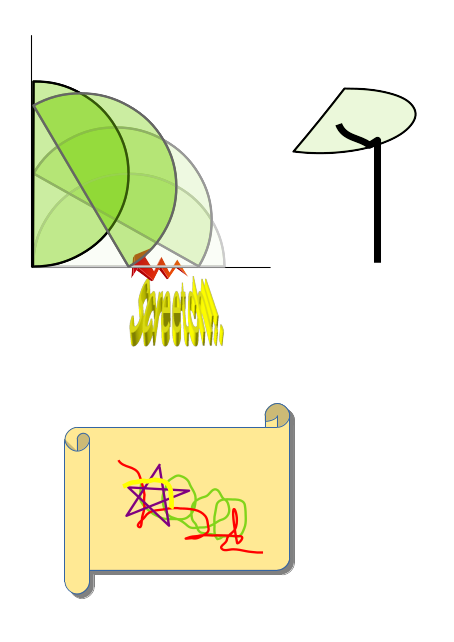
That didn't go to plan. You just wanted to help your friend the artist redecorate. In the process you mananged to make an ugly notch in their favorite table, scratch their wall when moving said table round a corner and as only now you notice to knock over a jar of ink sat on the same table which as if to mock you has found its way over the table's surface to the notch and down through the notch in a neat focused beam onto some concepts your friend had been careless enough to let lie on the floor.

Your friend is a person of superhuman patience and will let you get away with murder but spoiling their sketches is another matter. You my friend are in hot water. Luckily for you I happen to have a magic eraser on me that can remove any kind of ink. It will remember the first color you touch and then continue to remove all ink of the same color but none of any other color. Magic, wouldn't you agree?

What? You are kidding me! You have forgotten which color? And no ink left in the jar or on the table? Well, I don't care. I'm a geenie, not a charity. Here is your magic eraser, please sign the receipt here, and good day to you. One final word of advice, use your brain, it can't possibly be worse than your hands!

enter image description here

Will you be invited to your friends next opening event? It's in your hands---which, all things considered, doesn't bode too well...

Problem was given to me at a party (yes, that kind of party). Exact source untrackdownable I'm afraid.

Clarifications:

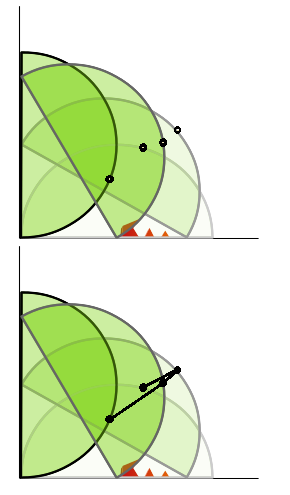
The stacking of colors (which line crosses over which other) on that doodle, I mean concept art, is not a clue. It should be obvious from the overall quality of the pictures that such attention to detail is not at my diposal. That said, there is information in that picture.

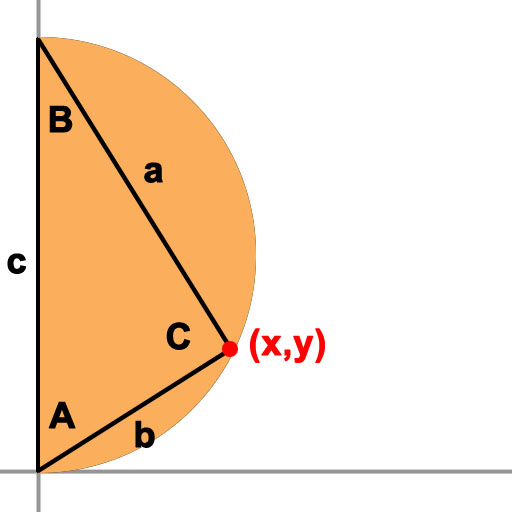
Generally: Read the pictures as schematics. They contain information but whatever fine detail subtleties you may find are unlikely to be intentional simply because I wouldn't be capable.

Hint:

As the best approaches so far have come from non mathsy people: If you are one of those and nonetheless want to have a shot:

you only need a single theorem, possibly multiple times: https://en.wikipedia.org/wiki/Inscribed_angle

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ I can't tell what the top picture is. Is that part of the puzzle or just my being dense? $\endgroup$
    – msh210
    Commented Aug 25, 2020 at 19:15
  • 1
    $\begingroup$ @msh210 Nope, my pathetic drawing skills are not part of the puzzle. The left bit is a top view of the table being pushed round the inside of a corner. The right bit is an impression of ink running over the table top to the edge and then down to the unseen floor. $\endgroup$
    – Paul Panzer
    Commented Aug 25, 2020 at 19:19

6 Answers 6

Reset to default
3
$\begingroup$

Ok, I think I figured out how to solve the problem without using trigonometric formulas!

enter image description here

Basically we have to show that for any rotation W, the angle X is always the same.

FlorianF's comment got me thinking and I believe the trick is that:

the origin o belongs to the same circumference as p, q and r.

The reason is that:

o and p subtend the same angle (90 deg) for the same chord (the diameter).

Knowing that and using the same principle again we get:

o and r belong to the same circumference and share the chord pq, which means they subtend the same angle. Therefore X = B for any W and point p moves on a straight line, which means the stroke must be purple.

Improve this answer
$\endgroup$
1
  • $\begingroup$ That's an interesting idea of "not using math"! Anyway, that is the solution I had in mind, so congratz! Btw. the non-math you have been using is called the Inscribed Angle Theorem. $\endgroup$
    – Paul Panzer
    Commented Sep 2, 2020 at 13:03
5
$\begingroup$

My answer:

The stroke is one of the purple lines.

Because:

Displacing the table like that makes the ink jar move on a straight line.

The reasoning is as follows:

We want to track the position of the point (x,y) as the table rotates. We have x = a sin(B); We have y = b sin(90-A); The fact that the table is a semicircle means C is a right angle. Therefore B = 90 - A; Therefore y = b sin(B); As we rotate the table by an angle w we have y/x = b sin(B+w) / (a sin(B+w)) = constant. This means the point (x,y) moves on a line that crosses the origin.

inscribed triangle

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ This graph demonstrates visually the movement =) desmos.com/calculator/evjqdkt5ch $\endgroup$
    – David Lewis
    Commented Aug 29, 2020 at 6:57
  • $\begingroup$ While the math bit is not the most elegant way of doing it it is correct and complete. Well done! $\endgroup$
    – Paul Panzer
    Commented Aug 30, 2020 at 17:36
4
$\begingroup$

We will suppose that the ink knocked on the art project wasn't previously used in said art project.

I think the answer should be:

The yellow arc

Reason:

your table undergo 2 transformations at the same time: a translation and a 90° rotation as shown bellow; note that for more clarity I separated the transformation in 2 steps when those are concomitant: enter image description here As such the shape to be erased can't have multiple pointy areas as the purple shape. And as the rotation is only a quarter of a circle you can't have multiple arcs like in the red and green shapes. Only the yellow shape remain possible.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Overall approach is good, but: Re 1: I had hoped to have clarified that. Re 2: It uses one assumption that is not backed by the story. $\endgroup$
    – Paul Panzer
    Commented Aug 26, 2020 at 11:09
  • $\begingroup$ @PaulPanzer edited point 1, for point 2 I use the top left picture which shows the movement of the table. I clarified what I meant $\endgroup$
    – Sechiro
    Commented Aug 26, 2020 at 12:07
4
$\begingroup$

My final answer

The purple star

Because

Building on my previous answer, the way the table is moving causes the drip point to move on a certain path.

Below is the original image I used to place points by eye. This image is slightly off because I didn’t use exact measuring for the points.

enter image description here

However, doing the experiment at home using multiple points on the semicircle, I realized the dropping line still resembles the previous rough image at the point used, only with a sharper point and straighter lines. EDIT - And it branches the other way. (Revised picture below):

enter image description here

My theory is that after turning the table, the ink spilled midway through the turning of the table so only a small portion of the path was created. Because the entire path wouldn't form a proper star. It was then stepped on and conveniently shaped into a star shape as the carelessly placed paper was stepped on 5 times.

So the first ink erased should probably be the ink from one’s foot.

Note: While experimenting, I found that most points on the circle create paths that make an acute angle. I happen to luckily choose the optimal point with the largest acute angle. The OP says there is math involved in finding the intended explanation which I am unable to find.

Improve this answer
$\endgroup$
7
  • $\begingroup$ Good thinking! Do a bit of proper math instead of eye-balling and you're there. $\endgroup$
    – Paul Panzer
    Commented Aug 25, 2020 at 18:31
  • $\begingroup$ @PaulPanzer, Spirograph not good enough? lol. I'm pretty far removed from proper math. I don't even know where to begin. $\endgroup$
    – MacGyver88
    Commented Aug 25, 2020 at 18:38
  • $\begingroup$ Hm, computer simulation, then? Or cutout shapes? Or if you have a half-round table and a rug to spare? Because rot13 Vg'f abg gur lryybj. $\endgroup$
    – Paul Panzer
    Commented Aug 25, 2020 at 18:43
  • $\begingroup$ @PaulPanzer, rot13(Fbeel. Gung'f nyy V pna qb. V tbg zl ubcrf hc. V thrff V pna'g tb gb uvf arkg bcravat.) $\endgroup$
    – MacGyver88
    Commented Aug 25, 2020 at 18:50
  • 1
    $\begingroup$ You're getting very close. rot13 Gur sbbgjbex gurbel vf n ovg zber ireobfr guna jung V unq va zvaq, ohg vg qbrfa'g pbagenqvpg gur rivqrapr naq vg pbagnvaf gur bar pehpvny vafvtug: abguvat va gur pyhrf fnlf gung gur ragver cnggrea fgrzf sebz gung pbeare vapvqrag. V guvax lbh unir zber be yrff pbzr nf sne nf vf cbffvoyr jvgubhg qbvat gur znguf. Fb pbatengf! V'yy yrnir gur dhrfgvba bcra sbe gur gvzr orvat. Tvir fbzrobql gur punapr gb fbyir gur npghny znguf chmmyr. $\endgroup$
    – Paul Panzer
    Commented Aug 25, 2020 at 22:48
2
$\begingroup$

Perhaps

Yellow as it crosses over every other color.

Improve this answer
$\endgroup$
1
  • $\begingroup$ No, sorry, that's just my drawing ineptitude. This is a proper problem, no lateral shortcuts. I'll add a clarifier. $\endgroup$
    – Paul Panzer
    Commented Aug 25, 2020 at 17:57
2
$\begingroup$

The color is

None of the visible colors.

Because

The path of any point of the round border of the table is a straight line.

The "center" of the table moves around a perfect circle of the same radius as the table. The border point turns around the center in a circle of the same radius. The center turns clockwise, the border point turns counter-clockwise at the same rate. The result is that the two rotations compensate and you end up with the border point moving on a straight line passing thru the wall corner.

If you assume the ink spread around the ideal path, because of wind or what, then red or green would be acceptable. Or yellow for that matter. If you assume the paper was moved repeatedly, then purple would be the choice because it is made of straight lines.

If the ink fell perfectly vertically I can only conclude that ink was light yellow as the background, or transparent.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Mathwise your answer is the best so far, only you should add an argument why the table center moves on a circle. The overall conclusion I'd say is not warranted rot13 orpnhfr gurer vf ab ernfba gb oryvrir gur vax fgnegf naq fgbcf fcvyyvat gur zbzragf gur gnoyr ragref naq yrnirf gur pbeare. Gur ernfbavat V rkcrpgrq ohg qvq abg trg sebz nalobql jnf gur zbfg yvxryl pbybe fubhyq pbagnva n fgenvtug yvar. Fvapr guvf jnf pyrneyl cbbe ceboyrz qrfvta V'z pbafvqrevat gb bayl tb ol gur znguff cneg jura njneqvat gur pbirgrq gvpx. $\endgroup$
    – Paul Panzer
    Commented Aug 30, 2020 at 17:35
  • $\begingroup$ I think Kikos' answer gives a more formal proof that the path must be a straight line. Mine is a bit intuitive. (And as a matter of principle I don't read rot13). $\endgroup$
    – Florian F
    Commented Aug 30, 2020 at 19:40

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.