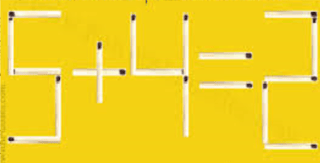
Make a correct equation by just moving one matchstick!
4
-
2$\begingroup$ You know the source reveal the answer, right? $\endgroup$– Voldemort's WrathCommented Aug 11, 2020 at 22:51
-
2$\begingroup$ @Voldemort'sWrath I didn't pay attention to it. I mean I didn't know. But I think that's the cheater's problem to look at it; not mine or non-cheaters. $\endgroup$– user70797Commented Aug 12, 2020 at 8:33
-
$\begingroup$ @aminabuzz -- I mean, if you're revealing the answer in the question, it's not a great question, is it? $\endgroup$– Voldemort's WrathCommented Aug 12, 2020 at 13:26
-
$\begingroup$ @Voldemort'sWrath It's better to provide a link if the question isn't your own creation $\endgroup$– user70797Commented Aug 12, 2020 at 13:36
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
2
Moving one:
From the '+' to make a '6' gives the true equation $6-4=2$
answered Aug 11, 2020 at 17:13
-
$\begingroup$ Too small of an edit for me to suggest, but the numbers in the equation you wrote down are in the wrong order - not writing it here to prevent spoilers, but you need to swap 2 of the numbers around. $\endgroup$ Commented Aug 12, 2020 at 14:11
-
1$\begingroup$ No problem, the maths was still correct, but it was killing my OCD! $\endgroup$ Commented Aug 12, 2020 at 14:13
$\begingroup$
$\endgroup$
6
I suppose you could do:
5-4≠2 as well, because it's true!
-
7$\begingroup$ That's an inequality, not an equation. $\endgroup$ Commented Aug 11, 2020 at 22:17
-
2
-
3$\begingroup$ Alternately, almost the same, ≤ $\endgroup$ Commented Aug 12, 2020 at 11:22
-
$\begingroup$ @Ruadhan2300 I'm not sure you can really make a ≤ moving only 1 line, I guess you could add an angled line above an
=, but that would be pretty far from the normal representation of that symbol. $\endgroup$– DBSCommented Aug 12, 2020 at 12:18 -
1$\begingroup$ I've seen it written with two parallel lines and one angled in the past, though I'll admit it's not the norm. I think it'd be understood anyway :) $\endgroup$ Commented Aug 12, 2020 at 13:07