A partial solution complementary to IanMacDonald's.
It is possible for this pair
S and Z
Place them in a fairly symmetric array, as follows. S is mirror image of Z.
 Now to prove that the minimal number of pieces is eight!
Now to prove that the minimal number of pieces is eight!
There is another tile that works:
It's "T" time [again]:
Again this seems to require eight tilesIt looks like this requires seven tetrominos.
Added proof that it is impossible to get out of "JaiL"
There is a pair of tetrominos that are unable to tile the chessboard.
This is a fairly exhaustive (and exhausting) proof that J tetromino (and by symmetry the L tetromino) cannot tile the board.
The proof starts by showing that tiling is impossible on an infinite board.
Then to avoid this on the chessboard, all pieces must be in close proximity to an edge or corner.
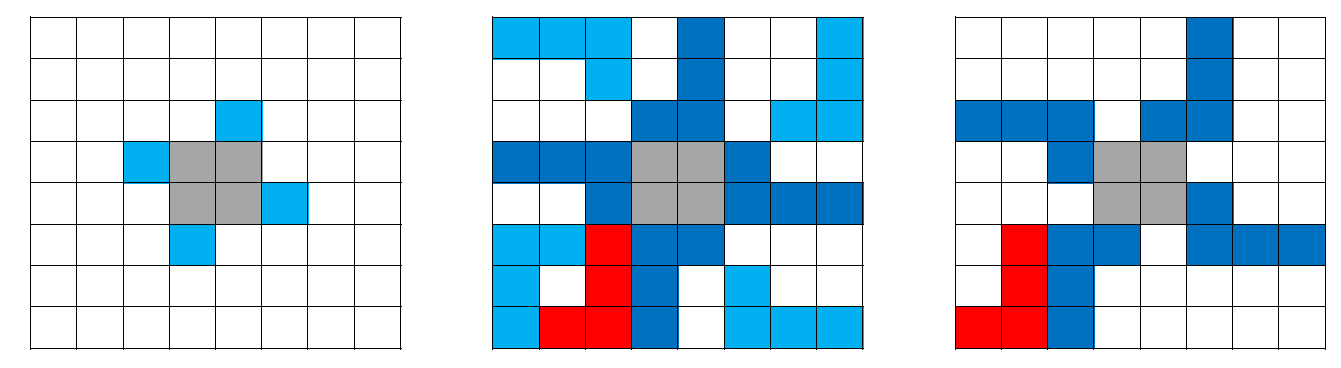
The next diagram shows there are three mandatory cells around every piece (blue) - these must be occupied or there will be a vacancy (gray) of the J shape. These are shown in light blue, assuming the playing field is rotated so that piece 1 is oriented as shown.
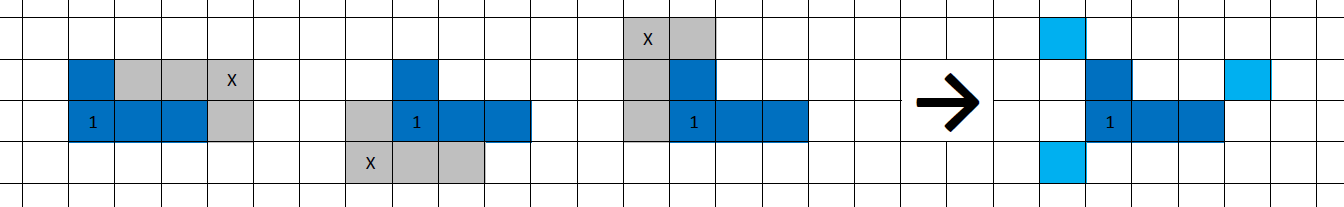 Then there are four possible positions of a second piece to cover the mandatory cell on the right. Two of these are shown in the next diagram. Both of these lead to a J vacancy because the second piece introduces new mandatory cells that cannot be occupied simultaneously (the red X's). Neither of these can be moved close to the top edge of a chessboard to avoid vacancies. In the first case, the gray J on the left will then be unavoidable. In the second case, rotational symmetry means the upside-down arrangement is very far from the new top edge.
Then there are four possible positions of a second piece to cover the mandatory cell on the right. Two of these are shown in the next diagram. Both of these lead to a J vacancy because the second piece introduces new mandatory cells that cannot be occupied simultaneously (the red X's). Neither of these can be moved close to the top edge of a chessboard to avoid vacancies. In the first case, the gray J on the left will then be unavoidable. In the second case, rotational symmetry means the upside-down arrangement is very far from the new top edge.
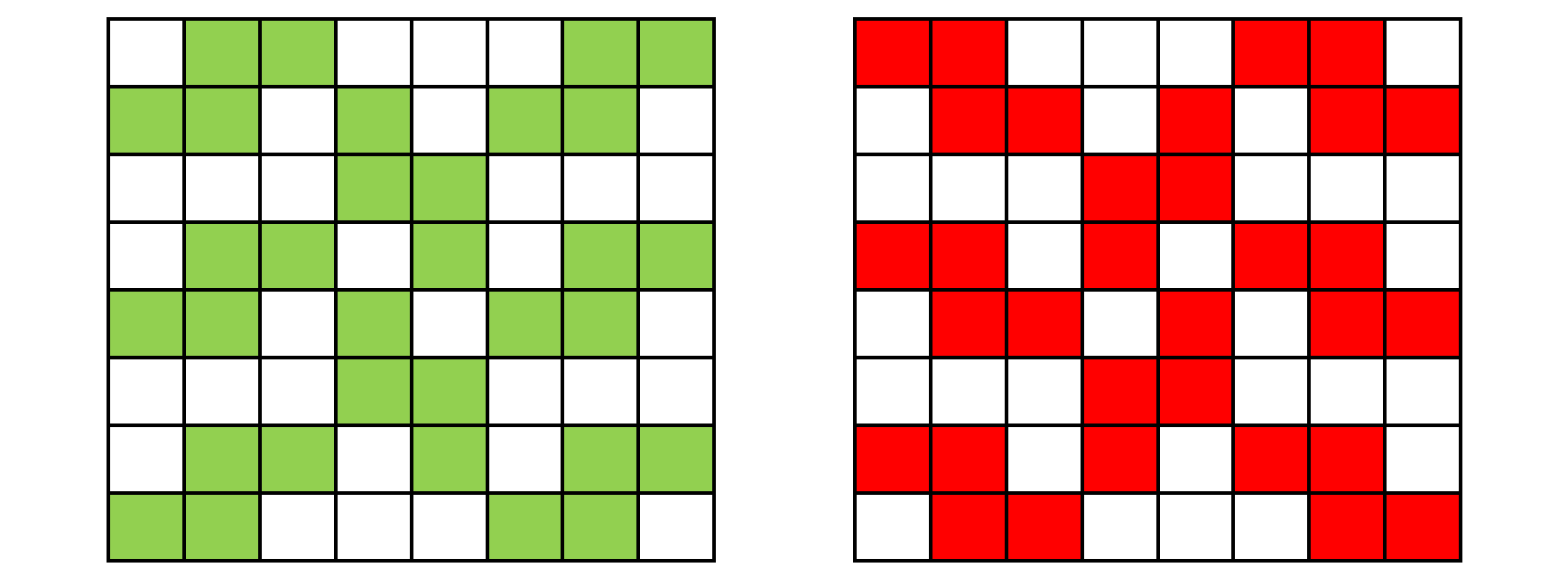
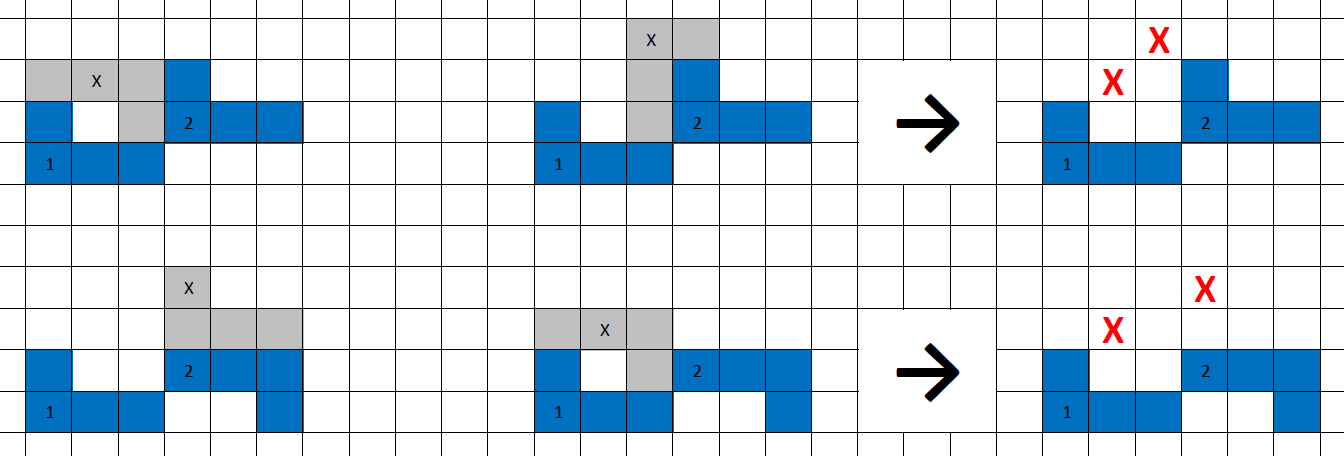 Then one other orientation immediately leads to a J vacancy, so there is only one possible orientation of the second piece relative to the first. This applies to a third piece relative to the second, and so on. So the result is a closed figure with an interior that cannot accomodate any more non-touching J pieces, but which has J vacancies.
Then one other orientation immediately leads to a J vacancy, so there is only one possible orientation of the second piece relative to the first. This applies to a third piece relative to the second, and so on. So the result is a closed figure with an interior that cannot accomodate any more non-touching J pieces, but which has J vacancies.
 In the last case, the cyclic arrangement can be avoided by placing the first two tetrominos against the top edge. But then, one of the next three arrangements results (the case where there is one column to the left is trivial), where placement of all the light blue pieces is forced, resulting in a J vacancy or touching edges in each case.
In the last case, the cyclic arrangement can be avoided by placing the first two tetrominos against the top edge. But then, one of the next three arrangements results (the case where there is one column to the left is trivial), where placement of all the light blue pieces is forced, resulting in a J vacancy or touching edges in each case.
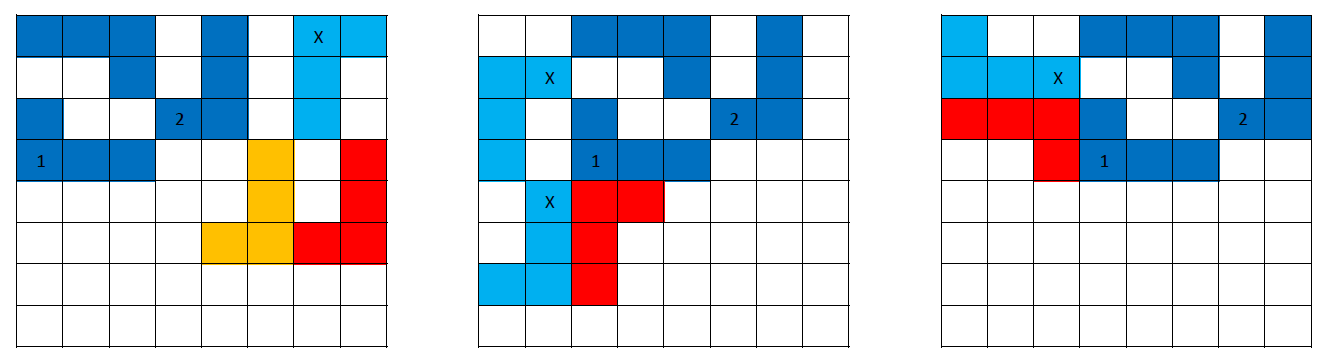
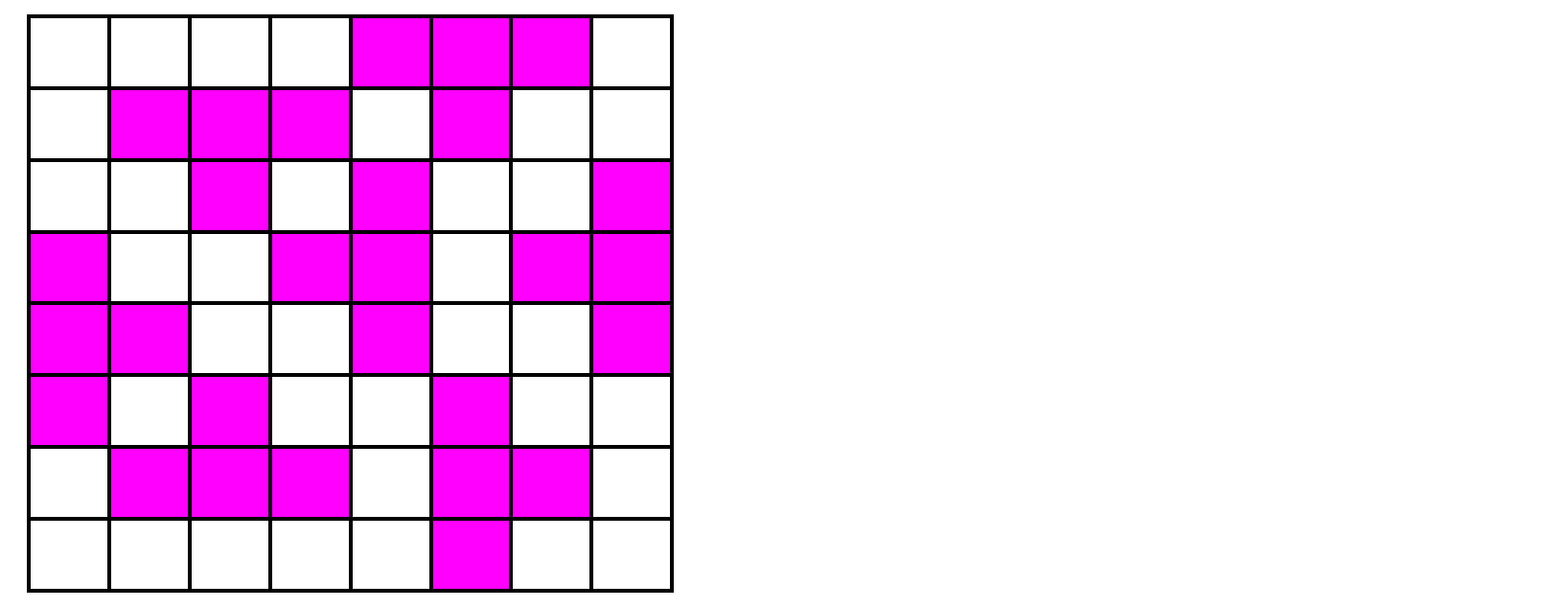
The only other way to avoid one of these arrangements is to place tetromino 1 so that it is abutting the right edge of the chessboard, so that there is no need to place tetromino 2 as in previous arrangements. Then **all tetrominos* must be placed this way with the "top" of the J touching an edge. The resulting arrangement leaves a 2x2 vacancy at the center of the board and hence the four mandatory cells in the next figure. Then J pieces can be placed in one of two symmetric ways (otherwise one of the mandatory cells will be vacant). But then it is impossible not to leave a J vacancy.