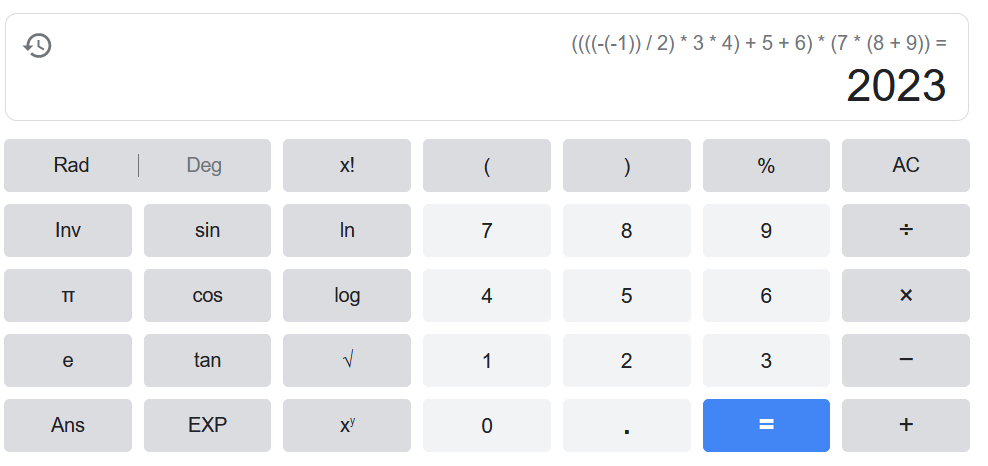
Using only the digits, 1, 2, 3, 4, 5, 6, 7, 8, and 9, in that order, how can you make the number 2023 using the +, -, ✕ and ÷ operations? (You can use as many parentheses as you want)
$\begingroup$
$\endgroup$
4
-
$\begingroup$ There are two solutions, one has already been found. $\endgroup$– RonnieChenCommented May 1, 2023 at 22:41
-
$\begingroup$ Clue: $2023=7^2\times17$ $\endgroup$– RonnieChenCommented May 2, 2023 at 4:08
-
7$\begingroup$ I think you mean $2023=7*17^2$. $\endgroup$– isaacgCommented May 2, 2023 at 4:20
-
$\begingroup$ yes, right. thank you $\endgroup$– RonnieChenCommented May 3, 2023 at 12:14
Add a comment
|
5 Answers
$\begingroup$
$\endgroup$
2
I don't think this one has been said yet:
$(1 - 2 - 3*4 + 5*6)*7*(8 + 9)$
No division needed here either!
-
$\begingroup$ Yes, very similar to the one by isaacg. $\endgroup$ Commented May 5, 2023 at 15:03
-
$\begingroup$ @RonnieChen agreed, yet somehow distinct! $\endgroup$ Commented May 5, 2023 at 16:15
$\begingroup$
$\endgroup$
Using the fact that $7*17*17=2023$,
$(1-2+3+4+5+6)*7*(8+9)$
No division needed!
$\begingroup$
$\endgroup$
3
Here is the answer with only using $+$, $\times$, and $\div$ without any parentheses and $-$;
$1+2*3+4*567*8/9$
-
$\begingroup$ Sorry, you cannot combine digits to form a multi-digit number. $\endgroup$ Commented May 1, 2023 at 22:39
-
6$\begingroup$ @RonnieChen in the question, you do not say you can use parentheses either :) $\endgroup$– OrayCommented May 2, 2023 at 7:15
-
11$\begingroup$ Actually, this is the most accurate answer given the question as it is written: digits are "pieces" of a number and parentheses aren't in the allowed symbol list. Great job! $\endgroup$ Commented May 2, 2023 at 10:05
$\begingroup$
$\endgroup$
1
Here's a solution using all the operations and the digits in the same order.
$((-(-1)/2)*3*4)+5+6)*(7*(8+9))$
Some proofs
Solve path
I looked for factors of 2023 and found 17 and 119. Looked for factors of 119 and found 7 amd 17. Tada, I can use 7,8,9 to get 119. Now, 17 is a prime number. No factors. So, a bit of trial and errors and we can easily use digits 1,2,3,4,5,6 to get 17. Idea was to use bigger digits 5,6 with additions/substractions and smaller ones for other operations.
-
$\begingroup$ That is correct, there is another solution $\endgroup$ Commented May 1, 2023 at 22:40
$\begingroup$
$\endgroup$
3
There are a number of solutions, but the first one I came across was:
(1 + 2 + 3) * 4 * 5 + 6 - 7 * (8 + 9)
-
10
-
1$\begingroup$ Looks like
((1 + 2 + 3) * 4 * 5 + 6 - 7) * (8 + 9)was actually meant. Or(1 * 2 * 3 * 4 * 5 + 6 - 7) * (8 + 9), for fewer parentheses. $\endgroup$ Commented May 2, 2023 at 10:49 -
$\begingroup$ Evidence:
dc <<<'1 2 3 4 5 **** 6+ 7- 8 9+*p'$\endgroup$ Commented May 2, 2023 at 10:53