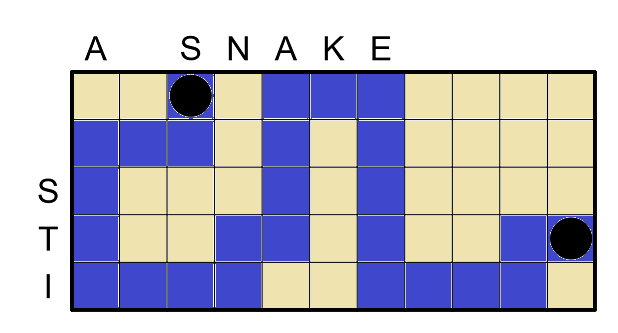
First of all,
Neither of S,N,A,K,E can be 0 because we couldn't connect the two ends in that case. Since there are only 5 cells for each column, each of S,N,A,K,E can be at max 5. And since the numbers need to be distinct, we know that each of 1,2,3,4,5 must appear exactly once.
Now,
One of S,N,A,K,E must be 5. It can't be the S because we need to get both to the leftmost column (remember, A cannot be 0) and to the other end on the right-hand side of the grid. The 5 cannot be any of N,A,K either, because a 5 there would force both adjacent numbers to be 1 and the numbers must be distinct. So we conclude that the only possibility is E=5. This gets us started:
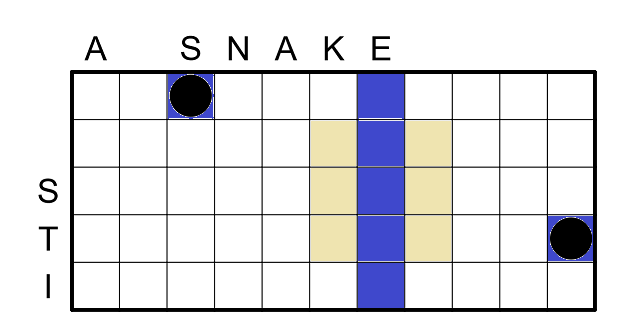
From here we see that
K must be 1, because there are only two cells available and filling them both would prevent us from reaching the other end on the right. A must be at least 3 because the snake needs to turn around without touching itself in the leftmost column. If A=3, N must be 2 because 1,3 are taken by K,A and there are only 3 cells available without touching the snake in the A column. And if A=4, N must also be 2 because there are 2 free cells and the 1 is already taken by K. So we know that K=1, N=2 and E=5, and A,S are 3,4 in some order.
The leftmost A column
Must have a filled cell in the middle, regardless of whether A is 3 or 4.
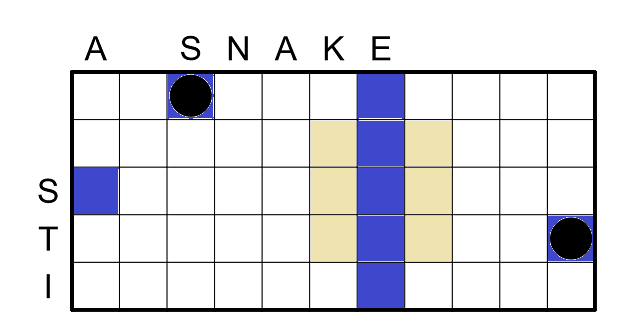
If K is filled at the bottom,
We can't fill A=(3,4) and N=2 without touching the black circle on the S column, which means the snake goes straight from the S column to E without going to the leftmost A column first. We can't do that, so we conclude that K must be filled at the top. This gives us most of the N and A columns as well as the column to the right of the E.
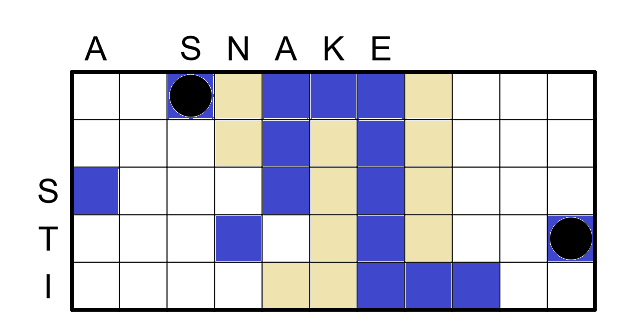
Looking at the S column,
There is no legal way to fill in 4 cells without touching the snake in the adjacent N column. This means we know A=4 and S=3, which gives us the entire centre of the grid as well us the entire S row in the middle, which also conveniently blocks off all the cells in the top-right corner. And since we now know A=4, we can finish off the left-hand side of the grid as well.
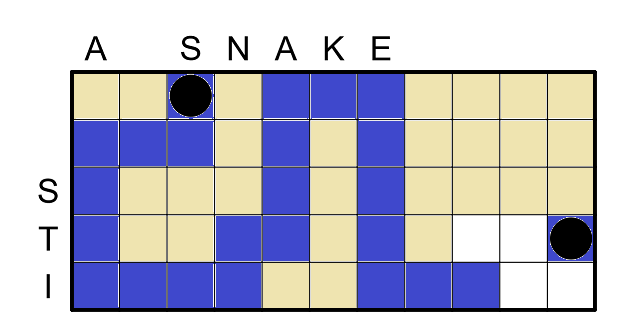
Finishing it off,
The snake cannot turn up immediately because that would make I=T=7. It also cannot go into the corner since that would make T=E=5. So there's only one place where it can make that turn, giving T=6 and I=8. Finished!