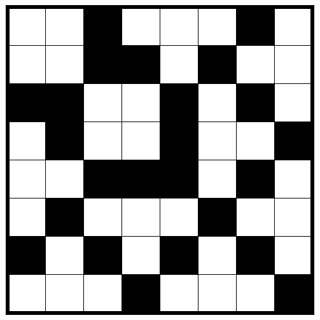
Here is another solution with the same number of squares as @msh210's:

For 24,

This looks very different to @msh210's, and has the nice property that

All blank regions are tetrominoes

Observation:

This solution looks quite ad-hoc apart from the symmetry, which could possibly indicate a solution with fewer squares is probably possible?

Here is another solution with the same number of squares as @msh210's:

For 24,

This looks very different to @msh210's, and has the nice property that

All blank regions are tetrominoes

Furthering on from that:

We might try a perimeter argument. Suppose there were 17 filled squares. Since all the blank regions are tetrominoes or smaller, the total perimeter of the blank regions is at least 2 times the number of blank squares. Also, the total perimeter is 4 times the number of filled squares plus all the border edges minus twice the number of border edges adjacent to a black square (at least six). Thus 94=472<=total perimeter<=417+32-2*6=88, a contradiction. So there are at least 18 filled squares.