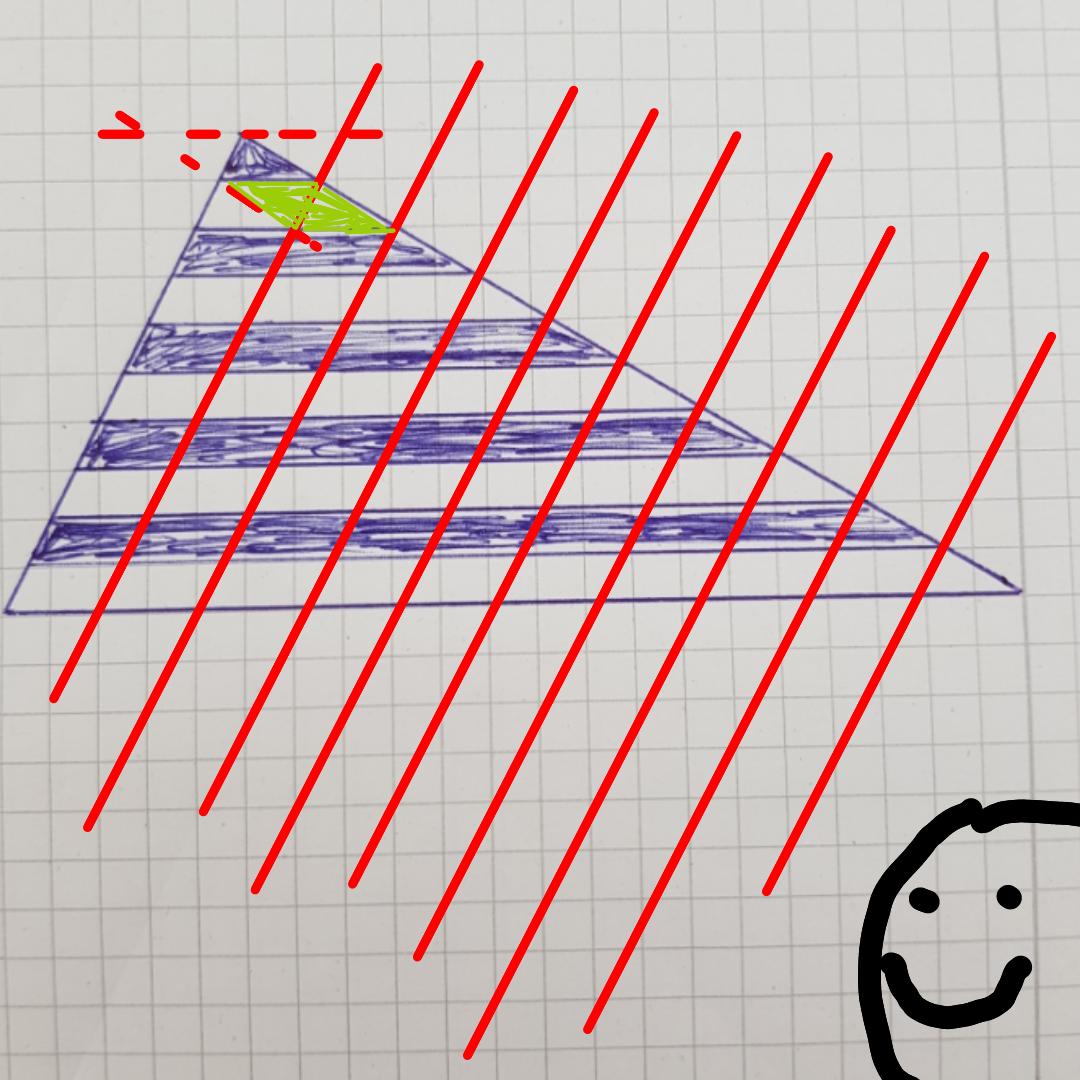
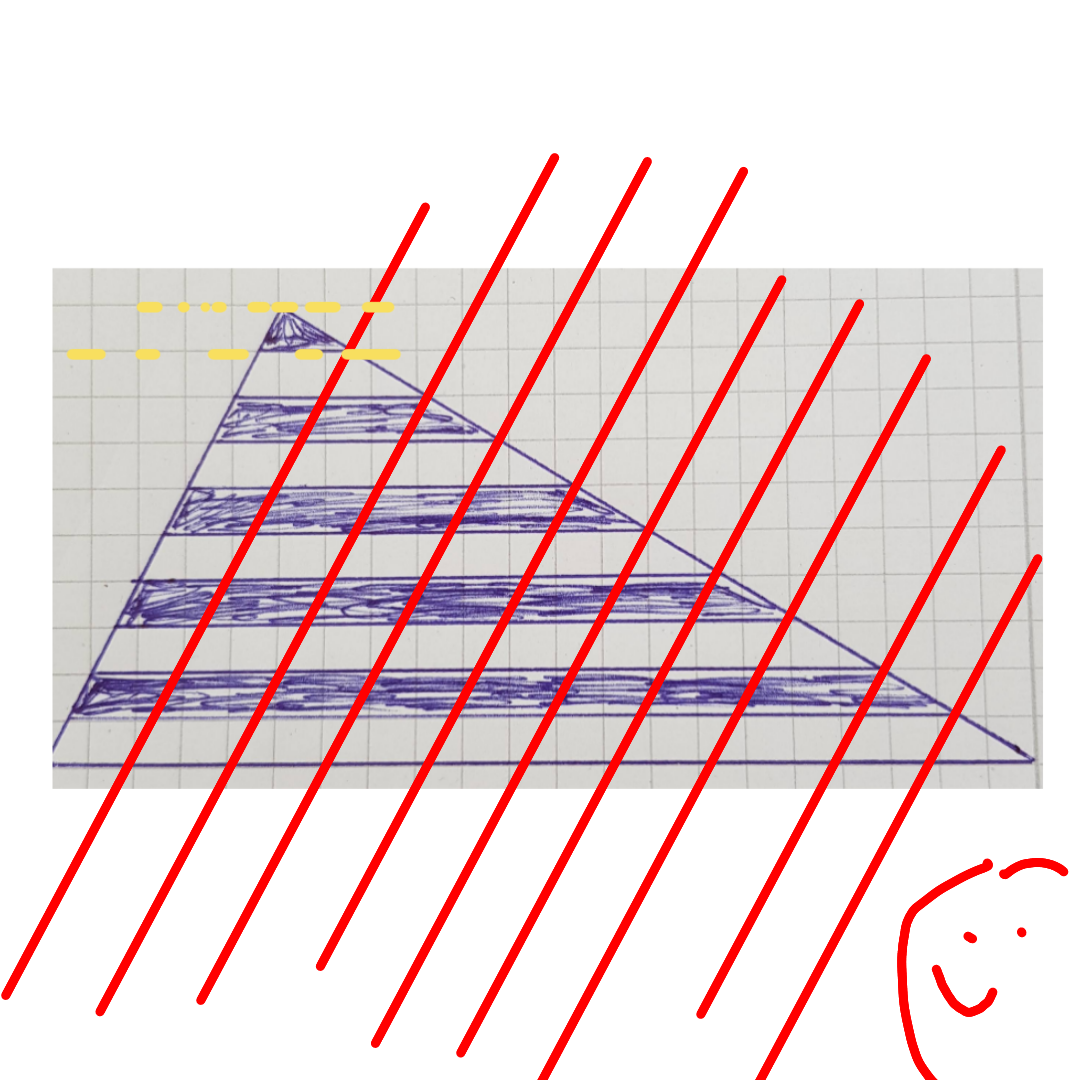
If you draw parallel lines to the left side of the triangle, you see that parallelograms and half parallelograms arise. On each ten segments divided by the red lines, you see that there is always one half white parallelogram more than the blue parallelograms. So there are 10 half white parallelograms more than black. Also you can see that each strip contains a number of these half parallelograms.
Total number of triangles:
$sum(2*n-1) = 100$, n starts at strip 1 and goes to strip 10.
w : white half parallelograms
b : blue half parallelograms
b + w = 100,
w = b + 10,
2 * b + 10 = 100,
b = 45
So...
45/100 = 45%
Image: