You only need to have read Part 1 to understand this question, reading Part 2 will only help understanding the epic storyline.
Your daughter refuses to talk to you even though you have (once more) replaced the numbered stickers you took from her. Another outgoing highway is being built, leading out from the city of Savage. This time you were prepared. You outsourced the production of the signs to another company whose sign-printing machine was functional. The shipment of signs arrived the same day you were required to place them, but when you opened the crates you saw this:
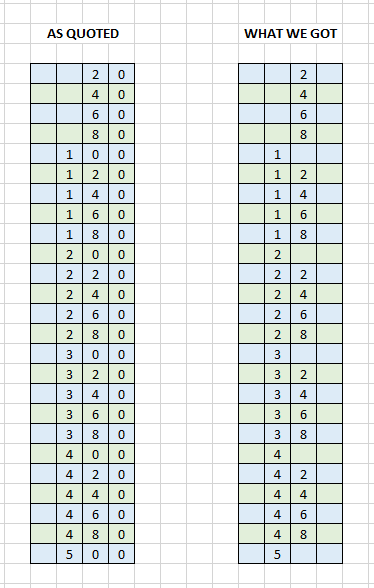
All of the zeros hadn't actually been printed! This can be fixed, however! We only need to steal the stickers again and surely we can figure something out. You run into your daughter's room to find her laughing like mad while throwing the pages of stickers into a trash can with flames coming out of it. You spill out the contents of the burning can and stamp out the fire. From the ashes you have recovered only some of the stickers... we now only have three of each digit!
What is the furthest distance marker sign you can place without breaking highway code?
This problem isn't open-ended but per @Rubio your answer should give a reasonable explanation as to why it can't be improved upon. Doesn't have to be a math proof, just convince me.
Answers should also identify which digits are stickers. I suggest using bold for the stickers like this: 148
Clarifications:
You only have the 25 signs shown above (missing zeros)
You have 30 total stickers (3 of each digit) that can be added to these signs. As before, 6's are different from 9's (no funny business)
Code requires 20km maximum difference between signs
As my chart hopefully implies, the signs are only big enough to fit 4 digits, and you can only add a sticker to a blank spot
Right now, the first sign in the list would read as 2. Adding a 5 in front of it would make 52. Then, adding a 6 you could make it 652 or even 526. The fact that the 2 was originally intended for the tens place means nothing. Beyond that idea, there shouldn't be any other "lateral-thinking" in this problem
This question has nothing to do with Part 2. Signs are single-sided and show the distance from Savage, just like in Part 1.
