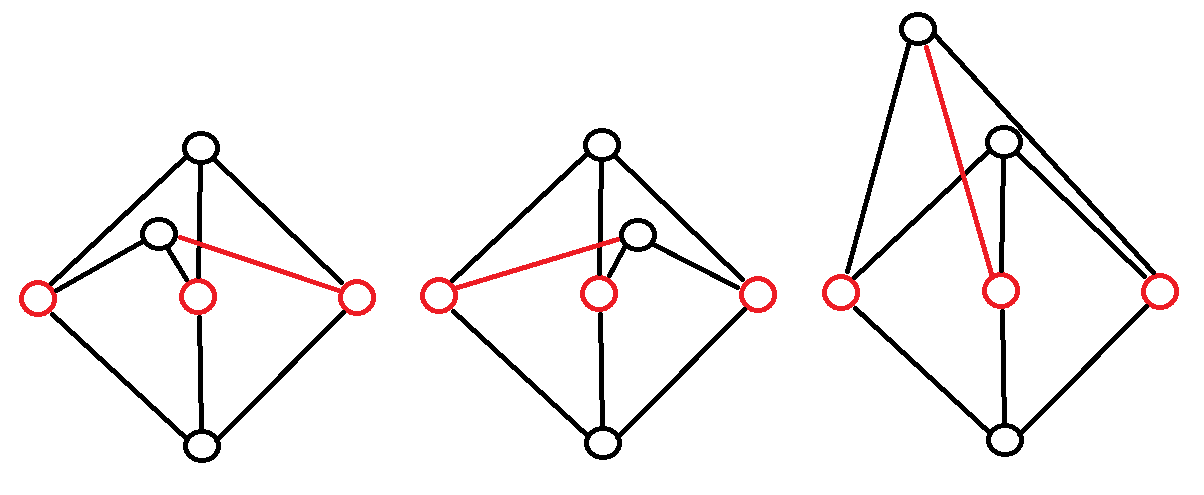
Simple proof of impossibility:
Draw the six lines connecting two black circles to all three red circles. This divides the plane into three four-sided "faces", with two black and two red circles on each face. Regardless of where you place the third black circle, it cannot reach the red circle not sharing its face, Q.E.D.