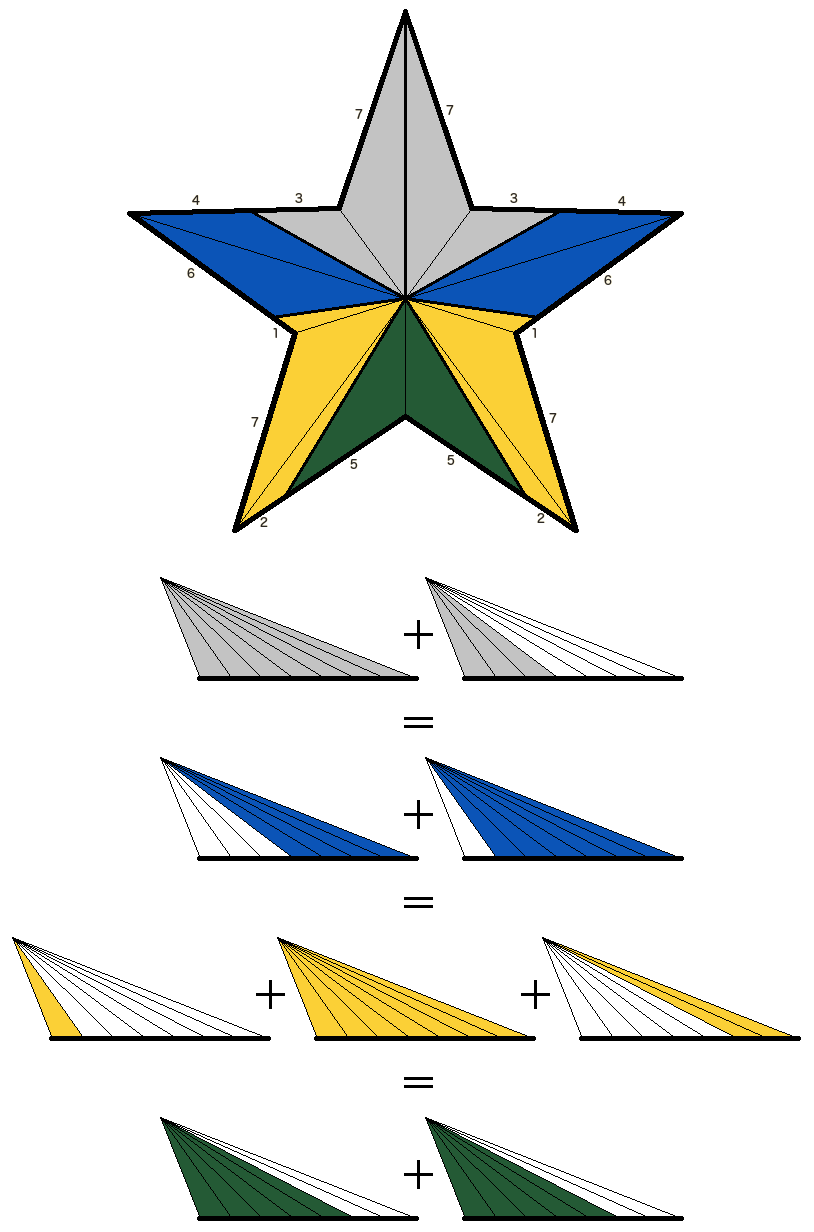
To divide the cake into n equal pieces,
To divide the cake into n equal pieces, simply divide the perimeter of the star into n equal lengths and cut from those points to the centre.
Each
Proof:
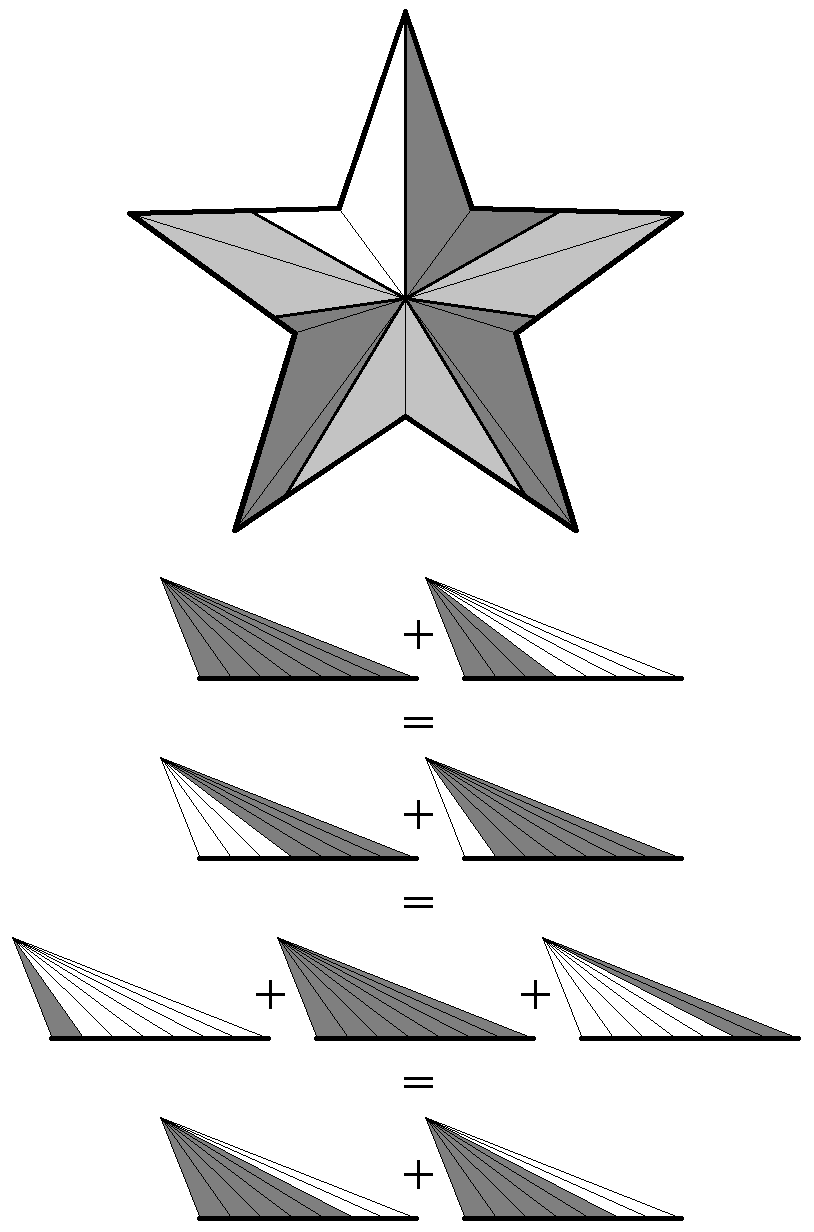
Each part of the perimeter can be seen as the base of a triangle with its apex at the centre of the star. Because of the symmetry of the cake, the heights of these triangles is the same. When you cut the cake into pieces by radial lines from the centre, each piece is made up of such triangles, so their top surface area is proportional to the total length of the bases of these triangles, which is equal to the part of the outer perimeter that the piece covers.
For each piece, not only is their top surface frosting area proportional to the outer perimeter used by the piece, so is the volume, as well as the area of the frosting on the side of the piece.
(At least to a first approximation, as it ignores the thickness of the frosting. This makes a small difference at the edges of the cake. In a pointy star, the tips of the stars will be all frosting and no cake, so a piece with a tip will actually have a little more frosting than a piece without).
Here is a drawing to illustrate how it works:
Here is a drawing to illustrate how it works: