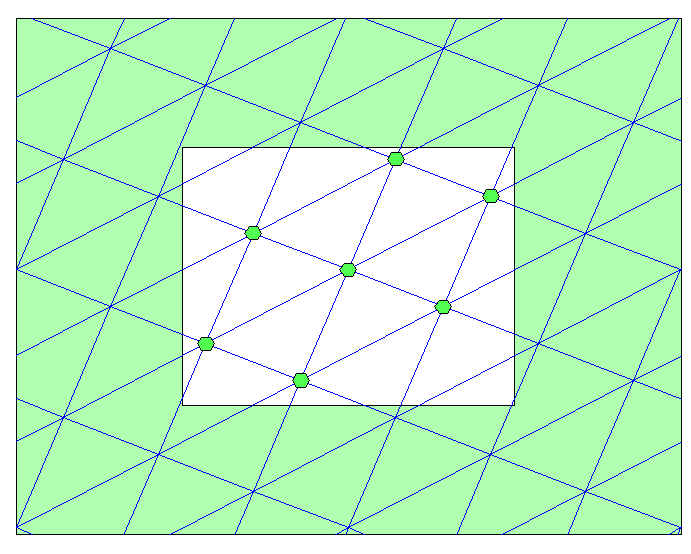
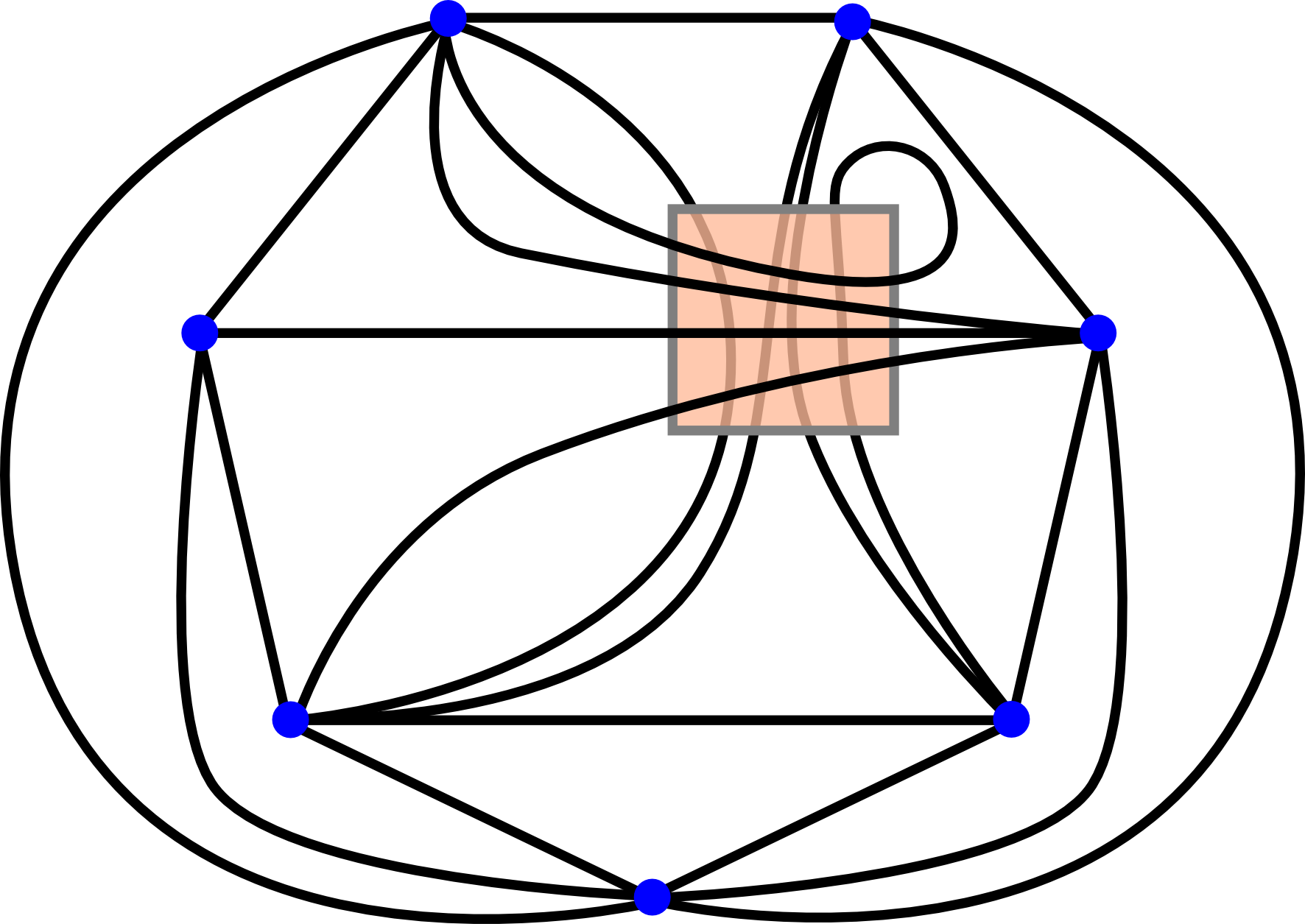
This is topologically equivalent to a torus, and you can go up to 7 points:

as shown by this Math.SE answer. (The diagram is too complicated to draw on the coffee cup, though.)

This is topologically equivalent to a torus, and you can go up to 7 points:

as shown by this Math.SE answer.

The diagram for this could look for example like this:

In this picture the lines going "under" the square represent connections going through the cup handle and the lines going "over" the square would go along the handle of the cup.

One can also just look up the answer if you know the question asks for the complete graph $K_n$ of degree $n$ with maximum $n$ such that the graph genus $\gamma (K_n)$ is at most $1$. Then

if you take the equation from Wolfram MathWorld $$ \gamma (K_n) = \left\lceil \frac{(n-3)(n-4)}{12} \right\rceil $$ you see that the genus $\gamma (K_n) \le 1$ as long as $n \le 7$.