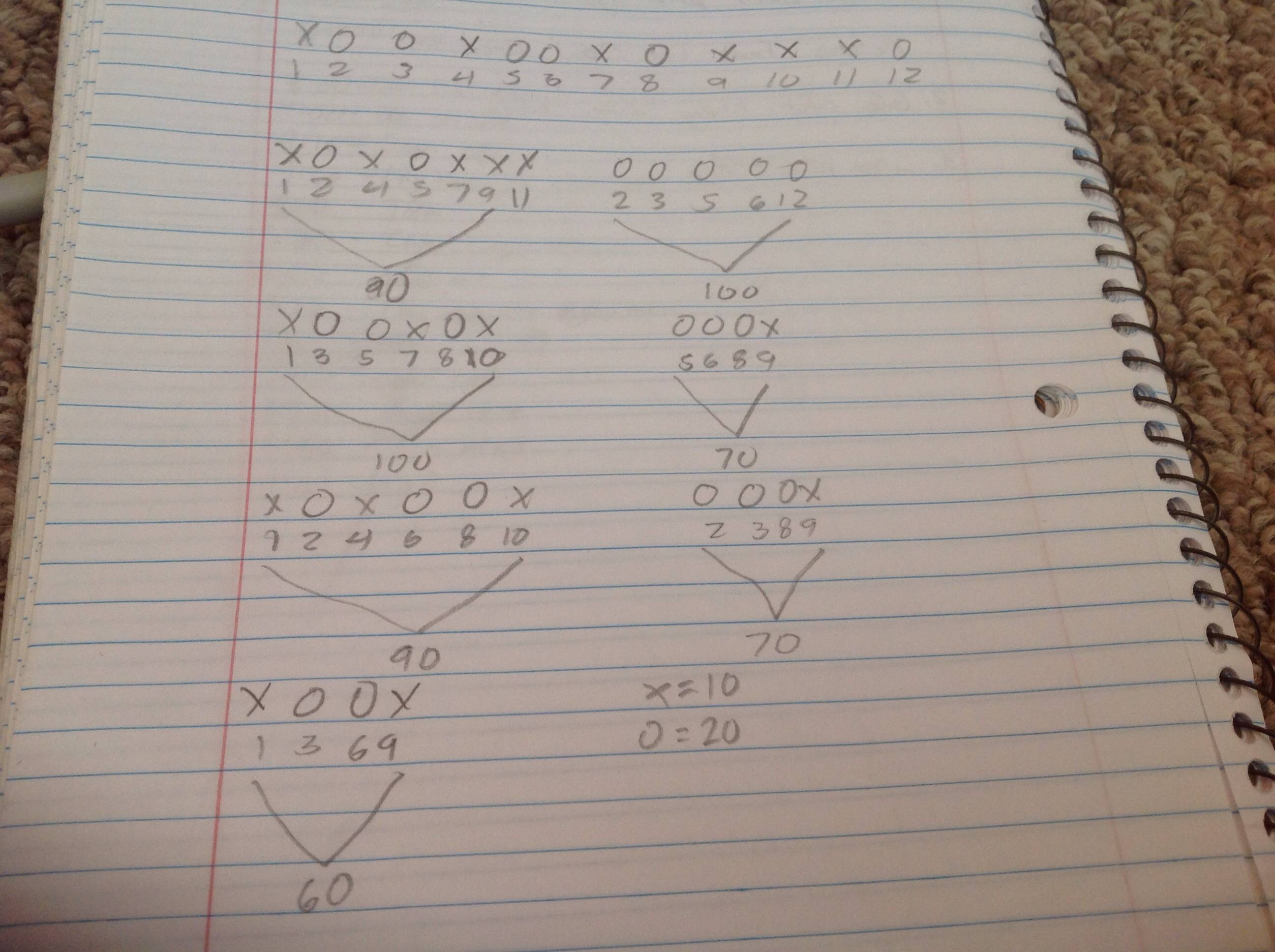Return to Answer

Edit: Revised Formula soon!

The least needed is:$7$.
First, $1,2,3,4=w$
Next, $5,6,7,8=x$
Also, $9,10,11.12=y$
3 weighings...
Then, weigh $1,2,5,11=a$; $3,9,7,10=b$; $6,8,4,12=c$
Another 3 weighings.
If you do guess and check, you will find some number pairs can be two numbers...
Ex:$1,3$ or $5,6$ The 7th weighing is $1,4,5,7=d$
With that measurement, you can confirm your guess and check.

Last Measurement

If say, you reach a pair of numbers, 3 and 4, and together they contain one light and one heavy coin, you need to weigh 3 and a coin number that is close to definite. For example, if the two pairs you can't differentiate are 9,10 and 3,4; you may weigh 3,2,9,11 or 1,3,8,9. Can't differentiate means that 9 could be a heavy or light coin, and 10 is the opposite of 9.

This is my revised guess and check strategy. You may argue that it's pointless and wastes time, but I don't want to spend the weekend reading up on college-level math.

Not sure if this is static or dynamic

First, weigh the below coins and note the weights.

• 1, 2, 4, 5, 7, 9, 11
• 2, 3, 5, 6, 12
• 1, 3, 5, 7, 8, 10
• 5, 6, 8, 9
• 1, 2, 4, 6, 8, 10
• 2, 3, 8, 9
• 1, 3, 6, 9

Thanks to @Julian for the weights... Then write all of them like this:

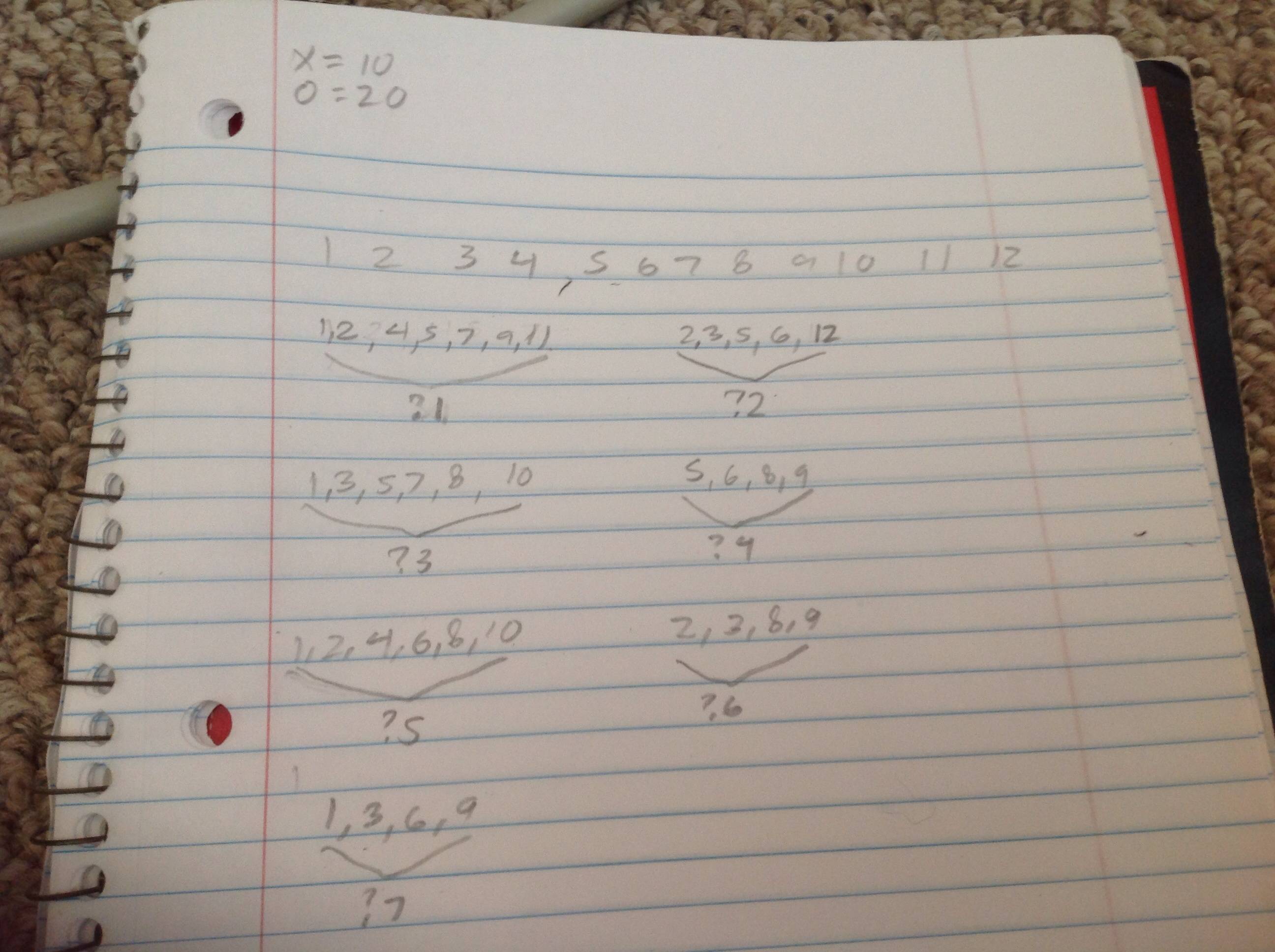
Substitute the ?$n$ for the actual weights. Then, see if there are any no guesses-like weighing $2$ being 100, or etc.

Guessing Tips

-Guess only when there are no more weights that can be deduced. -Try to only guess 1 weight at a time, never more than 2 -Use scratch paper and keep the un guessed version in case you messed up

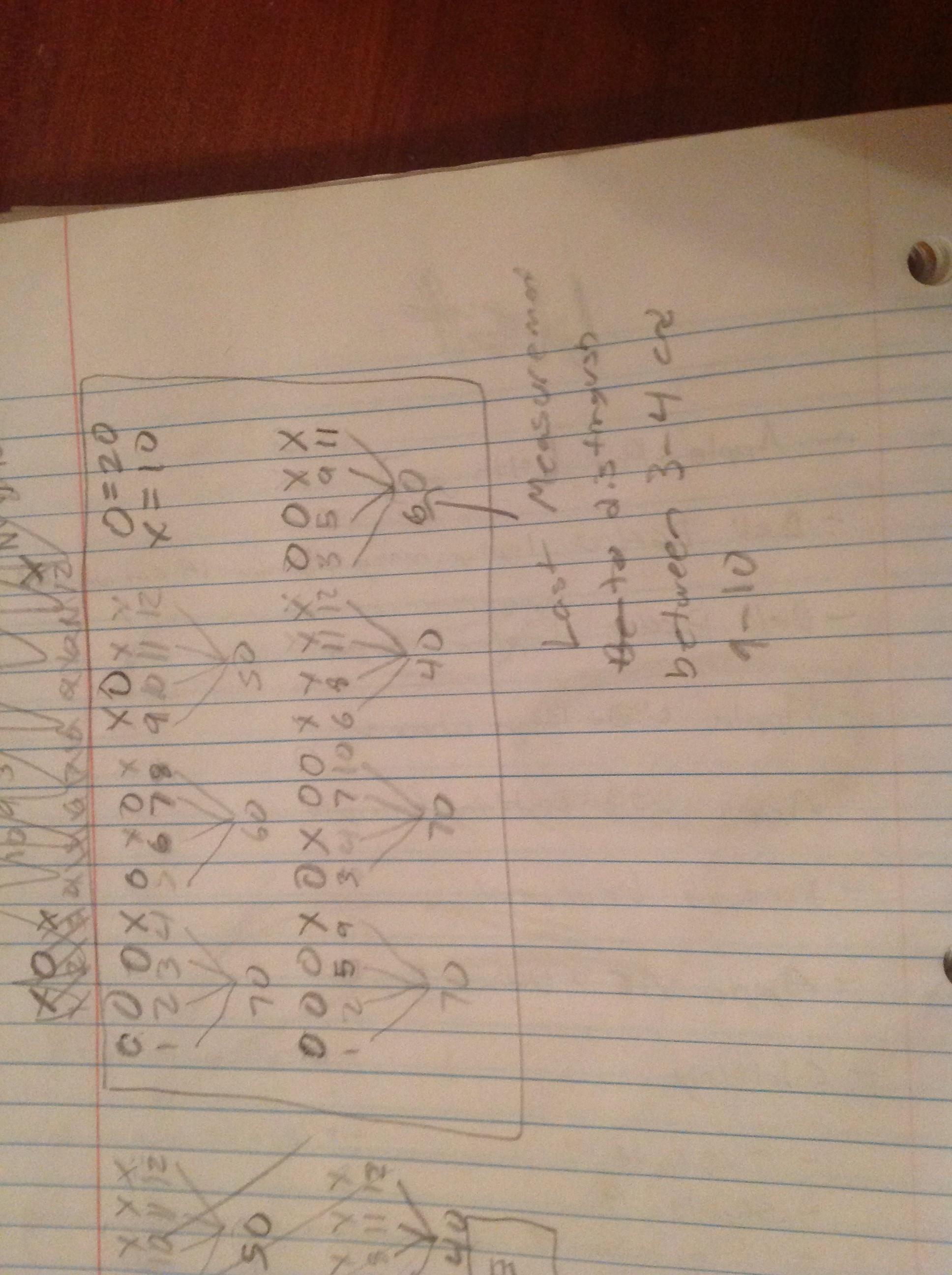Example of finished weights:

The least needed is:$7$.
First, $1,2,3,4=w$
Next, $5,6,7,8=x$
Also, $9,10,11.12=y$
3 weighings...
Then, weigh $1,2,5,11=a$; $3,9,7,10=b$; $6,8,4,12=c$
Another 3 weighings.
If you do guess and check, you will find some number pairs can be two numbers...
Ex:$1,3$ or $5,6$ The 7th weighing is $1,4,5,7=d$
With that measurement, you can confirm your guess and check.

Last Measurement

If say, you reach a pair of numbers, 3 and 4, and together they contain one light and one heavy coin, you need to weigh 3 and a coin number that is close to definite. For example, if the two pairs you can't differentiate are 9,10 and 3,4; you may weigh 3,2,9,11 or 1,3,8,9. Can't differentiate means that 9 could be a heavy or light coin, and 10 is the opposite of 9.

Edit: Revised Formula soon!

The least needed is:$7$.
First, $1,2,3,4=w$
Next, $5,6,7,8=x$
Also, $9,10,11.12=y$
3 weighings...
Then, weigh $1,2,5,11=a$; $3,9,7,10=b$; $6,8,4,12=c$
Another 3 weighings.
If you do guess and check, you will find some number pairs can be two numbers...
Ex:$1,3$ or $5,6$ The 7th weighing is $1,4,5,7=d$
With that measurement, you can confirm your guess and check.

Last Measurement

If say, you reach a pair of numbers, 3 and 4, and together they contain one light and one heavy coin, you need to weigh 3 and a coin number that is close to definite. For example, if the two pairs you can't differentiate are 9,10 and 3,4; you may weigh 3,2,9,11 or 1,3,8,9. Can't differentiate means that 9 could be a heavy or light coin, and 10 is the opposite of 9.

The least needed is:$7$.
First, $1,2,3,4=w$
Next, $5,6,7,8=x$
Also, $9,10,11.12=y$
3 weighings...
Then, weigh $1,2,5,9=a$; $3,4,6,10=b$; $5,6,11,12=c$
Another 3 weighings.
If you do guess and check, you will find some number pairs can be two numbers...
Ex:$1,3$ or $5,6$ The 7th weighing is $1,4,5,7=d$
With that measurement, you can confirm your guess and check.

Last Measurement

If say, you reach a pair of numbers, 3 and 4, and together they contain one light and one heavy coin, you need to weigh 3 and a coin number that is close to definite. For example, if the two pairs you can't differentiate are 9,10 and 3,4; you may weigh 3,2,9,11 or 1,3,8,9.

The least needed is:$7$.
First, $1,2,3,4=w$
Next, $5,6,7,8=x$
Also, $9,10,11.12=y$
3 weighings...
Then, weigh $1,2,5,11=a$; $3,9,7,10=b$; $6,8,4,12=c$
Another 3 weighings.
If you do guess and check, you will find some number pairs can be two numbers...
Ex:$1,3$ or $5,6$ The 7th weighing is $1,4,5,7=d$
With that measurement, you can confirm your guess and check.

Last Measurement

If say, you reach a pair of numbers, 3 and 4, and together they contain one light and one heavy coin, you need to weigh 3 and a coin number that is close to definite. For example, if the two pairs you can't differentiate are 9,10 and 3,4; you may weigh 3,2,9,11 or 1,3,8,9. Can't differentiate means that 9 could be a heavy or light coin, and 10 is the opposite of 9.