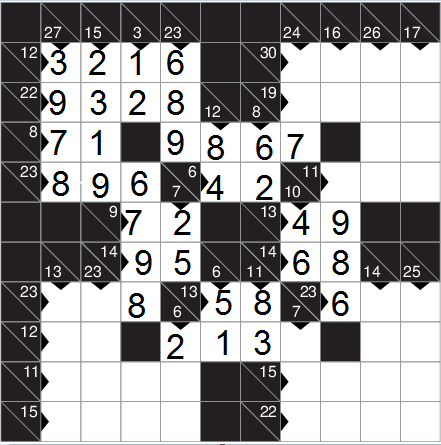
The final solution is:

Here are the steps:
First of all, focus on the top-left corner:
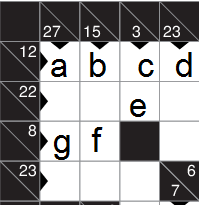
Here the only possibility for the vertical 23-run is 9 8 6. But 9 or 8 in d would render the 12-run unsolvable, so d=6. Then a+b+c=6, so they are 1,2,3 in some order. But there's no possible combination for 27 with 1 or 2, so a=3. Then the 27-run must be 3 7 8 9 in some order; but g can't be 9 or 8, so g=7, f=1; so b$\ne$1$\implies $b=2,c=1,e=2. Since the horizontal 23-run is 9 8 6, and we need 12 more for the 15-run, the only possibilities for the rest of the 15-run are 4 8 or 3 9. But in the first case, we'd need another 16 for the 22-run, forcing a 7 between a and g, which is bad since g=7. So the part is something like:
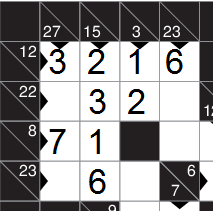
Now we'll solve four very similar regions. Focus on this part:

Clearly the only possibilities for the 12-run are 7 5 or 8 4. But the first case would imply d=1, b=7=a, which is bad. So (a,b,c,d)=(8,6,2,4). Similar strategy can be applied to the other 2x2 parts, giving:

Now that we know the 23-run considered in the first paragraph is 6 8 9, we can do some usual sum-chasing to arrive at the following:
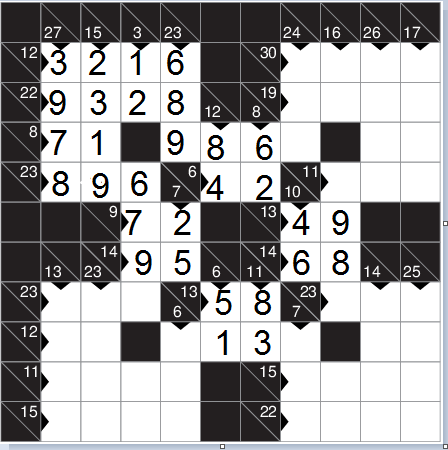
Now,
we apply some reasoning along the line "S has the only combination a1 a2 a3, but that cell can't have a1 or a2, so it's a3", to arrive at the following:
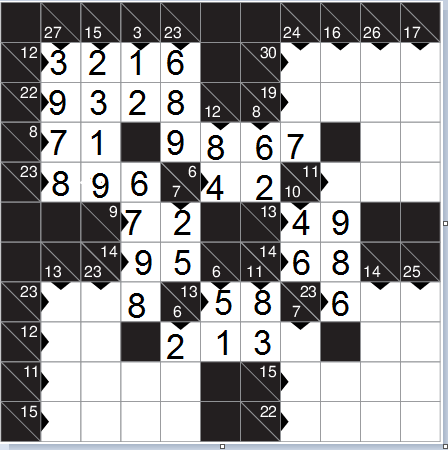
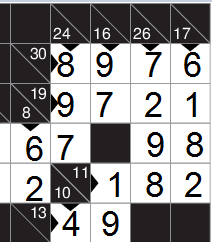
Next, consider the top-right corner.
The only possibility for the vertical 24-run is 9 8 7 in some order, and for 30, it's 9 8 7 6 in some order. So their intersection must be 9 or 8. If it's 9, then the 16-run must be 7 9, and the 24-run would be 9 8, which means we need to complete the 19 run from 8, 9 and 2 more numbers, which is impossible. So the only possibility is: 8 9 * * and 9 7 * *. Also, since the last two asterisks are 1 2 in some order, and there's no combination for 4-cell 26-run with a 1, we get that these two rows are 8 9 7 6 and 9 7 2 1. Now some sum-chasing leads to this:
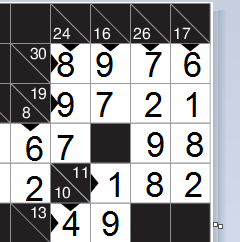
Now let's try the bottom-left corner.
The horizontal 23-run has to be 9 6 8, or 6 9 8; but the first possibility would mean we need to get a sum of 4 from 3 cells in the 13-run, so it has to be 6 9 8. The other three cells in the 13-run have to be 1 2 4 in some order. How 1 or 2 in that 12 run isn't possible, so the next row is 4 8.
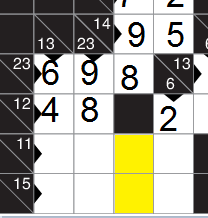
Now focus on the remaining 2x4 grid. By summing by rows and then by column, we conclude that the yellow cells must add up to 13; but the only possible combination for the 11-run is 1 2 3 5 in some order, so these yellow cells must be 5 8. Now the remaining cells in the bottom row are 1 2 4 in some order, and 4 fits only in the second cell. Now sum chasing yields the following:

To finish things off, look at the bottom right:
it's simple to get that the 23-run is 6 8 9 and the 7-run begins with 4. Now look at the 22-run. It's first element is 1 or 2 and the third element is among 1 2 3. So the other two cells must sum up to at least 22-(2+3)=17; but that's the maximum for two cells, so equality holds, and the 22-run is precisely 2 9 3 8. Now some quick casework yields the following:
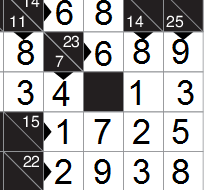
Now it's solved completely!