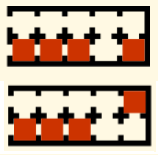
Send the first#0 kamikaze forward 2 spaces (spaces 1,3)
If If he lives, then we're in the first 8 scenarios from M Oehm's answer. If
he's he's dead, it's the last 8 scenarios. Since the 2 options are mirror images. I'll just paste the first 8:
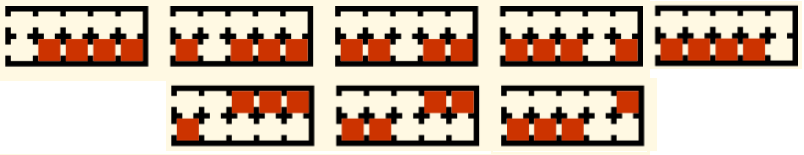
To solveFor the remaining 8, it's simple enough to just send a kamikaze towhen visited from the
end, then try top row exactly one space less until we don't hear himof the squares marked in yellow will not result in a scream. May as well check them in order. First kami scout is "lucky" and has 5/8 where he stays alive.
So: 2nd kamikaze goes all 5 spaces (top if scout1 lived, bottom otherwise). $40, 10/16. 3rd kamikaze goes 4 spaces same manner. $60, 2/16 4th kamikaze goes 3 spaces. $80, 2/16 No need for the 5th kami since the very first scout did that job.
`So:
- kamikaze goes all 5 spaces $40 total, 5/8.
- kamikaze goes 4 spaces then down 1. $60, 1/8
- kamikaze goes 3 spaces then down 1. $80, 1/8
The last yellow square is the answer if the #3 kami screamed. $80, 1/8
Total cost:
($40 * 5/8 + $60 * 1/8 + $80 * 1/8 + $80 * 1/8) = $52.50`
Note: If a happy-go-lucky was willing to work for 1 penny less, I could use a happy in-place of the first/second kamikaze. Half the time he'd survive to use his second order (save \$10). Half the time he wouldn't (extra \$10 cost).
SoDespite using all kamikazes, the total expected cost in lives is surprisingly "low":
$40 * 10/16 + $60 * 2/16 + $80 * 4/16 = $52.50`0. kami: 50-50 survival odds (4/8 chance of death)
- kami: 5/8 survival odds (3/8 chance of death)
- kami: 5/8 + 1/8 = 6/8 (2/8 chance of death)
- kami: 6/8 + 1/8 = 7/8 (1/8 chance of death)
Expected casualties is:
10/8 = 1.25`
**Bonus 2**Very similar to the base scenario, but test only the bottom 3 yellow squares.[![Bonus 2 order][3]][3]Cost is:`$40 2/8$60 2/8$80 4/8= $65`
And the lives lost is surprisingly "low":
1st kami: 8/16 survival odds (4/8 chance of death) 2nd kami: 5/8 survival odds (3/8 chance of death) 3rd kami: 5/8 + 1/8 = 6/8 (2/8 chance of death) 4th kami: 6/8 + 1/8 = 7/8 (1/8 chance of death)
So expected casualties is`0. kami:
10/8 = 1.25 50-50 survival odds (4/8 chance of death)
- kami: 2/8 survival odds (6/8 chance of death)
- kami: 2/8 + 2/8 = 4/8 (4/8 chance of death)
- kami: 4/8 + 2/8 = 6/8 (2/8 chance of death)
You: 7/8 (1/8 chance of death)
Expected Casualties is:
17/8 = 2.125`
Warning: You want the #1 kamikaze dead (2nd one sent). If he survives you're stuck with a choice, pay a scout $20 to take the 50-50 risk of death for treasure. Or do it yourself. Since you're nearly bankrupt, what have you got to lose?