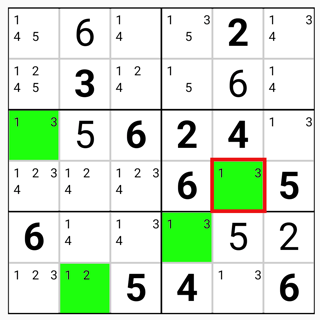
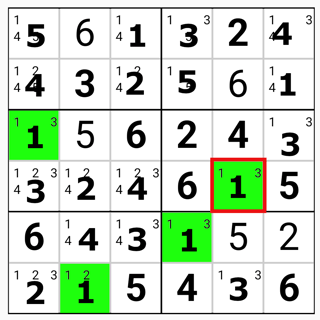
You can do sudoku to
whatever is in row 4, column 5.
That will eventually place the same digit in a spot where a three cannot go:
After that deduction, the grid will basically fill itself.
The technique that finds relationships like this is called "colouring"colouring the pair". When you have a lot of squares around the grid with the same two options only, it's a handy way to give an identity to the two options of the pair. Even if it hadn't conveniently placed a 1-or-3 in a spot that already sees a three, continuing to colour in the "squares that can only be 1 or 3, but cannot be green" in a different colour would have shown that the square in the bottom left corner (r6c1) sees both colours (both numbers of the 1-3 pair) and must therefore be a 2.