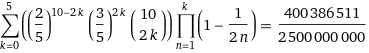I believe the exact answer you're looking for is
Explanation:
This follows from the answer to this related bullet problem (which is the product term in each of my summands). We are adding the probabilities for each case of 0, 2, 4, 6, 8, and 10 bullets fired, weighted by the probability computed via the binomial distribution of each of these cases occurring. Note that in the answer to this linked problem, the absolute speeds of the bullets do not matter but for their relative speeds compared to each other, and it does not matter that the gun in this problem may delay firing for several seconds between bullets.
This appears to corroborate Dmitry's rather than dipodomys's simulation, even if Dmitry's is flawed in design. The conclusion is at least one among the following: diopomys's simulations are flawed; my math is wrong; or my outsourced derivation is wrong, despite it being an accepted answer to the related problem. For example, to attest to the third point, I'm still not totally convinced that the inductive step of the argument for reducing n to n-2 bullets is statistically valid; see Penguino's comment in the link.
EDIT: The discrepancy has been resolved and all simulations now agree with this answer. I think it would still be nice to have a more rigorous proof for the inductive step, though.