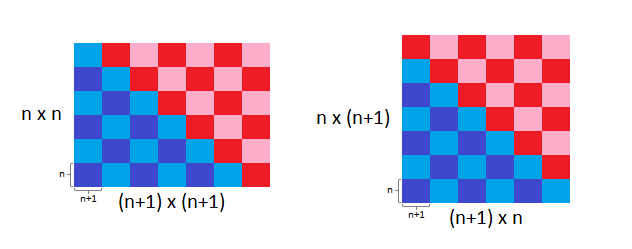The answer to your question:
Yes, this is always possible
Proof:
This solution, shown with n = 6$n = 6$, can be applied for all n$n$. The smaller sub-rectangles haverectangle with dimensions n$n^2$ by n+1$(n+1)^2$ is divided into $n\times(n+1)$ congruent rectangles with dimensions $n$ by $n+1$ such that there are $n$ rows of height $n$ and $n+1$ columns of width $n+1$. The rectangle is cut into two congruent pieces along a stair-step diagonal. These pieces can be arranged to form a square with edge length $n\times(n+1)$, divided into $n+1$ rows of height $n$ and $n$ columns of width $n+1$.