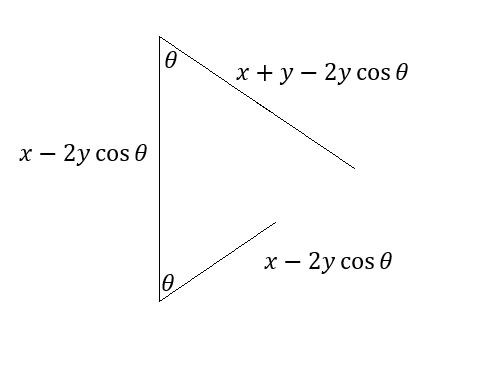ITL;DR
Our floating Robinsons can do this with $3$ pieces, but not with $2$
In this answer, I first show constructive examples with $4$ and then $3$ pieces, and then prove why $2$ pieces is impossible.
First, and easiest, we can do it in:
What about aNow that we understand that method, how can we do it better solution?
I have found a solutionWe can do it in three pieces. I found this, by attempting to prove it was impossiblein some sense combining two of the pieces from the four-piece dissection. After around an hour, and an (as I thought) almost complete proof, I realized a counterexample:
Cut the wood as follows: Choose an angle $\theta<90$ arbitrarily close to $90$ degrees. Then pick appropriate lengths $x,y$ such that the following 3 symmetrical trapezia may be cut out:
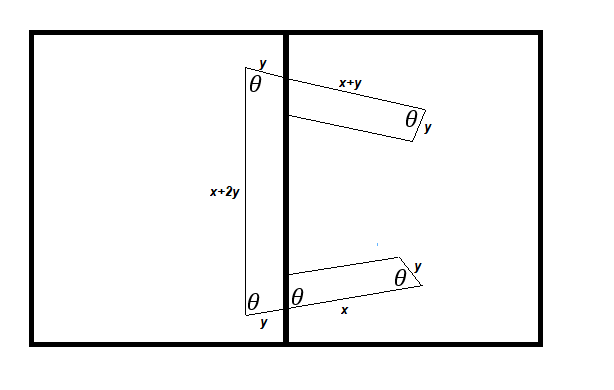
Then rearrange as follows:
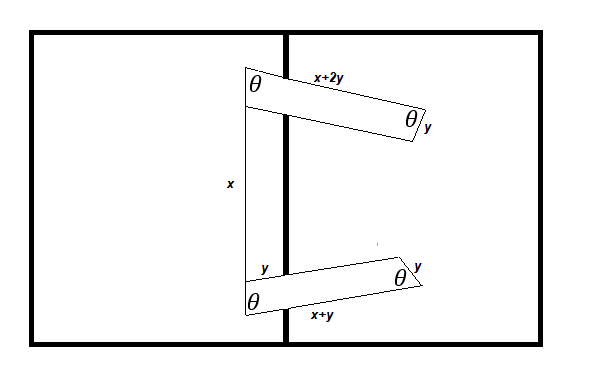
CanWe now need to prove that we cannot do better.
Suppose I have performed a dissection, cutting out two pieces and rearranging them such that the two rectangles are locked together.
Then there must be at least a single piece (call it $A$) in the rearrangement which crosses the dividing line $MN$ (otherwise our two rectangles could be slid past each other vertically). Now consider this piece by itself and imagine we have the following construction:

Now, take arbitrarily small left and right tangents to $P$ about the points $R$ and $S$ as follows, and consider the angles formed $w, x, y, z$:
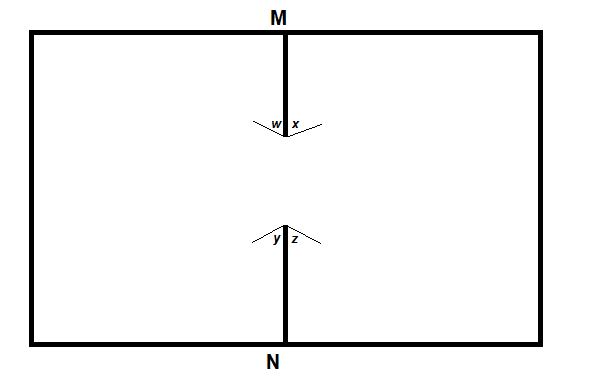
(Note that the left and right tangents about $R$ and $S$ need not be parallel, since $P$ may not be differentiable). Now suppose $w+x<180$, then we could construct a line as follows (arbitrarily close to the point $R$):
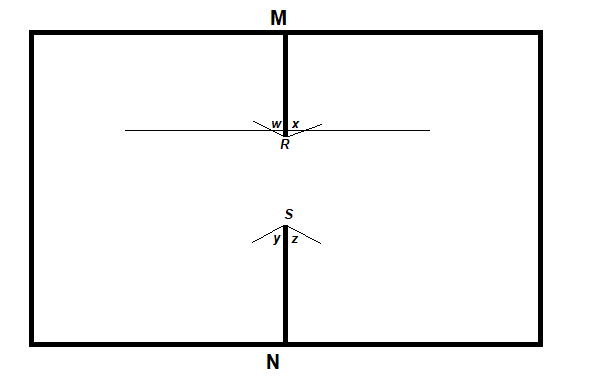
This line would be a straight line connecting two points in the shape $P$, but it would pass through the exterior of $P$. This would contradict convexity. Therefore $w+x\ge180$. By an identical argument, we can prove that $y+z\ge180$.
Now suppose $w+y<180$ and $x+z<180$. Then $w+x+y+z<360$ which is impossible, since $w+x\ge180$ and $y+z\ge180$. Therefore either $w+y\ge180$ or $x+z\ge180$. Without loss of generality, let $w+y\ge180$. Now extend these tangents as follows:
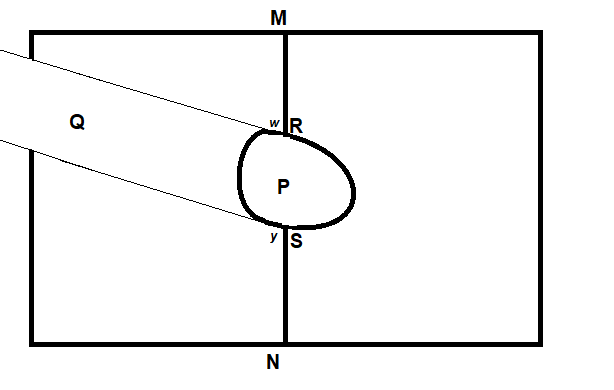
These tangents thus define a region $Q$ to the left of the line $MN$, which is either an infinite rectangle (if $w+x=180$$w+y=180$), or a triangle (if $w+x>180$$w+y>180$). The portion of $P$ that lies to the left of $MN$ must be wholly contained within $Q$. To see this, suppose without loss of generality that $P$ extends above $Q$. Then a line could be drawn through $R$ as follows:
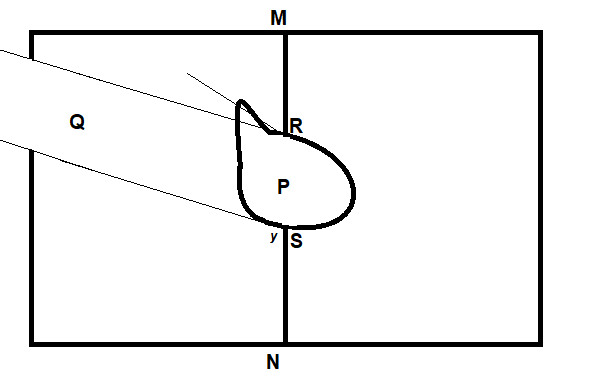
Which contradictcontradicts convexity of $P$.
Now my claim is that if we pull our left hand large rectangle in the direction shown by the following arrow, where the angle $u=\frac{w+x}{2}$$u=\frac{w+y}{2}$, then it can be pulled away from $P$ and the right hand larger rectangle.
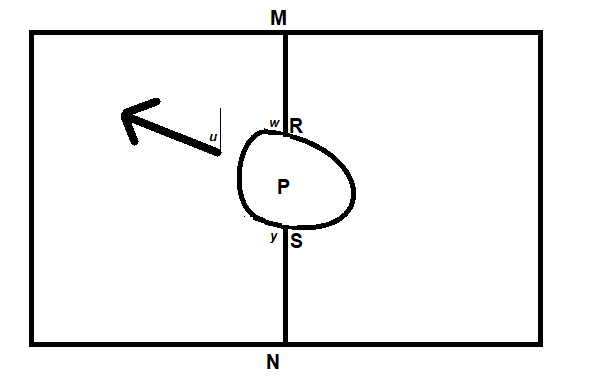
To prove this, assume the converse, i.e. that the left hand rectangle cannot be pulled away parallel to the arrow. Now consider all lines intersecting $P$ that can be drawn parallel to the arrow as follows:
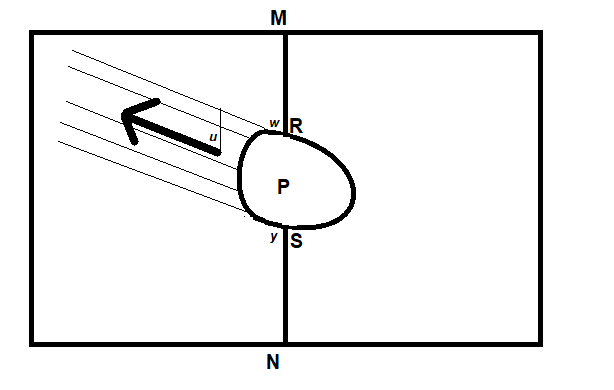
For there to be something preventing the left rectangle being pulled away, at least one of these lines (going left to right) would have to pass through part of $P$, and then pass through part of the rectangle $P$again before reaching $MN$. But at $MN$, all these lines pass through $P$ again, so if such a line existed, it would contradict convexity of $P$. Thus there is no line on which there is anything to stop the left rectangle being pulled away at an angle $u$.
Now, let us return to our final dissection (with pieces rearranged to lock the squares together). If only one piece in this dissection crosses $MN$, then we have shown there is some angle $u$ at which the left rectangle can be pulled to detach it. Thus there must be at least a second piece (call it $O$) that crosses $MN$ in the final dissection. Let us look at these two pieces alone (ignoring all others):
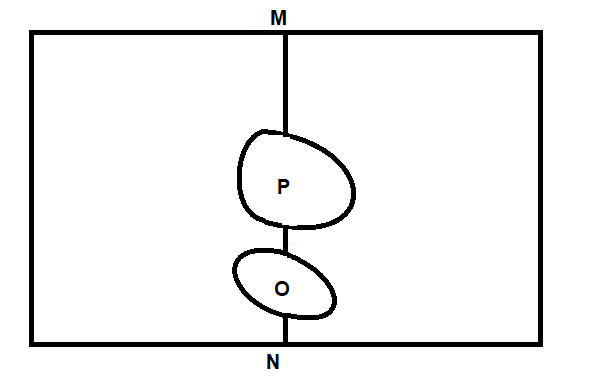
Now consider the cuts required to form these pieces. In making the initial cuts to form the slot that $P$ fits into, an area larger than $P$ may have been cut (to see why have a look at my example four-piece dissection, where $P$ corresponds to the piece $b$, which was formed as part of a larger rectangular cut). However, any cut that would generate the slot that $P$ fits into, must have crossed $MN$, and therefore have produced at least two different pieces (we label the parts that intersect $P$ as $P'$ and $P''$ - the actual pieces produced may have been larger). The same is true of $O$.
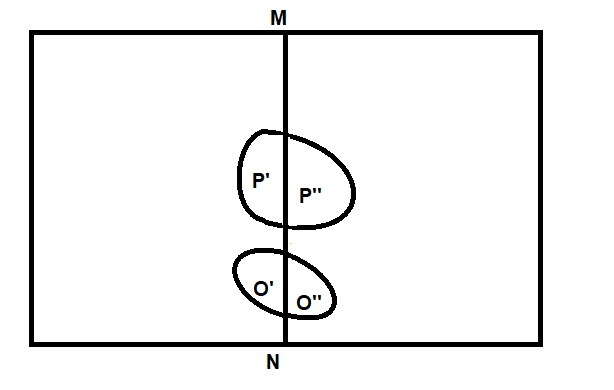
Thus for two pieces to be used, $P'$ and $O'$ must be part of one larger piece $\#$, and $P''$ and $O''$ must be part of one larger piece $\#'$. In the initial dissection (before rearrangment) all pieces must be entirely on one side of $MN$. Let us look at $\#$ by itself:
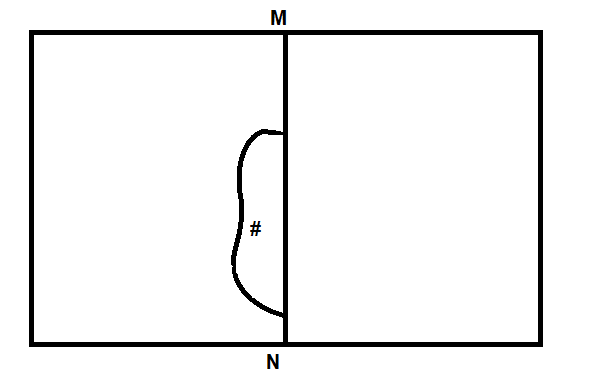
We can look at the angles produced by the tangents to $\#$:
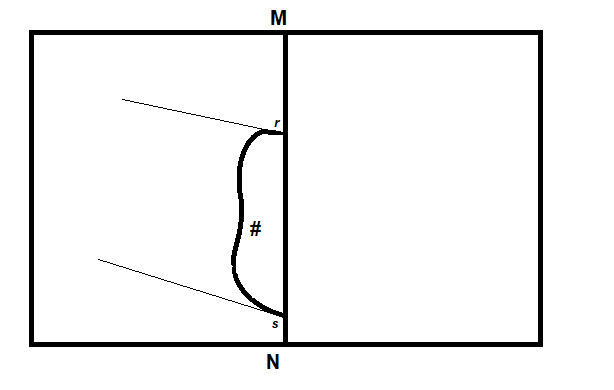
If $r+s\ge180$ then our argument from earlier shows that the left-hand rectangle can be pulled away in a certain direction. Therefore we must have $r+s<180$.
Now, let us rearrange our pieces. Since $\#$ was the only piece cut out of the left-hand rectangle, it is obvious that $P$ and $O$ (one of which may or may notmust be identical with $\#$) must fit perfectly into the space formed when $\#$ is removed. Let us ignore what happens underneath the grey square:
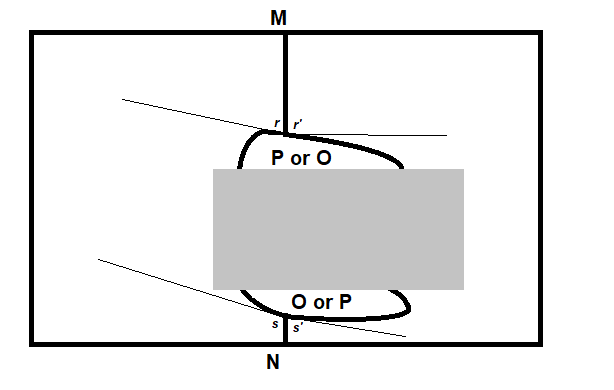
Now by convexity, we can show (as we showed earlier) that $r+r'\ge180$ and $s+s'\ge180$, which implies (since $r+s<180$) that $r'+s'\ge180$.
However, we could have made exactly the same argument with the piece $#'$$\#'$ to show that $r'+s'<180$:
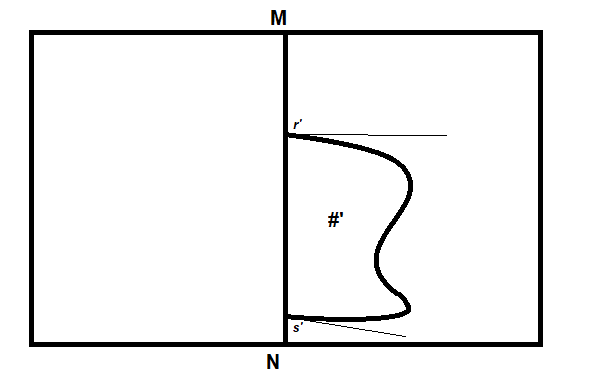
This is a contradiction. Thus it is impossible with two pieces.