Rules
As in Blokus, you have a total of 21 pieces (every piece from monomino to pentomino) in hand:
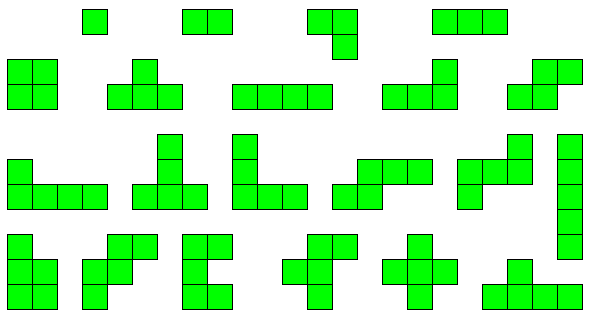
All of these polyominoes are free, this means that you can rotate or flip them as you wish before placing them on the board.
The only rule is that placed polyominos mustn't touch each other by the edge; however, touchings by the corner are acceptable.
(Unlike in the original game, it isn't an obligation for every piece to be corner to corner with at least one of the existing pieces)
Goal
Your goal is to fit all the pieces into a K-row rectangular board (where K is fixed) while trying to minimize the columns used.
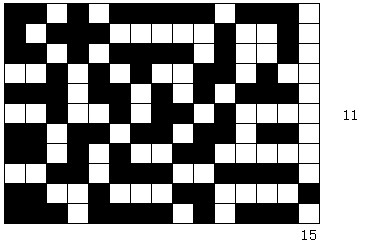
How many columns is optimal for K=11? Please give your answer and proof.
An example of 11x15:
For those who may be interested in other values of K, my current bests are as follows:
K=3 48
4 38
5 30
6 26
7 22
8 20
9 18
10 16
11 15
12 13