It is relatively easy to show that
overlaying square grids at odd angles, disjoint grids, non-uniform spacing of lines and non-rectangular grids are all inefficiencies, giving fewer squares for a given number of straight lines than a single complete rectangular grid.
Now
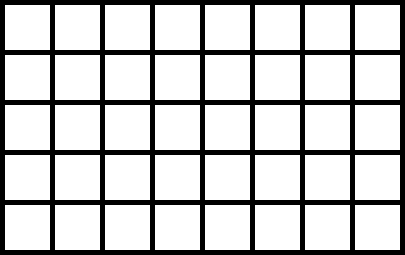
for an $m×n$ grid ($m\ge n$) using $m+n+2$ lines, the number of squares formed is $\frac n6(n+1)(3m-n+1)$ (see OEIS A115262). In particular, $f(8,5)=100$$s(8,5)=100$ and no $m,n$ with $m+n<8+5=13$ gives $f(m,n)=100$$s(m,n)=100$, so the minimum number of lines to make exactly $100$ squares is $15$:
To find the number of different ways to make a particular number $N$ of squares
while sticking to the rectangular grid, simply check whether $N=P_n+kT_n$ for some non-negative $k$, for $n=1,2,3,\dots$ until $P_n>N$, and pool all possibilities. Here $P_n$ is the $n$th square pyramidal number and $T_n$ the $n$rhth triangular number. There are two other ways to make $100$ squares up to symmetry, the $11×4$ and $100×1$ grids.