UPDATE: I've added a large $N$ solution for multiples of 3 that slightly betters OP's solution at $3\times (\frac N 3 - 1)^2 + 9$, see end of this post.
Just to show that @humn is not the only one capable of wasting eyewatering amounts of pizza here are
15
tiny but equal pieces of pizza made using 6 cuts.
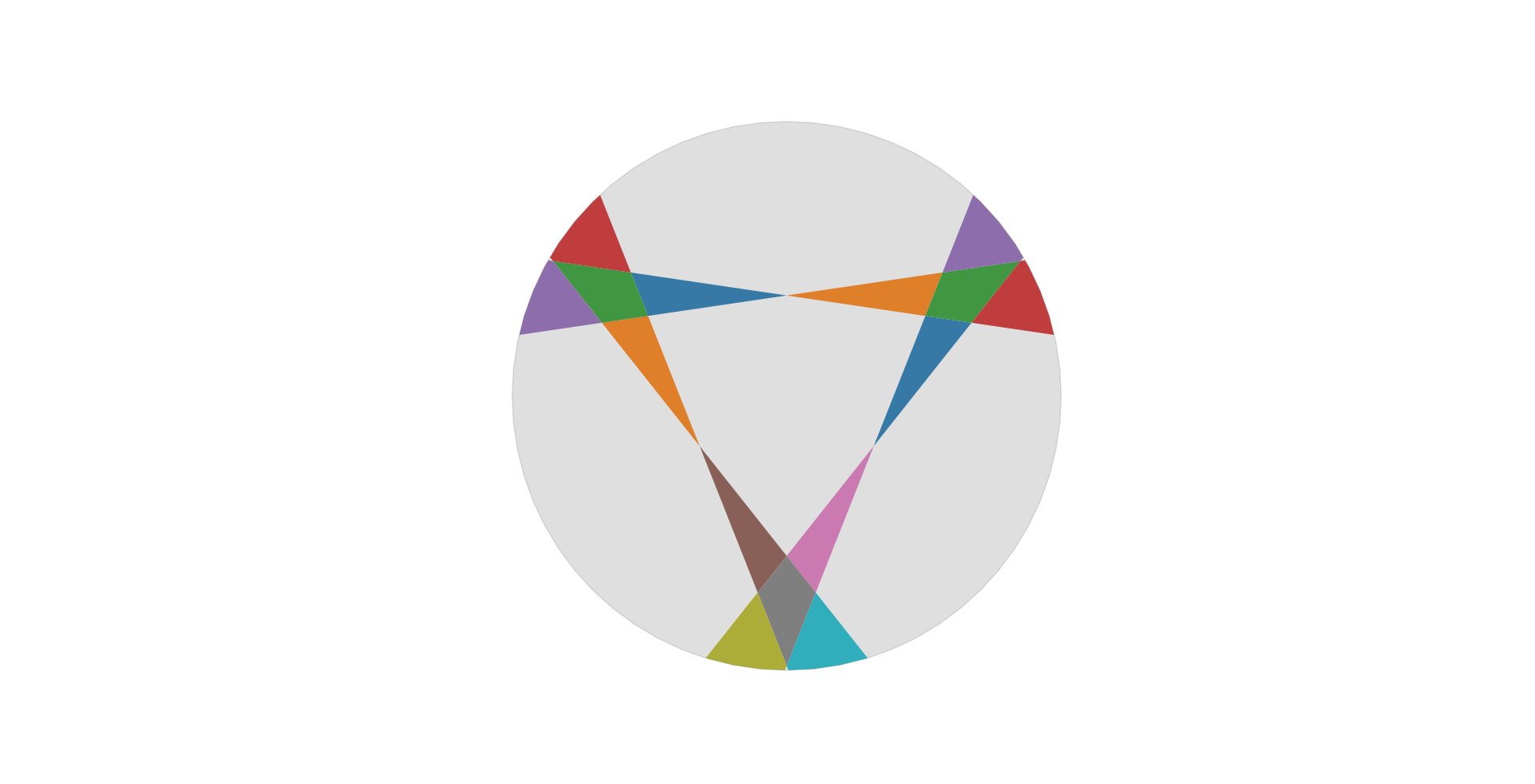
Due to symmetries there are only tree kinds of pieces; equalizing those costs 2 degrees of freedom which we can afford: Let $P$ be the point in the upper center where the blue and orange triangles meet. Then we can adjust the distance of P to the center and the angle between the lines meeting at $P$.
$N = 3n$ solution:
Example $N=12$, 36 slices:
