I've found this problem in my book "Riddles and reason" and after several attempts I still have no idea how to tackle it.

The problem is as follows:

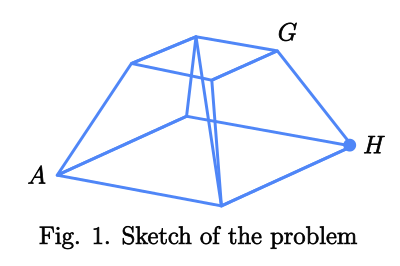
The figure from below shows a truncated pyramid. How different ways can you go from from point $A$ to point $G$ without going through by the same vertex more than once by traveling only the segments shown and without going through $H$?.

The alternatives given are:

$\begin{array}{ll} 1.&\textrm{11}\\ 2.&\textrm{9}\\ 3.&\textrm{12}\\ 4.&\textrm{10}\\ \end{array}$

Does it exist a way to solve this using a graphic or something? (perhaps this might be the best method for understanding this), is the right approach assigning numbers to each vertex?. There isn't any hint given. What sort of logic should be used here?.

I am not very familiar with combinatorics so if it uses them, perhaps the method which best fit me is one which use multiplication which I think maybe is the way to approach this, but I don't know how. But if combinatorics does make it less complicated it could accompany the answer so I could compare the methods. Can someone help me with this?.

I found this problem in my book "Riddles and Reason" and after several attempts I still have no idea how to tackle it.

The problem is as follows:

The figure from below shows a truncated pyramid. How different ways can you go from from point 𝐴 to point 𝐺 without going through by the same vertex more than once by traveling only the segments shown and without going through 𝐻?

The choices given are:

1. 11
2. 9
3. 12
4. 10

Does a way to solve this with a graphic exist? Is assigning numbers to each vertex the right approach? There isn't any hint given. What sort of logic should be used here?

I am not very familiar with combinatorics. The method that should work best for me uses multiplication, but I can't figure out how to do so. If combinatorics simplifies this problem, include it alongside another solution so I can compare methods.