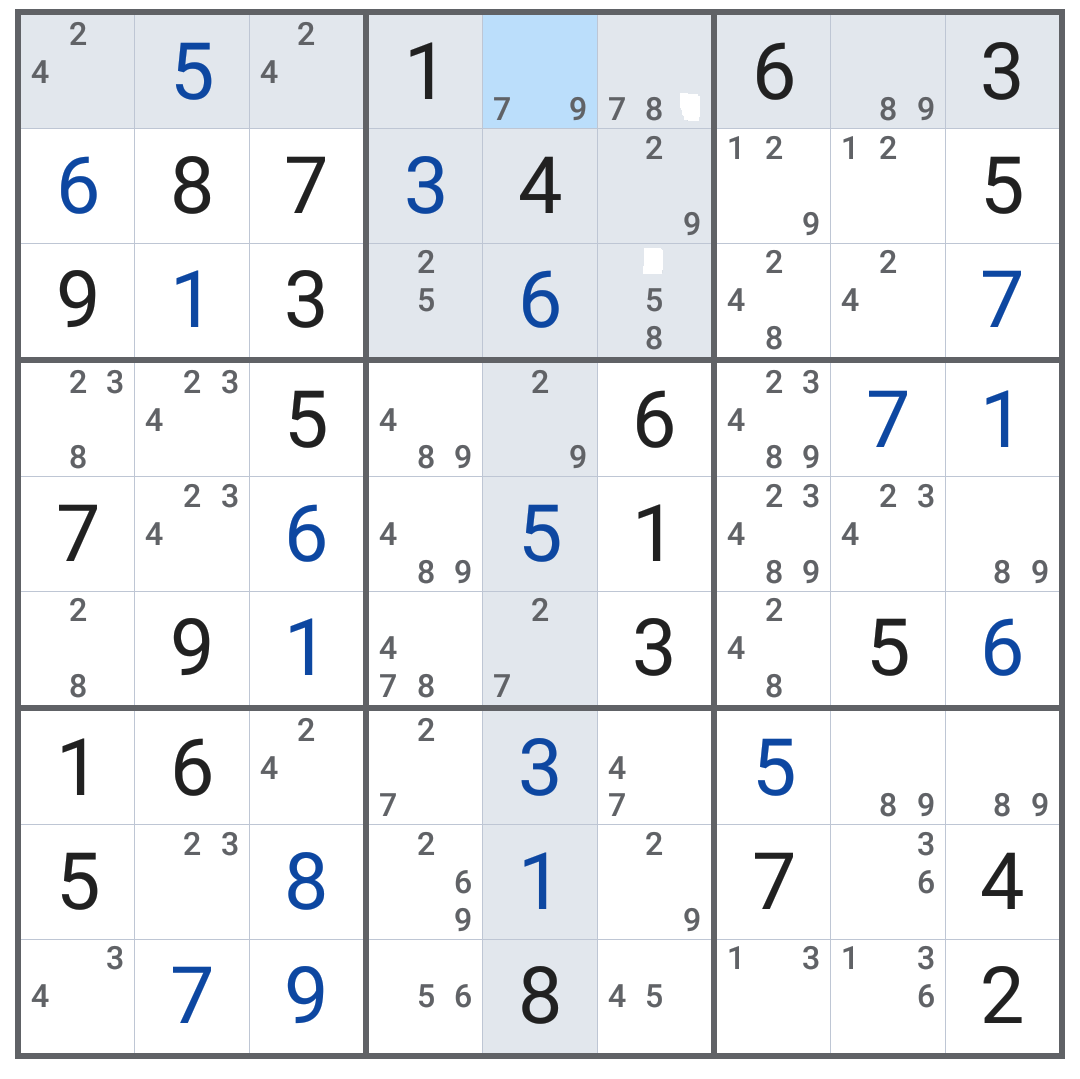
We can solve this puzzle with threetwo sudoko techniques.
First with Naked Pair (already @Glorfindel discussed). This one will fit with column $6^{th}$, where $2$ and $9$ will follow the naked pair, and remaining $2$ and $9$ from column $6^{th}$ would be eliminated.
second with Naked Triple, where $2$, $3$ and $4$ fit for the naked triple of row $5$. Then we will eliminate $4$ from $R5C4$ and $8$ and $9$ from $R5C7$.
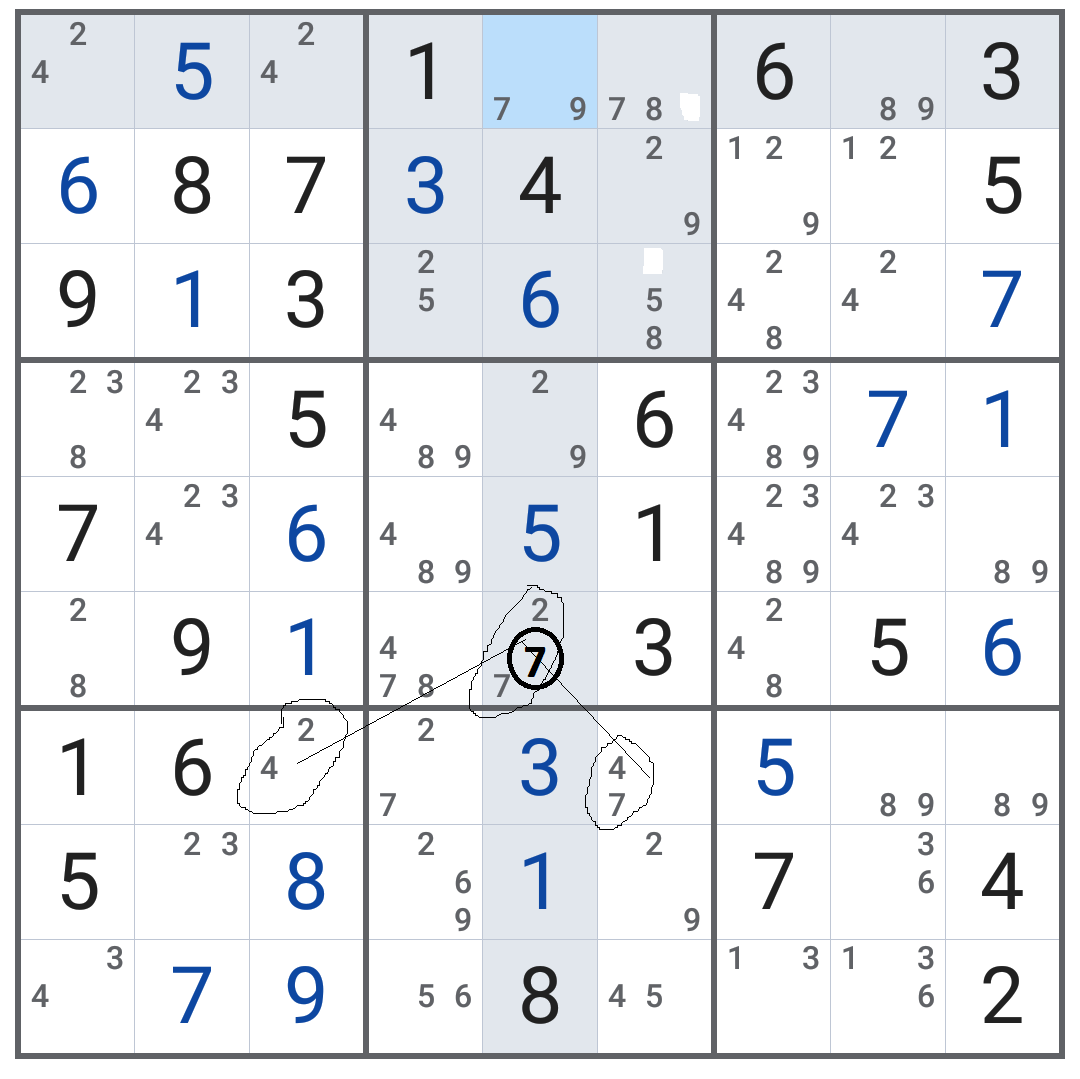
third with XY Wing-Wing : $R6C1$, $R5C4$where R6C5, R7C3 and $R8C6$R7C6 fit for the XY Wing-wing technique. Here we need to assume $8$ or $9$ ofWe will choose $R5C4$$7$ from R6C5, where we assumed $8$ and this assumptionnumber will fit properly (if we assumeother number $9$, it$2$ from R6C5 will not fit properlyhere). Please see the below in the picture.
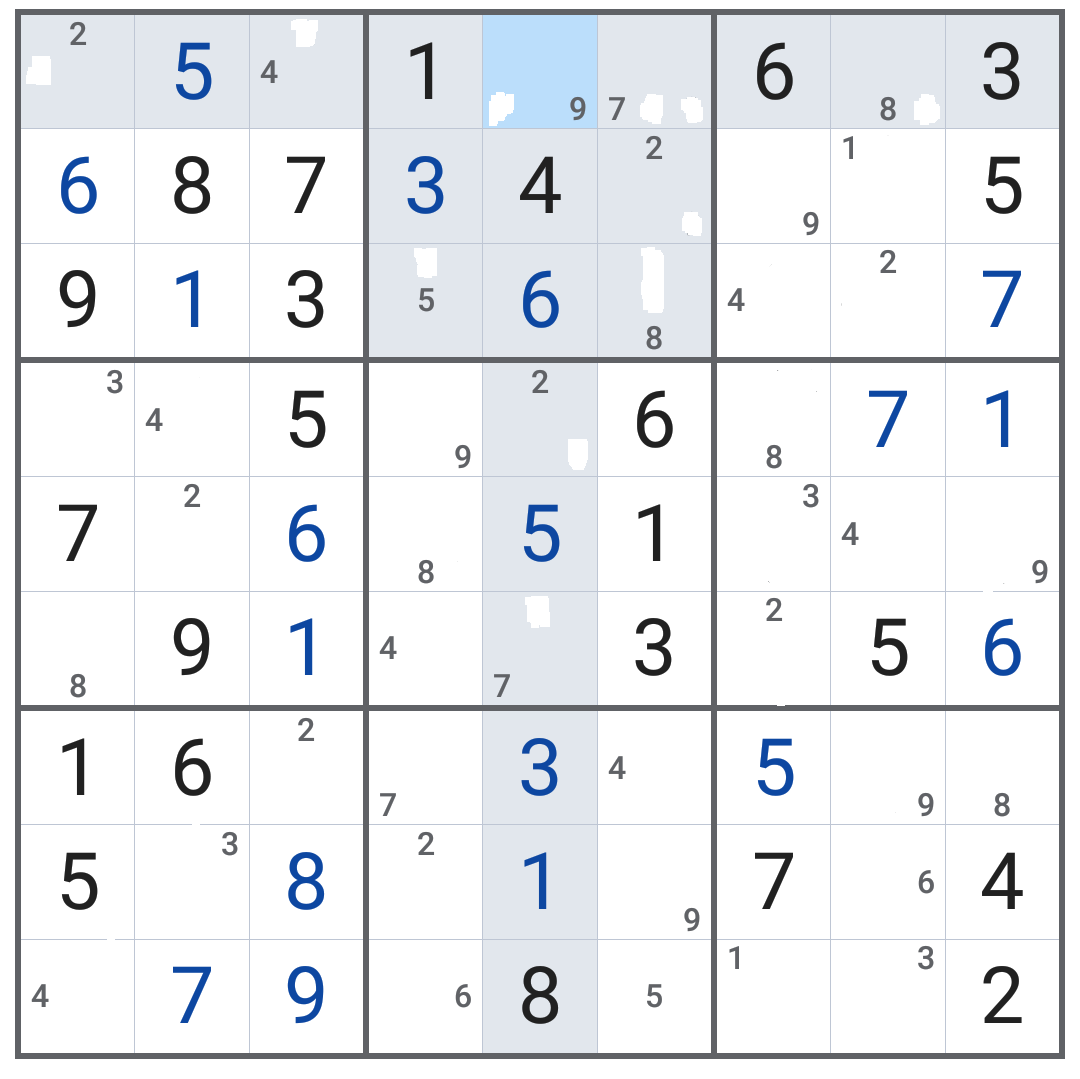
After assuming $8$ of $R5C4$, then the solution would be simple. Final solution looks like.