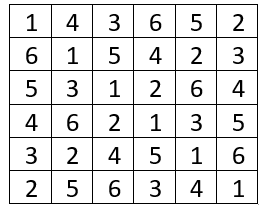
The following Latin Square has an interesting property: there are 6*5=30 possible “ordered dominos” containing distinct digits, each occurring exactly once horizontally and exactly once vertically. For instance 36 (63) occurs horizontally in the top (bottom) row and no other. Also 36 (63) appears vertically in column Two (Five) and no other etc. Let us say that such a Latin Square is perfect. I can’t find any perfect Latin Squares of odd order except for the trivial case of 1x1. Similarly, I can’t find any perfect non-symmetrical Latin Squares of even order. Can you find an example of either of the above, or conversely prove that none exists? Special thanks to my friend @Dmitri Kamenetsky@DmitryKamenetsky for a similar problemproblem about painting a rectangle with K different colours.
I don’t know the answer to this puzzle, so this is a chance to prove you are one of the Awesome People 😊
Text version of image:
1 4 3 6 5 2
6 1 5 4 2 3
5 3 1 2 6 4
4 6 2 1 3 5
3 2 4 5 1 6
2 5 6 3 4 1