A chess board and a coin! [closed]

Question

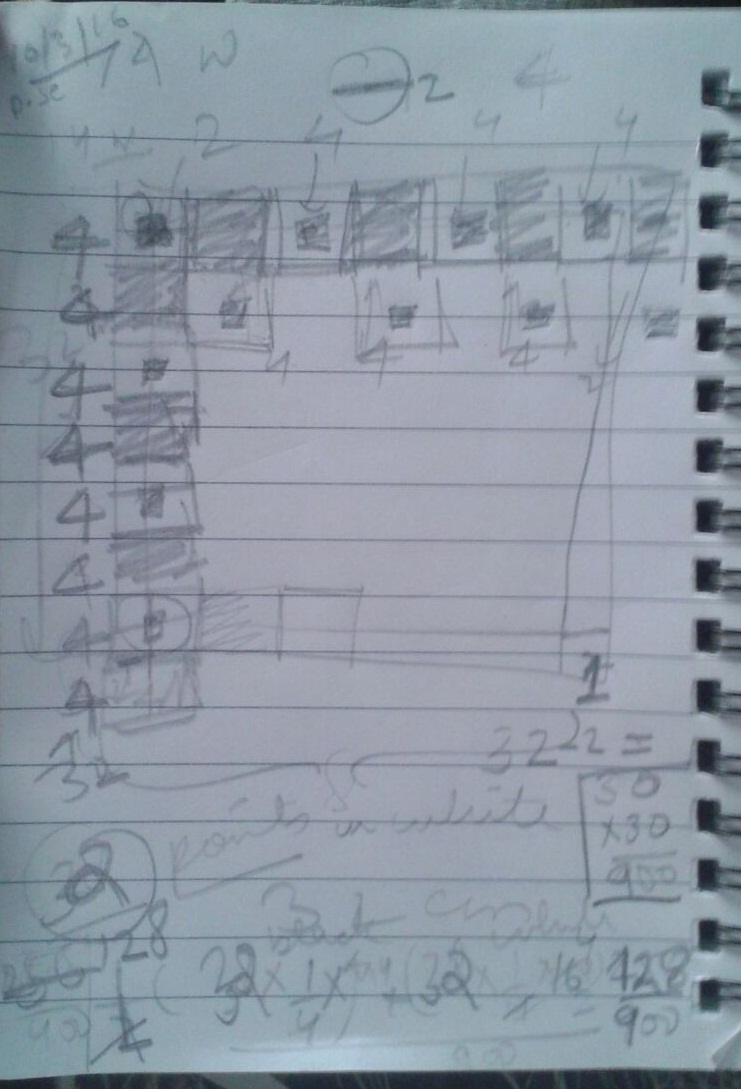
You have a standard chessboard, consisting of $64$ squares ($32$ white and $32$ black) each of dimensions $4\times4$ cm, and you have a standard coin of $2$ cm diameter with tail and head.

You flip the coin and let it drop on the board, and you somehow manage to guarantee that no part of the coin will stay out of the board. (This means that the coin cannot go off the board or stay in any corner of the board with one half on the board and its other half outside the board.)

What are the chances that:

• if you get tail, the coin would be inside a random white square as no part of the coin touches a black square (as defined before),
• if you get head, the coin would be inside a random black square as no part of the coin touches a white square?

Answer 1

The answer is

$128~/~30^2 ~~\approx~~ 0.14222$

---

Explanation:

1. The side length of the chessboard is $4\times8=32$.

2. Let us consider the possibilities, if no part of the coin stays out of the board. Then the center of the coin must land at least $1$cm away from the boundary, and hence must fall into a $30\times30$ square with area $30^2$.

3. Let us consider the possibilities where the coin lands inside one of the white squares. Then the center of the coin must be at least 1cm away from the boundary of such a white square, and hence fall into a small $2\times2$ square right in the middle of that white square. As there are $32$ white squares, the total area of these small $2\times2$ squares is $4\times32=128$.

4. We conclude that the probability that the coin lands inside one of the white squares is $p=128/30^2$. A symmetric argument shows that the probability that the coin lands inside one of the black squares is also $p$.

5. Finally the probability that the coin lands heads is $1/2$, and the probability that the coin lands tails is $1/2$. Hence the probability that the coin lands (heads AND in black square) OR (tails AND in white square) amounts to $(1/2)p+(1/2)p=p$. Done.

Answer 2

I'm not 100% percent sure but I think it's the following

Being heads or tails is independent of landing on a single square. So my guess is we only have to determine what the chance of landing on a single square is and divide it by two.

If it were an $\infty\times\infty$ board this would be a lot easier. The chance of landing only on a single square is when the center of the coin is on the $2\times2$ cm square area in the center of the square. This is $1/4$ of the area of the square so the final chance is $0.25\times0.5 = 0.125$.

But the border makes it a somewhat harder. Basically there is a 1cm width area along the border of the board where the center of the coin can't be. so the total area where the coin can fall is $(4\times 8-2)\times(4\times8-2) = 900$ cm${}^2$. And there are $64 \times 4$ cm${}^2 = 256$ cm${}^2$ where the coin is allowed to fall so I think the final answer is $256/900\times0.5 = 0.14222$.

Answer 3

I think the answer should be :

$\frac{128}{900} $

As given, the coin cannot fall out of the board, nor can it hang. So the total area which will count as the sample space is $30*30=900$.
And, inside each white(or black) square, the favourable area is one-fourth of $ 4*4$ i.e. $4 \space cm^2$

Also, there are 32 such favourable small squares for each black and white.

Thus, the probability: $\frac{total \space favourable \space area}{total\space possible\space area}$
Using independence of the two events (head/tails is independent of falling at random place on chessboard)

$\frac{1}{2} * \frac{32*4+32*4}{900}$

which, on simplifying gives.

$\frac{128}{900} $ or $0.14\overline{2} $

Answer 4

If we know the probability $p$ that the coin will fall inside a single square, then the answer to this question will be $\frac12p$, since given this event there is still a $50\%$ chance that the colour condition will fail (this is so whether the coin is fair on not!). To know whether the coin is inside a single square, it suffices to know the coordinates of its centre: if both the $x$ and $y$ coordinates lie at least at distance $1$ from the coordinate of dividing line between squares, that is of a number divisible by $4$, then we are OK, and otherwise we are not.

Since the coin falls entirely inside the board, the centre has both coordinates in the range $[1,31]$. The "good" coordinates are in one of the ranges $[1,3],[5,7],\ldots,[29,31]$, which accumulate a length Of $8\times2=16$. The the probability for one coordinate to be good is $16/30=8/15$. Then $p=(8/15)^2$, and the outcome of the puzzle is $\frac12p=\frac{32}{225}\approx0.14222$

Answer 5

should be around

7.11%

let me try to explain

Let's say we go for the tail on pure white landing.

We have 50% chance to get tail.

Another 50% to land on a white square

Then we should figure out the chance of the coin landing not on the near square. Here we have 3 sub-set of possibility:

1) "center" which is the 6x6 grid on the inside;

2) the 4 "corner"

3) and the remaining "side".

This happen because you said that the coin can't go outside the board, making the landing in corners and sides easyer for our purpose.For calculation purpose i say that we see where the center of the coin rest after the toss.

on a "Center" square we are surrounded by black square, this means that if our coin rest anywhere near 1cm from the border it will be touching the next square. Said that we can say that 4cm² of the 16cm² are good for us.

As I said on "sides" is easyer. we are surrounded only by 3 black square and the center of the coin can't rest too close the external border of the board cutting down the possible space to 4cm² over 12cm² max.

similar to the "sides", the "corner" squares have 4cm² over 9cm² making this the easyest toss.

putting all of this toghether we have: 256 cm² over a total of 900cm² of good surface where to land our coin, half of it is white and half of that is the time we get tail on our coin. do your math and 7.11%

i' m really sorry if i messed up something (more probably a lot) with my english but it isn't my first lenguage :/