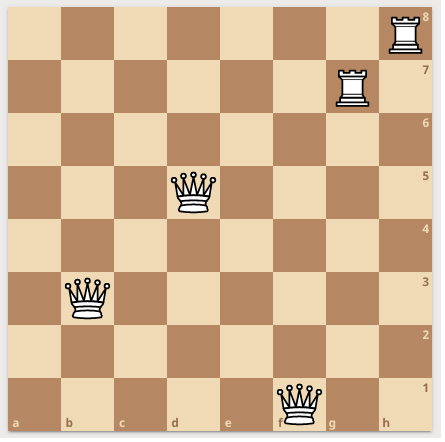
Can you place 3 queens and 2 rooks on a 8x8 chess board, such that every empty cell is under attack? Good luck!
$\begingroup$
$\endgroup$
asked Feb 8, 2021 at 10:17
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
7
I think this will work as a solution
-
1$\begingroup$ @DmitryKamenetsky The main thing I was aiming for here was using the two rooks to exhaust two rows and columns and then place the three queens on a 6x6. Within that framework there are lots of different ways to manoeuvre the two rooks to achieve the same result and then we can rotate/flip the formation of the queens within the 6x6. Do those count as different solutions? $\endgroup$– hexominoCommented Feb 8, 2021 at 11:21
-
1$\begingroup$ @DmitryKamenetsky Then, no, I can't. I think the 6x6 domination is unique up to rotations reflections and setting up the rooks so as not to create the 6x6 space seems like it would be detrimental to placing the queens. I imagine there isn't another way to do it outside what I've suggested in the comment above. $\endgroup$– hexominoCommented Feb 8, 2021 at 12:09
-
1$\begingroup$ Correct. There is no other way of doing it. You get the tick. $\endgroup$ Commented Feb 8, 2021 at 12:26
-
1$\begingroup$ @DmitryKamenetsky Sure there isn´t any other way? $\endgroup$ Commented Feb 8, 2021 at 18:21
-
1$\begingroup$ I see basically 4 ways of setting up the rooks, not counting rotations/reflections. There's your method (x4), swapping to g8,h7 (x4), opposite corners (x2), and opposite sides and one row off, like a7,h8 (x8). Within each of those, there are 4 rotations for the queens in the 6x6 block, so 18 rook arrangements x 4 queen arrangements gives 72 possible solutions. $\endgroup$ Commented Feb 8, 2021 at 20:45